Three distinct points P(3u2,2u3);Q(3v2,2v3) and R(3w2,2w3) are collinear then
Video Solution
|
Answer
Step by step video & image solution for Three distinct points P(3u^(2),2u^(3));Q(3v^(2),2v^(3)) and R(3w^(2),2w^(3)) are collinear then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
The matrices
P[(u1,v1,w1),(u2,v2,w2),(u3,v3w3)]andQ=19⎡⎢⎣22112−5m−815⎤⎥⎦
are such that PQ=l, an identify matrix. Solving the equation ⎡⎢⎣u1v1w1u2v2w2u3v3w3⎤⎥⎦⎡⎢⎣xyz⎤⎥⎦=⎡⎢⎣115⎤⎥⎦, the value of y comes out to be -3, then the value of m is equal toA27B7C−27D−720u3v6(2)−15u2v10u4v+30u3v3
Which of the following is the reduced form of the expression above?A5uv40u7v4B2v−1u+2uv2C4uv−32u2+2uv2D2uv−3uv2u2+6If y=√u ,u=(3-2v)v and v=x2, then dydx=
A4−3x2√3−2x2B3+4x2√3−2x2C3−4x2√3−2x2D4+3x2√3−2x2
If u=v2=w3=z4 ,then logu(uvwz) is equal to
View SolutionThe square root of the complex number z=u2v2+v2u2+12i(uv+vu)+3116 is (in terms of u,v,w)
View SolutionIn the circuit as shown in figure,
E1=2V,E2=2V,E3=1V,andR=r1=r2=r3Ω.
The potential difference between points A and B will be
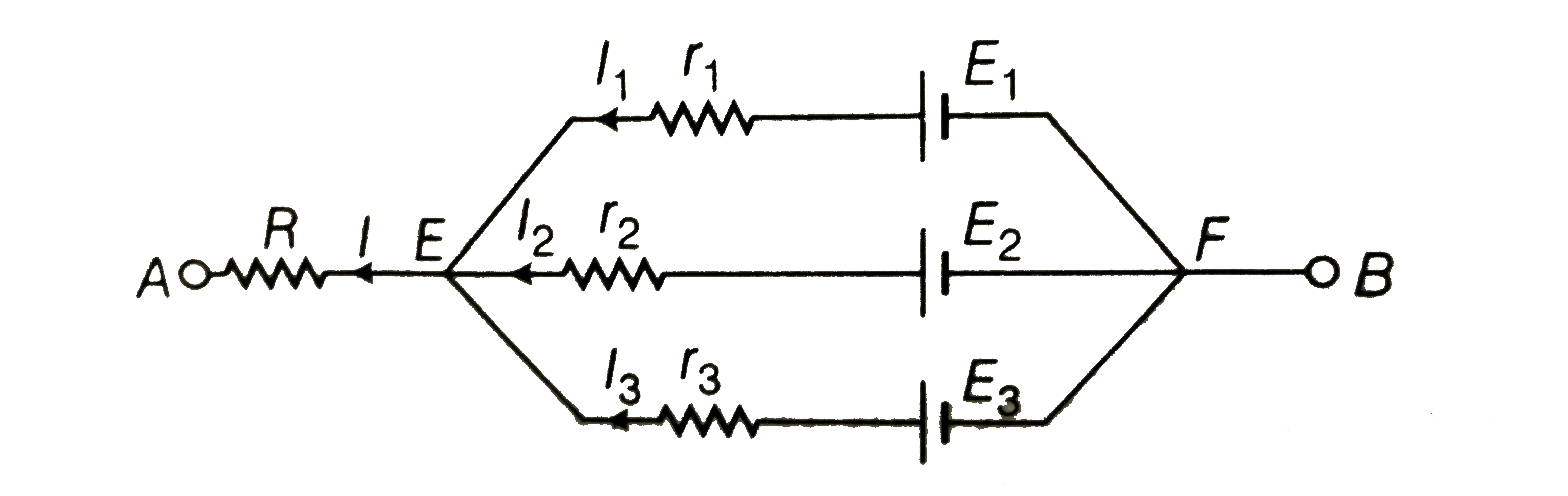
View SolutionFind each of the products: (43u2vw)× (−5uvw2)×(13v2wu)
View SolutionSolve:3(2u+v)=7uv, 3(u+3v)=11uv
View Solution