A0,A1,A2,A3,A4,A5 be a regular hexagon inscribed in a circle of unit radius ,then the product of (A0A1⋅A0A2⋅A0A4 is equal to
Step by step video & image solution for A_0, A_1 ,A_2, A_3, A_4, A_5 be a regular hexagon inscribed in a circle of unit radius ,then the product of (A_0A_1*A_0A_2*A_0A_4 is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
Let A0A1A2A3A4A5 be a regular hexagon inscribed in a circle of unit radius. Then the product of the lengths of the line segments A0A1,A0A2,A0A4 is
A34B3√3C3D3√32Let A0A1A2A3A4A5 be a regular hexagon inscribed in a circle of unit radius. Then the product of the lengths of the line segments A0A1,A0A2andA0A4 is
A34B3√3C3D√32The number of permutations of the letters a1,a2,a3,a4,a5 in which the first letter a1 does not occupy the first position (from the left) and the second letter a2 does not occupy the second position (from the left) is
A96B78C60D42
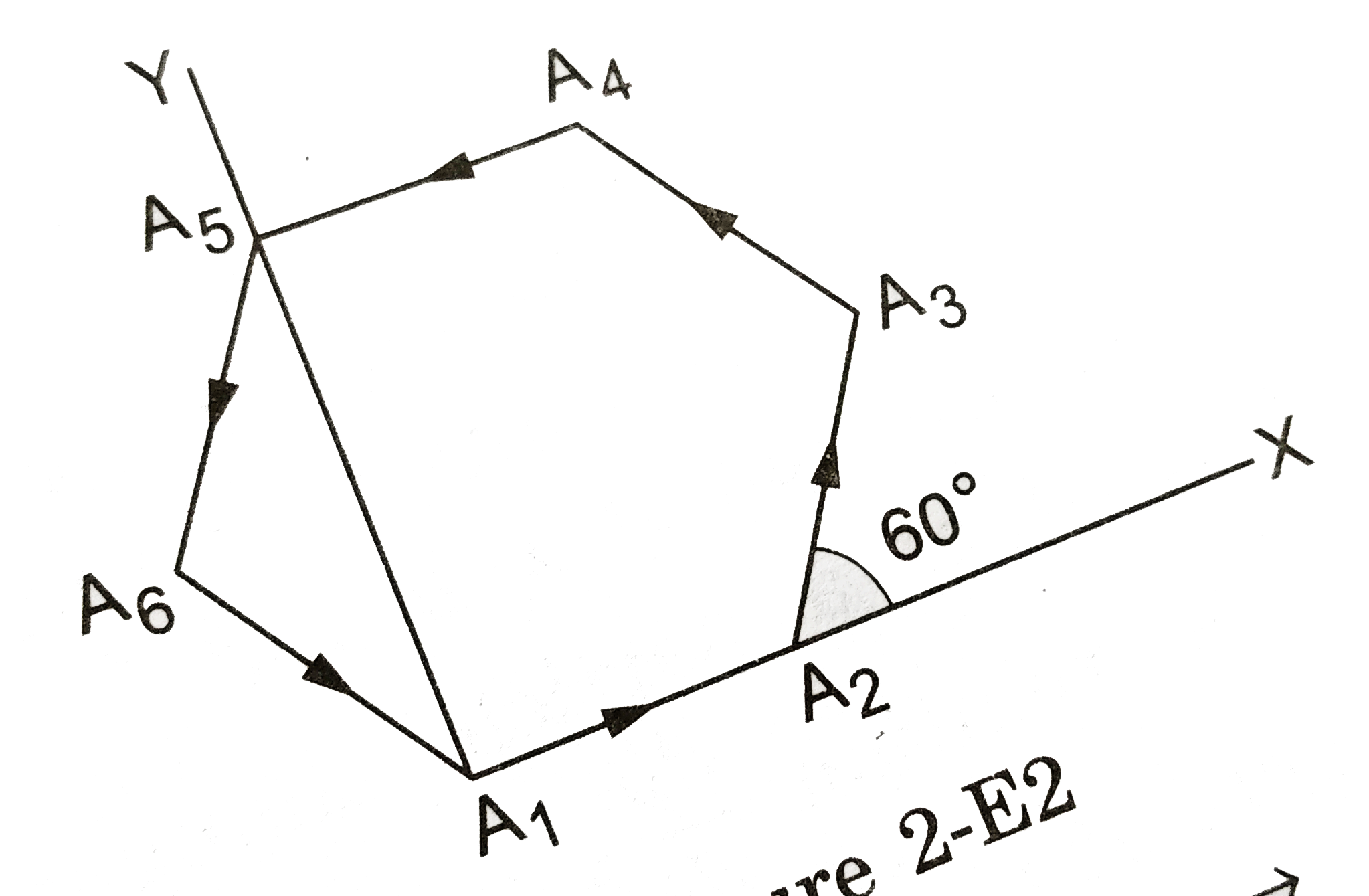
Let A1A2A3A4A5A6A1 be a regular hexagon. Write the x-components of the vectors representeed by the six sides taken in order. Use the fact that the resultant of these six vectors is zero, to prove that cos0+cosπ3+cos(2π)3+cos(4π)3+cos(5π)3=0 Use the known cosine values of verify the result.
 .
.
View SolutionLet 6 Arithmetic means A1,A2,A3,A4,A5,A6 are inserted between two consecutive natural number aandb(a>b). If A21−A22+A23−A24+A25−A26 is equal to prime number then 'b' is equal to
View SolutionLet 6 Arithmetic means A1,A2,A3,A4,A5,A6 are inserted between two consecutive natural number aandb(a>b) .If (A1)2−(A2)2+(A3)2−(A4)2+(A5)2−(A6)2 is equal to prime number then b ' ' is equal to (A)1(B)2(C)3(D)4
View SolutionLet (1+x^2)^2(1+x)^n=A_0+A_1 x+A_2 x^2+…. If A_0, A_1, A_2 ares in A.P. then the value of n is
View SolutionThe average of a1,a2,a3,a4 is 16. Half of the sum of a2,a3,a4 is 23. What is the value of a1
View Solution
