A0,A1,A2,A3,A4,A5 be a regular hexagon inscribed in a circle of unit radius ,then the product of (A0A1⋅A0A2⋅A0A4 is equal to
To solve the problem, we need to find the product of the lengths of the segments A0A1, A0A2, and A0A4 in a regular hexagon inscribed in a circle of unit radius.
1. Understanding the Regular Hexagon:
A regular hexagon has all sides equal and is inscribed in a circle. The radius of the circle is given as 1.
2. Identifying the Vertices:
The vertices of the hexagon can be represented in the Cartesian coordinate system as follows:
- A0=(1,0)
- A1=(12,√32)
- A2=(−12,√32)
- A3=(−1,0)
- A4=(−12,−√32)
- A5=(12,−√32)
3. Calculating the Lengths of the Segments:
- Length A0A1:
A0A1=
⎷(1−12)2+(0−√32)2=
⎷(12)2+(−√32)2=√14+34=√1=1
- Length A0A2:
A0A2=
⎷(1−(−12))2+(0−√32)2=
⎷(1+12)2+(−√32)2=
⎷(32)2+(−√32)2=√94+34=√124=√3
- Length A0A4:
A0A4=
⎷(1−(−12))2+(0−(−√32))2=
⎷(1+12)2+(√32)2=
⎷(32)2+(√32)2=√94+34=√124=√3
4. Calculating the Product:
Now, we can find the product of the lengths:
A0A1⋅A0A2⋅A0A4=1⋅√3⋅√3=1⋅3=3
Final Answer:
The product A0A1⋅A0A2⋅A0A4 is equal to 3.
A regular hexagon has all sides equal and is inscribed in a circle. The radius of the circle is given as 1.
The vertices of the hexagon can be represented in the Cartesian coordinate system as follows:
- A0=(1,0)
- A1=(12,√32)
- A2=(−12,√32)
- A3=(−1,0)
- A4=(−12,−√32)
- A5=(12,−√32)
- Length A0A1:
A0A1=
⎷(1−12)2+(0−√32)2=
⎷(12)2+(−√32)2=√14+34=√1=1
A0A2=
⎷(1−(−12))2+(0−√32)2=
⎷(1+12)2+(−√32)2=
⎷(32)2+(−√32)2=√94+34=√124=√3
A0A4=
⎷(1−(−12))2+(0−(−√32))2=
⎷(1+12)2+(√32)2=
⎷(32)2+(√32)2=√94+34=√124=√3
Now, we can find the product of the lengths:
A0A1⋅A0A2⋅A0A4=1⋅√3⋅√3=1⋅3=3
The product A0A1⋅A0A2⋅A0A4 is equal to 3.
Topper's Solved these Questions
Similar Questions
Let A0A1A2A3A4A5 be a regular hexagon inscribed in a circle of unit radius. Then the product of the lengths the line segments A0A1,A0A2 and A0A4 is
View SolutionLet A0A1A2A3A4A5 be a regular hexagon inscribed in a circle of unit radius. Then the product of the lengths the line segments A0A1,A0A2 and A0A4 is
View SolutionIf the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
View SolutionIf the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
View SolutionIf A1,A2,A3,A4andA5 are the five A.M.'s between 2 nd 8, then find the value of A1+A2+A3+A4+A5
View SolutionLet A1A2A3A4A5A6A1 be a regular hexagon. Write the x-components of the vectors representeed by the six sides taken in order. Use the fact that the resultant of these six vectors is zero, to prove that cos0+cosπ3+cos(2π)3+cos(4π)3+cos(5π)3=0 Use the known cosine values of verify the result.
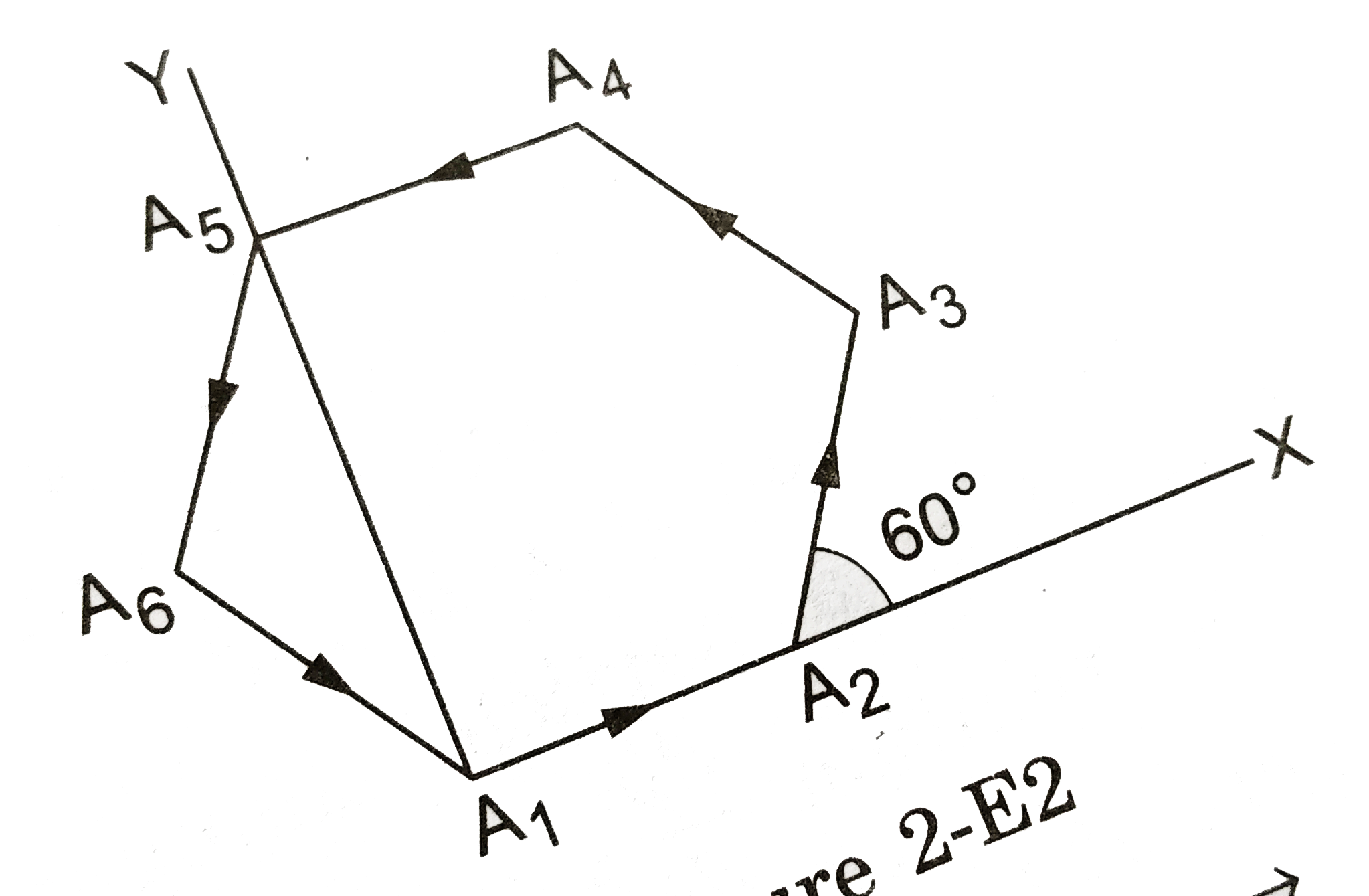 .
.
View SolutionIf A,A1,A2 and A3 are the areas of the inscribed and escribed circles of a triangle, prove that 1√A=1√A1+1√A2+1√A3
View SolutionFive different objects A1,A2,A3,A4,A5 are distributed randomly in 5 places marked 1, 2, 3, 4, 5. One arrangement is picked at random. The probability that in the selected arrangement, none of the object occupies the place corresponding to its number, is
View SolutionIf cos4x=a0+a1cos2x+a2cos4x is true for all values of x∈R, then the value of 5a0+a1+a2 is_______
View SolutionIf cos4x=a0+a1cos2x+a2cos4x is true for all values of x∈R, then the value of 5a0+a1+a2 is_______
View Solution