A rotating table has angular velocity 'ω' and moment of inertia I1. A person of mass 'm' stands on the centre of rotating table. If the preson moves a distance r along its radius then what will be thefinal angualr velcotiy of the rotating table ?
To solve the problem, we will use the principle of conservation of angular momentum. The angular momentum of a system remains constant if no external torques act on it.
1. Define Initial Conditions:
- The initial angular velocity of the rotating table is given as ω1.
- The moment of inertia of the rotating table is I1.
- The person of mass m is initially at the center of the table (distance r=0).
2. Calculate Initial Angular Momentum:
- The initial angular momentum L1 of the system (table + person) can be calculated as:
L1=I1⋅ω1
- Since the person is at the center, their contribution to the moment of inertia is zero at this point.
3. Define Final Conditions:
- When the person moves a distance r along the radius, their new position contributes to the moment of inertia.
- The moment of inertia of the person when they are at a distance r from the center is given by:
Iperson=m⋅r2
- The new total moment of inertia I2 of the system becomes:
I2=I1+m⋅r2
4. Calculate Final Angular Momentum:
- The final angular momentum L2 of the system after the person moves is:
L2=I2⋅ω2
- Here, ω2 is the final angular velocity we want to find.
5. Apply Conservation of Angular Momentum:
- According to the conservation of angular momentum:
L1=L2
- Substituting the expressions for L1 and L2:
I1⋅ω1=(I1+m⋅r2)⋅ω2
6. Solve for Final Angular Velocity ω2:
- Rearranging the equation to solve for ω2:
ω2=I1⋅ω1I1+m⋅r2
Final Answer:
The final angular velocity of the rotating table when the person moves a distance r along its radius is given by:
ω2=I1⋅ω1I1+m⋅r2
- The initial angular velocity of the rotating table is given as ω1.
- The moment of inertia of the rotating table is I1.
- The person of mass m is initially at the center of the table (distance r=0).
- The initial angular momentum L1 of the system (table + person) can be calculated as:
L1=I1⋅ω1
- Since the person is at the center, their contribution to the moment of inertia is zero at this point.
- When the person moves a distance r along the radius, their new position contributes to the moment of inertia.
- The moment of inertia of the person when they are at a distance r from the center is given by:
Iperson=m⋅r2
- The new total moment of inertia I2 of the system becomes:
I2=I1+m⋅r2
- The final angular momentum L2 of the system after the person moves is:
L2=I2⋅ω2
- Here, ω2 is the final angular velocity we want to find.
- According to the conservation of angular momentum:
L1=L2
- Substituting the expressions for L1 and L2:
I1⋅ω1=(I1+m⋅r2)⋅ω2
- Rearranging the equation to solve for ω2:
ω2=I1⋅ω1I1+m⋅r2
The final angular velocity of the rotating table when the person moves a distance r along its radius is given by:
ω2=I1⋅ω1I1+m⋅r2
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 1
90 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 2
40 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-4
38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBOOK - MOTIONCHAPTER - SEMI CONDUCTOR AND LOGIC GATESEXERCISE - EXERCISE 3
34 Videos
Similar Questions
A turn table of mass M and radius R is rotating with angular velocity ω0 on frictionless bearing. A spider of mass m falls vertically onto the rim of the turn table and then walks in slowly towards the centre ofthe table. What is the angular velocity of the system when spider is at a distance r from the centre. Compute also the angular velocity of the turn table when the spider is at the rim and at the centre of the table .Is the energy of the system in this problem conserved ?
View SolutionA solid cylinder of mass 'M' and radius 'R' is rotating along its axis with angular velocity ω without friction. A particle of mass 'm' moving with velocity v collides aganist the cylinder and sticks to its rim. After the impact calculate angular velocity of cylinder .
View Solution
Knowledge Check
A rotating table completes one rotation is 10 sec. and its moment of ineratia is 100 kg-m2. A person of 50 kg. mass stands at the centre of the rotating table. If the person moves 2m. from the centre, the angular velocity of the rotating table in rad/sec. will be:
A2π30B20π30C2π3D2πA man stands at the centre of turn table and the turn table is rotating with certain angular velocity. If he walks towards rim of the turn table, then
Amoment of inertia of the system decreasesBangular momentum of system increasesCangular velocity of the system increasesDkinetic energy of the system decreasesA person is standing on a rotating table with metal spheres in his hands. If he withdraws his hands to his chest, then the effect on his angular velocity will be.
AincreaseBdecreaseCremain sameDcan't say
Suppose a person of mass m stands at the edge of a circular platform of radius R and moment of inertia I. The platform is at rest initially, but the platform begins to rotate when the person begins to move with velocity v. Determine the angular velocity of the platform.
View SolutionCalculate the work that has to be done to stop an iron sphere of radius R rotating about its diameter with an angular velocity ω.
View SolutionDemonstrate that in the reference frame rotating with a constant angular velocity ω about a stationary axis a body of mass m experiences the resultant
(a) centrifugal force of inertia Fcf=mω2RC, where RC is the radius vector of the body's centre of inertia relative to the rotation axis,
(b) Coriolis force Fcor=2m[v′Cω], where v′C is the velocity of the body's centre of inertia in the rotating reference frame.
View SolutionA small bead of mass m moving with velocity v gets threaded on a stationary semicircular ring of mass m and radius R kept on a horizontal table. The ring can freely rotate about its centre. The bead comes to rest relative to the ring. What will be the final angular velocity of the system?
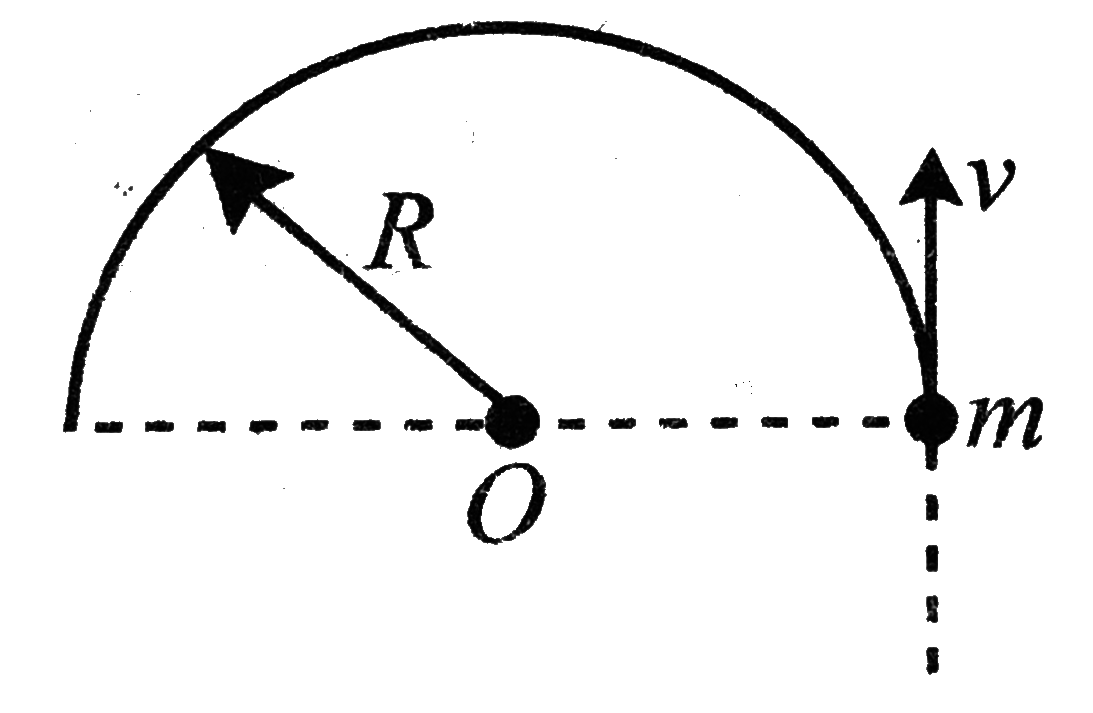
View SolutionA thin circular ring of mass m and radius R is rotating about its axis perpendicular to the plane of the ring with a constant angular velocity ω. Two point particleseach of mass M are attached gently to the opposite end of a diameter of the ring. The ring now rotates, with an angular velocityω2. Then the ratiomM is
View Solution
