Two stars A and B of surface area Sa and Sb & temperature TaandTb glow red and blue respectively. Choose the correct option.
T4>Tb
Ta<Tb
TaSa=TbSb
TaSb=TbSa
The correct Answer is:B
To solve the problem, we will utilize Wien's Displacement Law and Stefan-Boltzmann Law to analyze the relationship between the temperatures and the colors of the stars A and B.
1. Understanding Wien's Displacement Law:
- Wien's Displacement Law states that the wavelength (λm) at which the emission of a black body spectrum is maximized is inversely proportional to its absolute temperature (T). This can be expressed mathematically as:
λm⋅T=b
where b is a constant (approximately 2.898×10−3m K).
2. Identifying the Colors:
- In the question, star A glows red and star B glows blue. We know that red light has a longer wavelength than blue light. Therefore, we can denote:
λA>λB
- Since λ is inversely proportional to T, we can write:
λA⋅TA=bandλB⋅TB=b
3. Relating Temperatures:
- From the above equations, we can express the temperatures in terms of wavelengths:
TA=bλAandTB=bλB
- Since λA>λB, it follows that:
TA<TB
- This means that the temperature of star A (which glows red) is less than the temperature of star B (which glows blue).
4. Analyzing Power Radiated:
- According to Stefan-Boltzmann Law, the power radiated by a black body is given by:
P=σEAT4
where σ is the Stefan-Boltzmann constant, E is the emissivity, and A is the surface area.
- For stars A and B, the power radiated can be expressed as:
PA=σESAT4AandPB=σESBT4B
- Without specific values for SA and SB, we cannot determine which star radiates more power based solely on the information given.
5. Conclusion:
- From the analysis, we conclude that:
- The temperature of star A is less than that of star B.
- We cannot definitively compare the power radiated by the two stars without additional information about their surface areas.
Final Answer:
The correct statement is that the temperature of star A (red) is less than the temperature of star B (blue).
- Wien's Displacement Law states that the wavelength (λm) at which the emission of a black body spectrum is maximized is inversely proportional to its absolute temperature (T). This can be expressed mathematically as:
λm⋅T=b
where b is a constant (approximately 2.898×10−3m K).
- In the question, star A glows red and star B glows blue. We know that red light has a longer wavelength than blue light. Therefore, we can denote:
λA>λB
- Since λ is inversely proportional to T, we can write:
λA⋅TA=bandλB⋅TB=b
- From the above equations, we can express the temperatures in terms of wavelengths:
TA=bλAandTB=bλB
- Since λA>λB, it follows that:
TA<TB
- This means that the temperature of star A (which glows red) is less than the temperature of star B (which glows blue).
- According to Stefan-Boltzmann Law, the power radiated by a black body is given by:
P=σEAT4
where σ is the Stefan-Boltzmann constant, E is the emissivity, and A is the surface area.
- For stars A and B, the power radiated can be expressed as:
PA=σESAT4AandPB=σESBT4B
- Without specific values for SA and SB, we cannot determine which star radiates more power based solely on the information given.
- From the analysis, we conclude that:
- The temperature of star A is less than that of star B.
- We cannot definitively compare the power radiated by the two stars without additional information about their surface areas.
The correct statement is that the temperature of star A (red) is less than the temperature of star B (blue).
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - HEAT TRANSFER & THERMAL EXPANSION EXERCISE - Exercise - 3 Section-B19videos
HEAT TRANSFER & THERMAL EXPANSION
- BOOK - MOTIONCHAPTER - HEAT TRANSFER & THERMAL EXPANSION EXERCISE - EXERCISE - 245videos
HEAT TRANSFER & THERMAL EXPANSION
- BOOK - MOTIONCHAPTER - HEAT - 1EXERCISE - EXERCISE -4 (Level - II) Previous Year | JEE Advanced22videos
HEAT - 1
- BOOK - MOTIONCHAPTER - HEAT-2 EXERCISE - EXERCISE-4 (LEVEL-II)30videos
HEAT-2
Similar Questions
Knowledge Check
- Question 1 - Select One
Choose the correct option w.r.t TMV
ACapsomeres are arranged in polyhedral formBPossess non-infectious RNACBeijerinck found that they can pass through bacteria-proof filtersDCrystals consist largely of proteins - Question 2 - Select One
Choose the correct option w.r.t RNA.
APresence of thymine in place of unracilBAbsence of free 2'OH in sugarCMutates at faster rateDIs non-catalytic - Question 3 - Select One
If a>b,then choose the correct option
Aa+3≥b+3B3a>3bCa3<b3Da2<b2
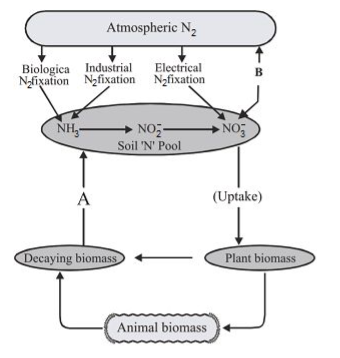
Choose the correct option for A and B :-View SolutionIn the reported figure, there is a cyclic process ABCDA on a sample of 1 mol of a diatomic gas. The temperature of the gas during the process A→BandC→D are T1andT2(T1>T2) respectively.
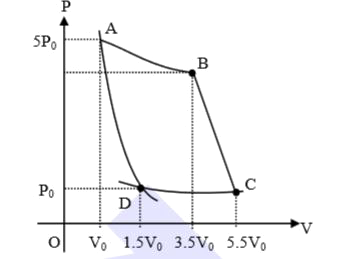
Choose the correct option out of the following for work done if processes BC and DA are adiabaticView SolutionChoose correct option w.r.t. Lenticels
View SolutionLet TaandTb be the final temperatures of the samples A and B respectively in the previous question.
View SolutionOn the P−T graph of an ideal gas, choose the correct option(s):
View Solution