The line segments joining the midpoints M and N of parallel sides AB and DC respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD=BC
Step by step video & image solution for The line segments joining the midpoints M and N of parallel sides AB and DC respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD=BC by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
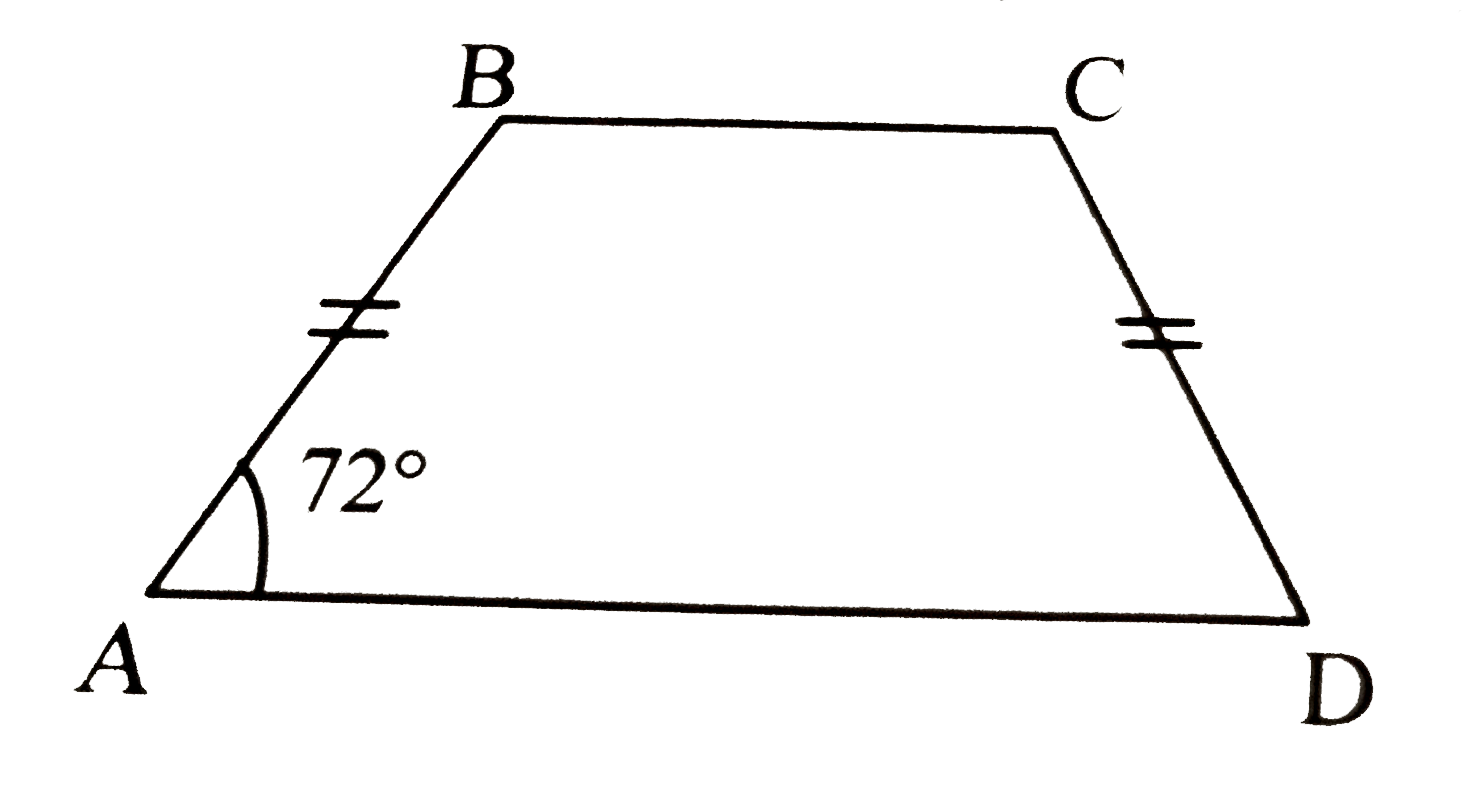
In trapezium ABCD, side BC ∣∣ side AD, side AB ≅ side DC. If ∠A=72∘ , then find the measures of ∠B and ∠D .
View SolutionThe line segments joining the midpoints M and N of parallel sides AB and DC respectively of a trapezium ABCD is perpendicular to both the sides AB and DC. Prove that AD=BC.
View SolutionThe line segment joining the points M and N between the parallel sides AB and DC respectively of a trapezoid ABCD is perpendicular to both sides AB and DC. Prove that AD = BC.
View SolutionIn trapezium ABCD, side BC ∣∣ side AD, side AB ≅ side DC.
If ∠A=72∘ , then find the measures of ∠B and ∠D .

View Solutionआकृति में, ABCD एक समलम्ब चतुर्भुज हैं, जिसमें भुजा AB भुजा DC के समान्तर हैं तथा E भुजा AD का मध्य-बिंदु हैं। E भुजा BC पर इस प्रकार है खंड EF, भुजा DC के समान्तर हैं। सिद्ध कीजिए कि EF=12(AB+DC)
View SolutionIn trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is mid-point of BC.
Prove that : AB + DC = 2EF.
View SolutionIn quadrilateral ABCD ,side DC is largest show that AB + AD > DC - BC
View SolutionIn quadrilateral ABCD, AB=DC and AD=BC. Prove that the sides AB and DC are parallel to each other.
View Solution
