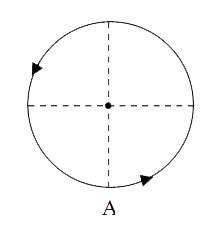
A particle is describing uniform circular motion in the anti-clockwise sense such that its time period of revolution is T. At t = 0 the particle is observed to be at A. If θ1 be the angle
between acceleration vector at t=T4 and average velocity vector in the time interval 0 to T/4 and θ2 be the angle between acceleration vector at t=T4 and the vector representing change in velocity in the time interval 0 to T/4, then :
θ1=135∘,θ2=45∘
θ1=135∘,θ2=135∘
θ1=45∘,θ2=135∘
θ1=45∘,θ2=45∘
The correct Answer is:A
Let initial point A is on –y-axis and at t =T/4, it is at + x-axis.
At t=T/4,...…(i)
Att=0,−V=Vˆi,att=T/4,−V=Vˆj
△−V=Vˆj−Vˆi…(ii)
Average velocity from t=0 to t=T/4 is equal to Rˆi−(−Rˆj)T/4=4RT=(ˆi+ˆj)…(iii)
⇒θ1 = angle between vectors −ˆiandˆi+ˆj=135∘andθ2 =angle between vector −ˆiandˆj−ˆi=45∘
Similar Questions
Knowledge Check
- Question 1 - Select One
A particle starts moving at t = 0 in a circle of radius R=2m with constant angular acceleration of a−3rad/sec2. Initial angular speed of the particle is 1 rad/sec. At time t0 the angle between the acceleration vector and the velocity vector of the particle is 37∘.
What is the magnitude of the acceleration of the particle at t0 ?A6m/s2B4.5m/s2C7.5m/s2D5m/s2 - Question 2 - Select One
For the acceleration - time (a - t) graph shown in figure, the change in velocity of particle from t = 0 to t = 6 s is
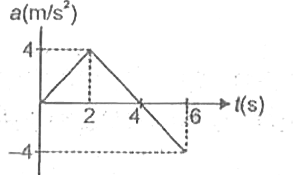 A10 m/sB4 m/sC12 m/sD8 m/s
A10 m/sB4 m/sC12 m/sD8 m/s - Question 3 - Select One
A particle starts moving at t = 0 in a circle of radius R=2m with constant angular acceleration of a−3rad/sec2. Initial angular speed of the particle is 1 rad/sec. At time t0 the angle between the acceleration vector and the velocity vector of the particle is 37∘.
What is the value of t0 ?A1sB12sC13sD16s
A particle move with a velocity v=αt3 along a straight line.The average velocity in time interval t=0 to t=T is
View SolutionA particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
View SolutionA particle is moving along x-axis. Its X-coordinate varies with time as, X=2t2+4t−6 Here, X is in meters and t in seconds. Find average velocity between the time interval t=0→t=2s.
View SolutionFor body moving with uniform acceleration a, initial and final velocities in a time interval t are u and v respectively. Then its average velocity in the time interval t is
View SolutionVeolocity-time graph of a particle undergoing rectilinear motioin is plotted upto t=t4 as shown in the figure. Average acceleration of the particle is zero in the time internal between
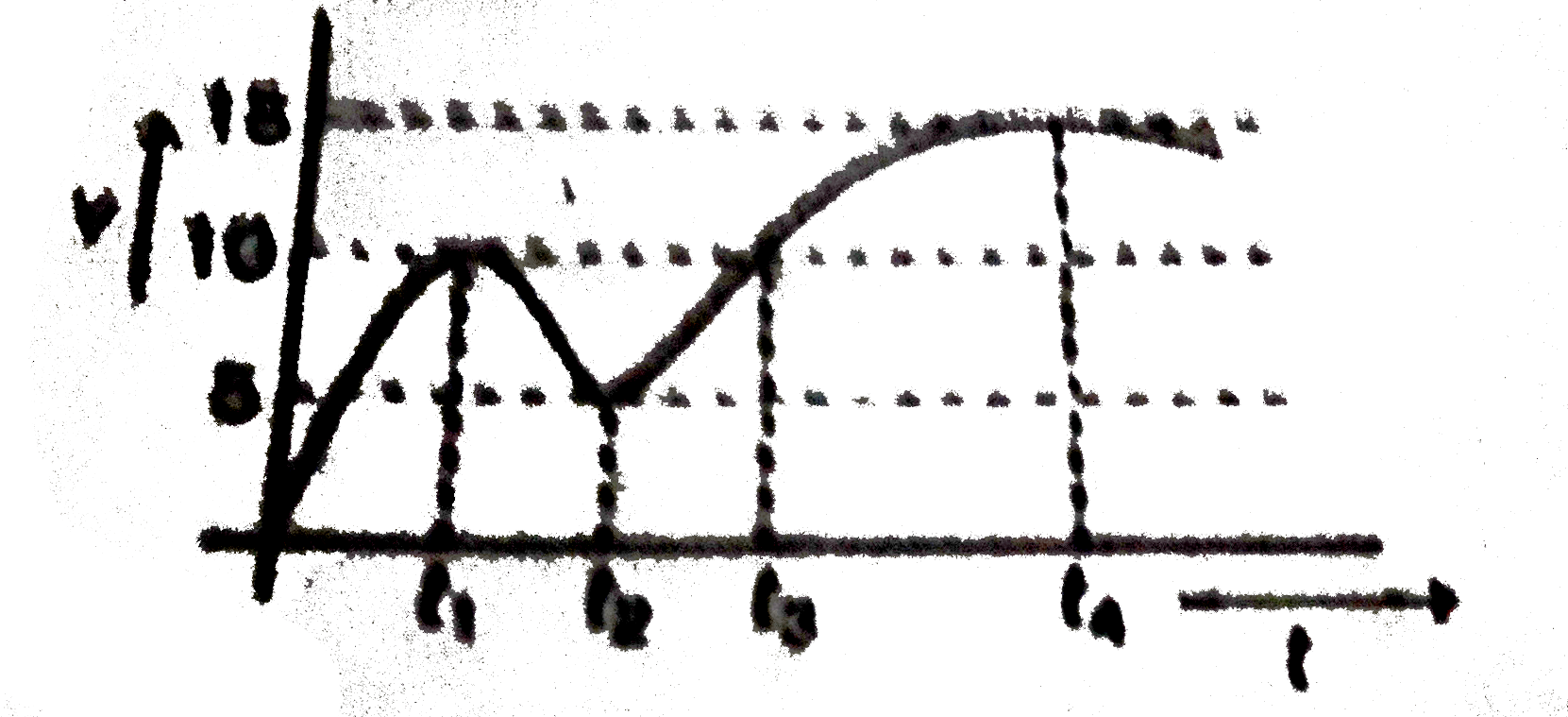 View Solution
View Solution

