If ∫(2x+3)x(x+1)(x+2)(x+3)+1dx=−1px2+qx+r+c then 3p−qr=Solution in Telugu
0
1
2
−1
The correct Answer is:A
Step by step video, text & image solution for If int ((2x+3))/(x(x+1)(x+2)(x+3)+1)dx =-1/(px^(2)+qx+r)+c then (3p-q)/r= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
If f:[0,∞)→[0,∞) is defined by f(x)=x1+x, then f is
03:00View SolutionIf [x] denotes the greatest integer <x then the domain of the function function f(x)=√4−x2[x]+2 is
05:44View Solutionint((2x+3)dx)/(x(x+1)(x+2)(x+3)+1)=C-(1)/(f(x))
02:08View Solution∫pxp+2q−1−qxq−1x2p+2q+2xp+q+1dx
06:56View SolutionIf α and β are the zeros of the polynomial f(x)=x2+px+q , then a polynomial having 1α and 1β is its zeros is (a) x2+qx+p (b) x2−px+q (c) qx2+px+1 (d) px2+qx+1
05:19View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
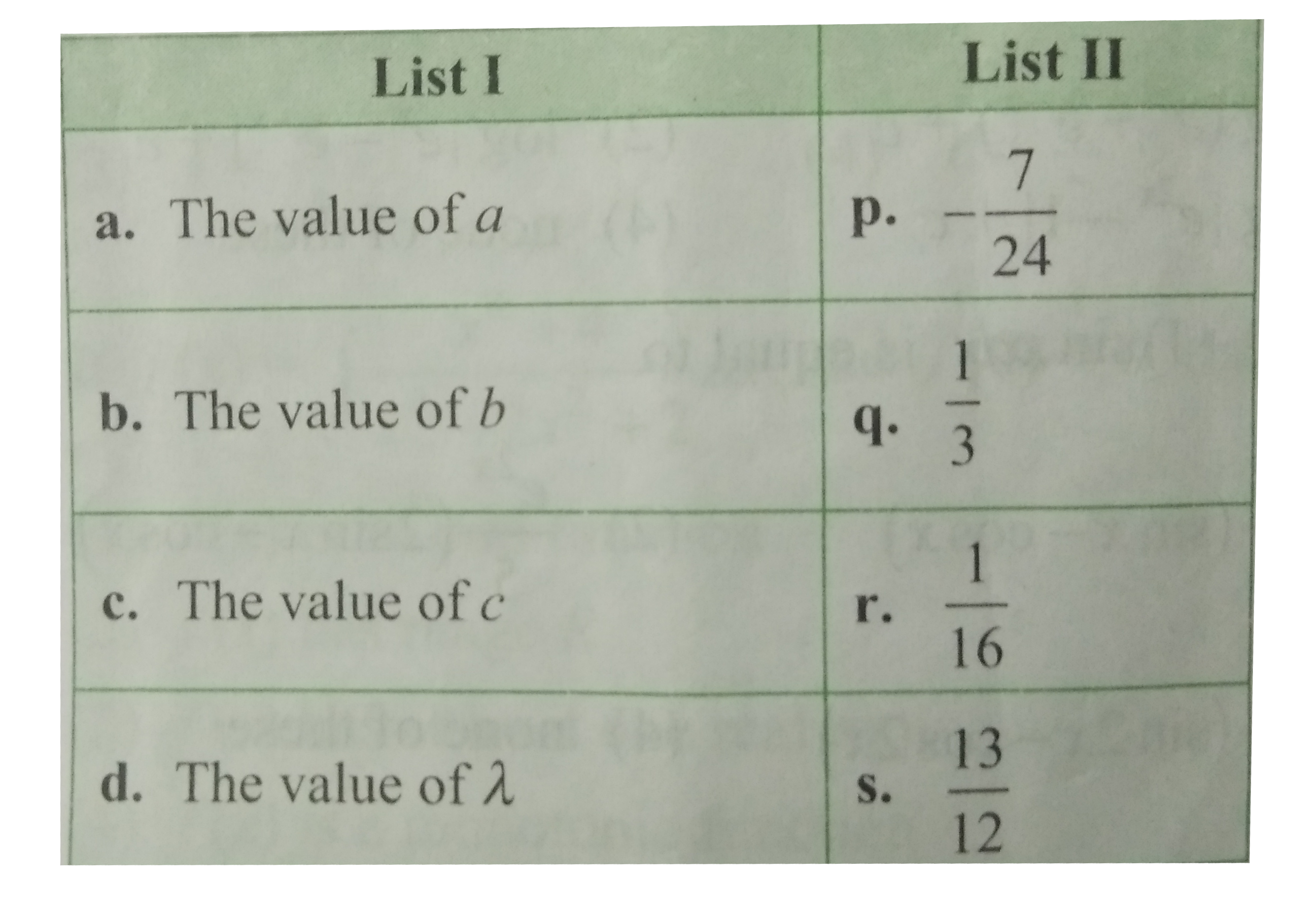
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr02:59View Solutionp एवं q के मान निकाले यदि x−1 तथा x+2 बहुपद 2x3+px2+qx−14 के गुणनखंड हो।
03:39View SolutionIf a,b,c are roots of x3−px2+qx−r=0 and r ≠ 0, then find 1a2+1b2+1c2 in terms of p,q,r.
View SolutionIf f (x) ={px2+qfor x≤1qx2+px+rfor xl>1 where q≠0 is derivable at x =1 ,then
05:53View Solution∫1x+√x2−x+1dx=Plog(x+√x2−x+1)+Qlog(2x−1+2√x2−x+1)+R(32x−1+2√x2−x+1)+c
Then the values of P, Q, R areView Solution∫2x12+5x9(x5+x3+1)3dx=xpq(x5+x3+1)r+c, then p−q−r=
View Solutionif ∫(2x+3)x(x+1)(x+2)(x+3)+1dx=−1px2+qx+r+c , than 3p−qr
03:07View Solution(i) ∫2x(x2+1)(x2+3)dx
(ii) ∫2x(1+x3)(2+x3)dx08:33View Solutionx3+px2−qx+r నకు 3 ఒకే ఒక శూన్యం అయిన -3p+r విలువ
06:49View Solution∫(x3−1)dxx4√6x6−4x3+2(x>0)=√6x6−4x3+PQxR+c then P+Q−R is equal to
03:31View Solution

