Three identical charges, each $2 μ C$ lie at the vertices of a right angled triangle as shown in the figure. Forces on the charges at B due to the charges at A and C respectively are $F_{1} and F_{2}$ . The angle between their resultant force and $F_{2}$ is .

A

${tan}^{- 1} (\frac{9}{16})$

B

${tan}^{- 1} (\frac{9}{7})$

C

${tan}^{- 1} (\frac{16}{9})$

D

${tan}^{- 1} (\frac{7}{9})$ .

Text Solution

Verified by Experts

The correct Answer is:C

$tan θ = \frac{F_{1}}{F_{2}}$ $F_{1} = k . \frac{q_{1} q_{2}}{{(3)}^{2}}$ .
$F_{2} = k . \frac{q_{1} q_{3}}{{(4)}^{2}}$ .
$tan θ = \frac{\frac{k . q_{1} q_{2}}{{(3)}^{2}}}{\frac{k . q_{1} q_{2}}{{(4)}^{2}}} = \frac{{(4)}^{2}}{{(3)}^{2}} = \frac{16}{9}$ .
$tan θ = \frac{16}{9}$
$θ = {tan}^{- 1} (\frac{16}{9})$ .

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Three identical charges are placed at the vertices of an equilateral triangle. The force experienced by each charge, (if $k = \frac{1}{4} π ε_{0}$ ) is
A $2 K \frac{q^{2}}{r^{2}}$
B $\frac{K q^{2}}{2 r^{2}}$
C $\sqrt{3} K \frac{q^{2}}{r^{2}}$
D $\frac{K q^{2}}{\sqrt{2} r^{2}}$
Question 2 - Select One
Three charges are placed at the vertices of an equilateral trianlge of side $a$ as shown in the following figure. The force experienced by the charge placed at the vertex $A$ in a direction normal to $B C$ is

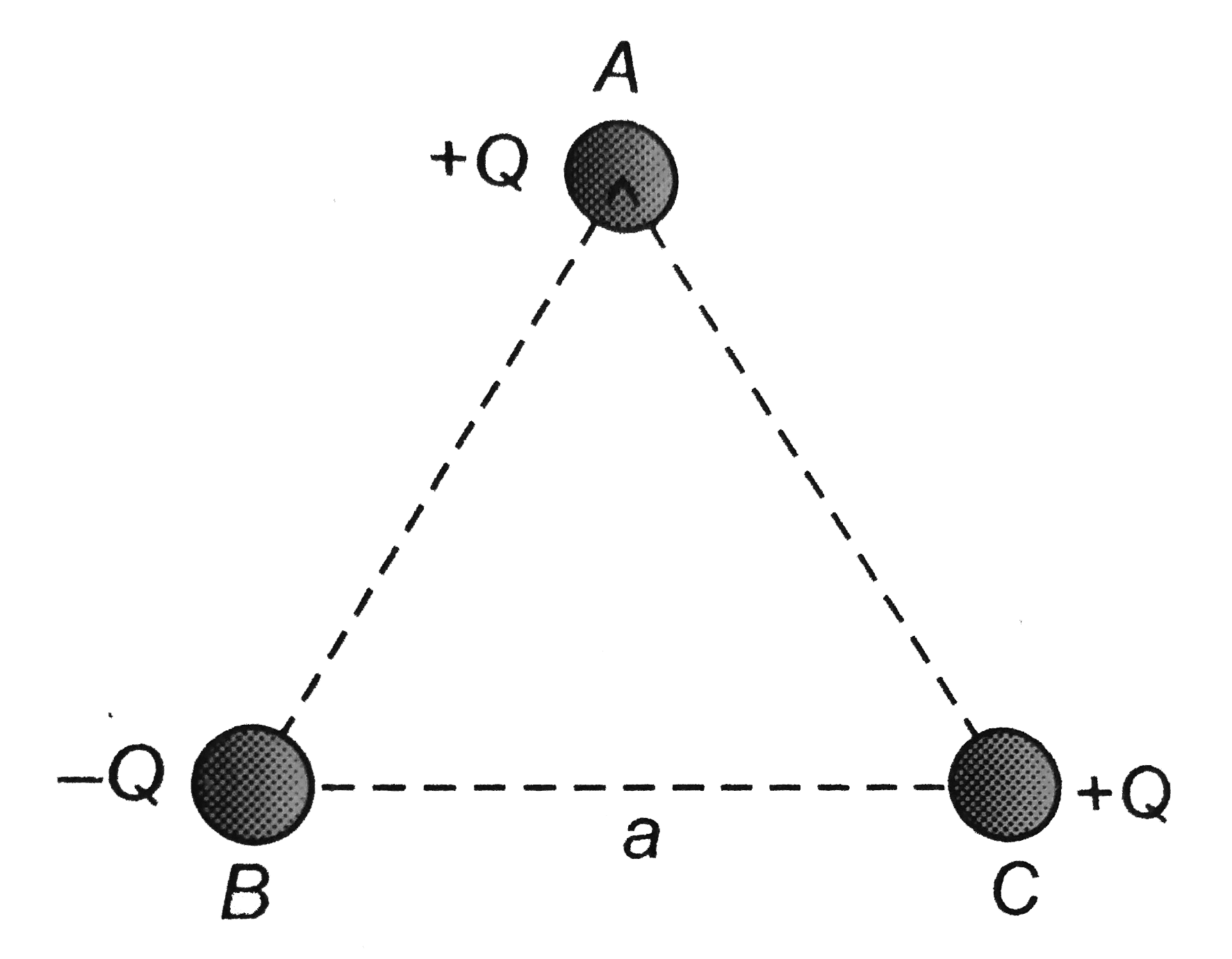A $\frac{Q^{2}}{4 π ε_{0} a^{2}}$
B $- \frac{Q^{2}}{4 π ε_{0} a^{2}}$
Czero
D $\frac{Q^{2}}{2 π ε_{0} a^{2}}$
Question 3 - Select One
Three identical point charges, q, are placed at the vertices of an equilateral triangle as shown in the figure. The length of each side of the triangle is d. Determine the magnitude and direction of the total electrostatic force on the charge at the top of the triangle.

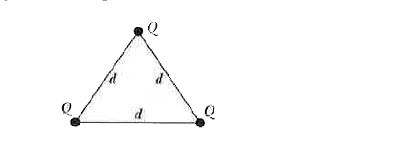A $\frac{Q^{2} \sqrt{3}}{4 π ε_{0} d^{2}}$ , directed upward
B $\frac{Q^{2} \sqrt{3}}{4 π ε_{0} d^{2}}$ , directed downward
C $\frac{Q^{2} \sqrt{3}}{2 π ε_{0} d^{2}}$ , directed upward
D $\frac{Q^{2} \sqrt{3}}{2 π ε_{0} d^{2}}$ , directed downward

SIA PUBLICATION-EAMCET -2016 (AP)-PHYSICS

Home
Ask Doubt
Profile