A mass of 2.9 kg is suspended from a string of length 50 cm and is at rest. Another body of mass 100 g, which is moving horizontally with a velocity of 150m/s strikes and sticks to it. Subsequently when the string makes an angle of 60∘ with the vertical, the tension in the string is (g = 10m/s2)Solution in Telugu
140 N
135 N
125 N
90 N
The correct Answer is:B
From Law of conservation of momentum
m1+m2U2=m1V1+m2V2
2.9×0+0.1×150=(2.9+0.1)V
v=5m/s
For circular motion,
Centripetal force = Net force towards centre
⇒m1+m2v21l=T−(m1+m2)gcos60∘
⇒T=3×9.8×12+3v210.5
=14.7+6V21
According to Law of conservation of mechanical energy,
K.E at lowest point = (K.E + P.E)
12(m1+m2)V2=12(m1+m2)V_(1)(2)+(m1+m2)gh
(12)(3)(5)2=12(3)V21+3×9.8×0.5(1−12)
[∵h=l(1−cosθ)=0.5(1−cos60∘)]
V21=20.1(m/sec)
On substituting V2 in equation (1),
T=14.7+6×20.1=135.3N.

Topper's Solved these Questions
Similar Questions
R.M.S. velocity of oxygen molecules at N.T.P. is 0.5 Km/s. The R.M.S. velocity of he hydrogen molecule at N.T.P. is
06:14View SolutionA circular loop and a square loop are formed from two wires of same length and cross section. Same current is passed through them. Then the ratio of their dipole moments is
03:56View SolutionA mass of 2.9kg is suspended from a string of length 50cm and is at rest . Another body of mass 100gm which is moving horizontal with a velocity of 150m/s strikes it . After striking the two bodies combine together . Tension in the string , when it is at an angle of 60∘ with the velocity is : g=10m/s2
04:48View SolutionA mass of 2.9 kg, is suspended from a string of length 50 cm, and is at rest. Another body of mass 100 gm moving horizontally with a velocity of 150 m/sec, strikes and sticks to it. What is the tension in the string when it makes an angle of 60º with the vertical
03:32View SolutionA particle of mass m suspended by a string of length l revolves in a horizontal circle (θ is the angle the string makes with vertical). Which of the following statement(s) is // are correct?
04:37View SolutionA mass of 2 kg tied to a string 1 m length is rotate in a verticle circle with a uniform speed of 4m/s. The tension in the string will be 52 N, when the mass is at (g=10m/s2)
02:28View SolutionA mass of 5 kg is tied to a string of length 1.0 m is rotated in vertical circle with a uniform speed of 4m/s . The tension in the string will be 170 N when the mass is at (g=10m/s2)
04:51View SolutionA mass of 5 kg is tied to a string of length 1.0 m and is rotated in vertical circle with a uniform speed of 4m//s . The tension in the string will be 130 N when the mass is at (g= 10 m//s^2 )
01:47View SolutionA 10 kg steel ball is suspended by two strings as shown. The tensions in the two strings are: (g=9.8 m/s2)
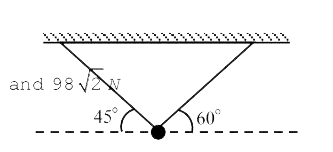 03:34View Solution
03:34View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m//s^(2))
05:14View SolutionA particle of mass 100 g is suspended from the end of a weightless string of length 100 cm and is rotated in a vertical plane. The speed of the particle is 200cm⋅s−1 when the string makes an angle of θ=60∘ with the verctcal. Determine
(i) the tension in the string when θ=60∘ andView SolutionA body of mass m is suspended by two strings making angles αandβ with the horizontal. Find the tensions in the strings.
View SolutionA mass of 3.9 kg suspended from a string of length 0.5 m is at rest. Another body of mass 0.1 kg moving horizontally with a velocity 200ms−1 strikes and stricks to it. The maximum angle through which the system swings just before the tension in the string becomes zero is
08:34View SolutionA mass of 2.9 kg is suspended from a string of length 50 cm and is at rest. Another body of mass 100 g which is moving horizontally with any velocity of 150 m/s strikes and sticks to it subsequently when the string makes an angle of 60∘ with the vertical. The tension in the string is (g=10m/s2)
05:14View SolutionA mass of 2.9 kg is suspended from a string of length 50 cm and is at rest. Another body of mass 100 g, which is moving horizontally with a velocity of 150m/s strikes and sticks to it. Subsequently when the string makes an angle of 60∘ with the vertical, the tension in the string is (g = 10m/s2)
03:38View Solution

