A particle of mass m moving in the x direction with speed 2v is hit by particle of mass 2m moving in the y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to:
0.44
0.5
0.56
0.62
The correct Answer is:C
To solve the problem, we need to calculate the percentage loss in kinetic energy during a perfectly inelastic collision between two particles. Let's break down the solution step by step:
Step 1: Identify the masses and velocities of the particles
- Particle 1 has mass m and is moving in the x-direction with speed 2v.
- Particle 2 has mass 2m and is moving in the y-direction with speed v.
Step 2: Calculate the initial kinetic energy of both particles
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
- For Particle 1:
KE1=12m(2v)2=12m⋅4v2=2mv2
- For Particle 2:
KE2=12(2m)v2=mv2
- Total initial kinetic energy KEinitial:
KEinitial=KE1+KE2=2mv2+mv2=3mv2
Step 3: Determine the final velocity after the collision
In a perfectly inelastic collision, the two particles stick together and move with a common velocity. We can use the conservation of momentum to find this velocity.
- Initial momentum in the x-direction:
px,initial=m⋅(2v)+0=2mv
- Initial momentum in the y-direction:
py,initial=0+(2m)⋅v=2mv
- Total initial momentum vector:
→pinitial=(2mv)^i+(2mv)^j
The total mass after the collision is:
mtotal=m+2m=3m
Using conservation of momentum:
→pinitial=→pfinal
(2mv)^i+(2mv)^j=(3m)→vfinal
Dividing by 3m:
→vfinal=(2v3^i+2v3^j)
Step 4: Calculate the magnitude of the final velocity
The magnitude of the final velocity vfinal is:
vfinal=√(2v3)2+(2v3)2=√4v29+4v29=√8v29=2√2v3
Step 5: Calculate the final kinetic energy
The final kinetic energy KEfinal is:
KEfinal=12(3m)(2√2v3)2=12(3m)⋅8v29=4mv23
Step 6: Calculate the change in kinetic energy
The change in kinetic energy ΔKE is:
ΔKE=KEfinal−KEinitial=4mv23−3mv2
=4mv23−9mv23=−5mv23
Step 7: Calculate the percentage loss in kinetic energy
The percentage loss in kinetic energy is given by:
Percentage Loss=(ΔKEKEinitial)×100
=⎛⎜⎝−5mv233mv2⎞⎟⎠×100=(−59)×100=−55.56%
Since we are interested in the magnitude of the loss:
Percentage Loss≈55.56%
Final Answer
The percentage loss in energy during the collision is approximately 56%.
---
- Particle 1 has mass m and is moving in the x-direction with speed 2v.
- Particle 2 has mass 2m and is moving in the y-direction with speed v.
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
KE1=12m(2v)2=12m⋅4v2=2mv2
KE2=12(2m)v2=mv2
KEinitial=KE1+KE2=2mv2+mv2=3mv2
In a perfectly inelastic collision, the two particles stick together and move with a common velocity. We can use the conservation of momentum to find this velocity.
px,initial=m⋅(2v)+0=2mv
py,initial=0+(2m)⋅v=2mv
→pinitial=(2mv)^i+(2mv)^j
mtotal=m+2m=3m
→pinitial=→pfinal
(2mv)^i+(2mv)^j=(3m)→vfinal
→vfinal=(2v3^i+2v3^j)
The magnitude of the final velocity vfinal is:
vfinal=√(2v3)2+(2v3)2=√4v29+4v29=√8v29=2√2v3
The final kinetic energy KEfinal is:
KEfinal=12(3m)(2√2v3)2=12(3m)⋅8v29=4mv23
The change in kinetic energy ΔKE is:
ΔKE=KEfinal−KEinitial=4mv23−3mv2
=4mv23−9mv23=−5mv23
The percentage loss in kinetic energy is given by:
Percentage Loss=(ΔKEKEinitial)×100
=⎛⎜⎝−5mv233mv2⎞⎟⎠×100=(−59)×100=−55.56%
Percentage Loss≈55.56%
The percentage loss in energy during the collision is approximately 56%.
Similar Questions
A particle of mass m moving with velocity 2v collides with another particle of mass 3m moving with velocity v in the same direction.If it is perfect inelastic collision,the loss of kinetic energy of the system is
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View Solution
Knowledge Check
A particle of mass m moving in the x direction with speed 2v is hit by another particle of mass 2m moving in they y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to :
A56%B62%C44%D50%A particle of mass m moving in the x direction with speed 2v is hit by another particle of mass 2m moving in they y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to :
Adevelopement of air current when the plate is placedBinduction of electrical charge on the plateCshielding of magnetic lines of force as aluminium is a paramagnetic material.Delectromagnetic induction in the aluminium plate giving rise to electromagnetic damping.A particle of mass m moving in the x direction with speed 2v is hit by another particle of mass 2m moving in they y direction with speed v. If the collision is perfectly inelastic, the percentage loss in the energy during the collision is close to :
A56%B62%C44%D50%
Two ball bearings of mass m each moving in opposite directions with same speed v collide head on with each other. If the collision is perfectly elastic, what will be the outcome of the collision ?
View SolutionTwo particles of mass 2m and m moving with speed v and 2v respectively perpendicular to each other collides perfectly inelastically, then
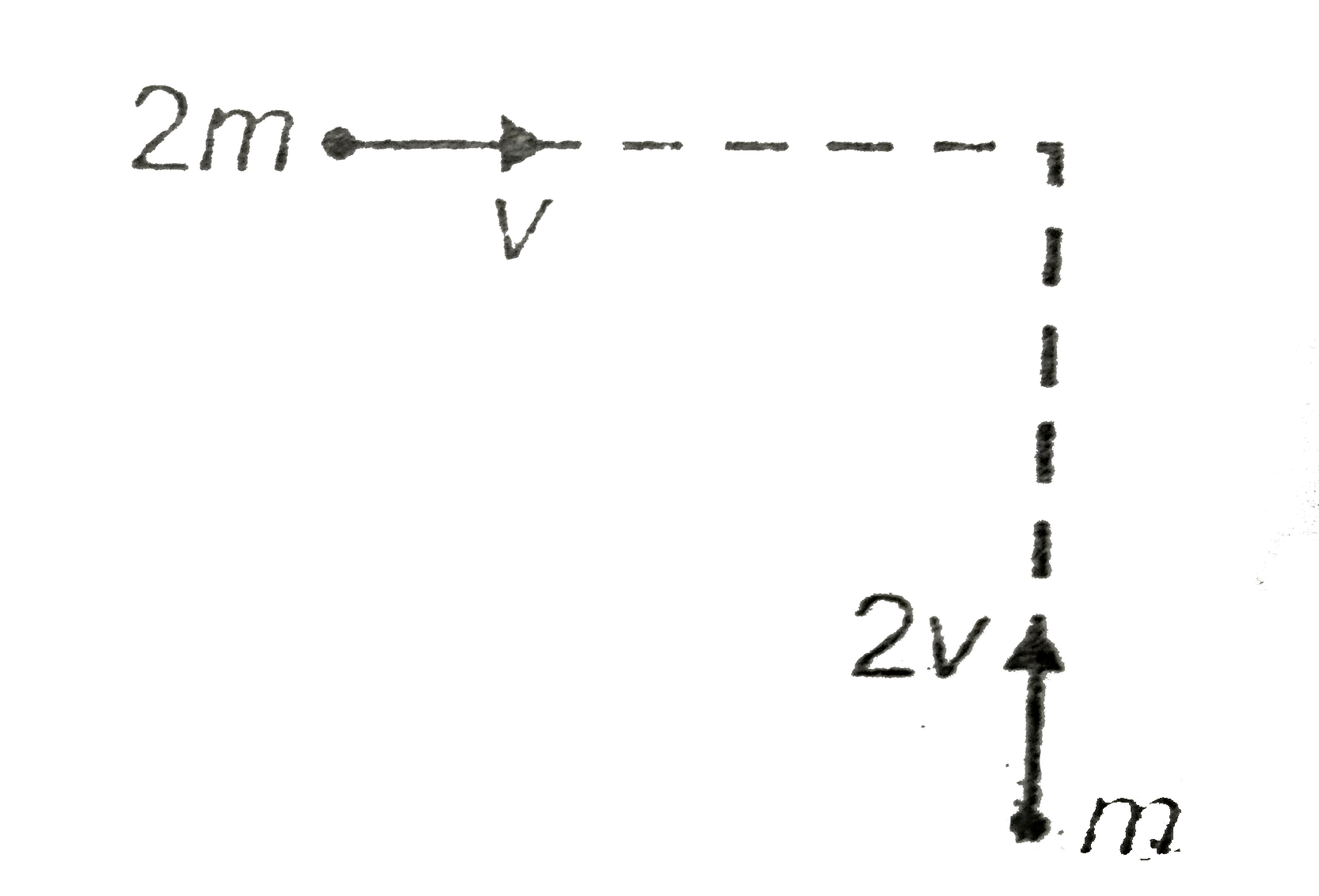
Loss in kinetic energy of the system
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 3 m at rest. Loss of KE of system in the collision is
View SolutionA particle of mass m moving with velocity v strikes a stationary particle of mass 2m and sticks to it. The speed of the system will be.
View SolutionA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
View Solution
