A wire of length 1 m and area of cross section 2×10−6m2 is suspended from the top of a roof at one end and a load of 20 N is applied at the other end. If the length of the wire is increased by 0.5×10−4m, calculate its Young’s modulus (in 1011N/m2).
The correct Answer is:2
To solve the problem, we need to calculate the Young's modulus of the wire using the given parameters. Let's break down the solution step by step.
Step 1: Write down the known values
- Length of the wire (L) = 1 m
- Area of cross-section (A) = 2 × 10^(-6) m²
- Force applied (F) = 20 N
- Change in length (ΔL) = 0.5 × 10^(-4) m
Step 2: Calculate the stress
Stress (σ) is defined as the force applied per unit area. The formula for stress is:
σ=FA
Substituting the values:
σ=20N2×10−6m2
Calculating this gives:
σ=202×10−6=10×106N/m2=107N/m2
Step 3: Calculate the strain
Strain (ε) is defined as the change in length per unit original length. The formula for strain is:
ϵ=ΔLL
Substituting the values:
ϵ=0.5×10−4m1m
Calculating this gives:
ϵ=0.5×10−4=5×10−5
Step 4: Calculate Young's modulus
Young's modulus (Y) is defined as the ratio of stress to strain. The formula for Young's modulus is:
Y=σϵ
Substituting the values we calculated:
Y=107N/m25×10−5
Calculating this gives:
Y=1075×10−5=2×1012N/m2
Step 5: Convert Young's modulus to the required form
The problem asks for Young's modulus in terms of 1011N/m2. We can express 2×1012N/m2 as:
Y=20×1011N/m2
Thus, in terms of 1011N/m2, the answer is:
Y=20
Final Answer
The Young's modulus of the wire is 20×1011N/m2.
---
- Length of the wire (L) = 1 m
- Area of cross-section (A) = 2 × 10^(-6) m²
- Force applied (F) = 20 N
- Change in length (ΔL) = 0.5 × 10^(-4) m
Stress (σ) is defined as the force applied per unit area. The formula for stress is:
σ=FA
Substituting the values:
σ=20N2×10−6m2
Calculating this gives:
σ=202×10−6=10×106N/m2=107N/m2
Strain (ε) is defined as the change in length per unit original length. The formula for strain is:
ϵ=ΔLL
Substituting the values:
ϵ=0.5×10−4m1m
Calculating this gives:
ϵ=0.5×10−4=5×10−5
Young's modulus (Y) is defined as the ratio of stress to strain. The formula for Young's modulus is:
Y=σϵ
Substituting the values we calculated:
Y=107N/m25×10−5
Calculating this gives:
Y=1075×10−5=2×1012N/m2
The problem asks for Young's modulus in terms of 1011N/m2. We can express 2×1012N/m2 as:
Y=20×1011N/m2
Thus, in terms of 1011N/m2, the answer is:
Y=20
The Young's modulus of the wire is 20×1011N/m2.
Similar Questions
A wire of length 1m and area of cross section 4×10−8m2 increases in length by 0.2 cm when a force of 16 N is applied. Value of Y for the material of the wire will be
View SolutionThe adjacent graph shows the extra extension (Δx) of a wire of length 1m suspended from the top of a roof at one end with an extera load Δw connected to the other end If the cross sectional area of the wire is 10−5m2 calculate the Young's modulus of the meterial of the wire
(A) 2×1011N/m2 (B) 2×10−11N/m2 (c) 3×10913)N/m3 (D) 2×1016N/m2
 .
.
View Solution
Knowledge Check
The adjacent graph shows the estension (Δl) of a wire of length 1m suspended from the top of a roof at one end and with a load W connected to the other end. If the cross-sectional area of the wire is 10−6m2, calculate the Young's modulus of the material of the wire.
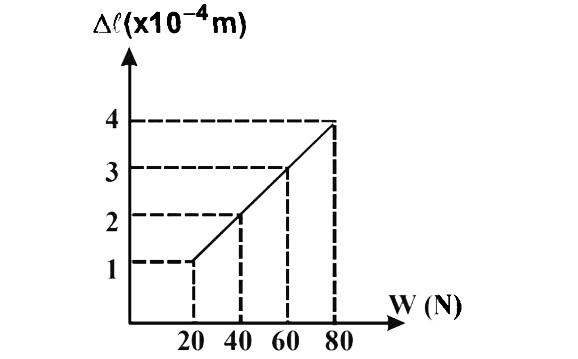 A(a) 2×1011N/mB(b) 2×10−11N/mC(c) 3×10−12N/mD(d) 2×10−13N/m
A(a) 2×1011N/mB(b) 2×10−11N/mC(c) 3×10−12N/mD(d) 2×10−13N/mThe adjacent graph shows the extension Δl of a wire of length 1m, suspended from the f top of a roof at one end and with a loaf w connected to the other end. If the cross-sectional area of the wire is 106m2 calculate the young's modulus of the material of the wire .
 A2×1011Nm−2B2×10−11Nm−2C3×10−12Nm2D2×10−13Nm2
A2×1011Nm−2B2×10−11Nm−2C3×10−12Nm2D2×10−13Nm2A wire of length 10 m and cross-section are 10−6m2 is stretched with a force of 20 N. If the elongation is 1 mm, the Young's modulus of material of the wire will be
A1010N/m2B1011N/m2C2×1010N/m2D2×1011N/m2
_S01_065_Q01.png)
The graph shows the extension of a wire of length 1m suspended from the top of a roof at one end and with a load W connected to the other end. If the cross sectional area of the wire is 1mm2, then the young's modulus of the material of the wire is
(a). 2×1011Nm−2
(b). 2×1010Nm−2
(c). 12×1011Nm−2
(d). none of these
View SolutionA steel wire of length 5 m and area of cross-section 4mm2 is stretched by 2 mm by the application of a force. If young's modulus of steel is 2×1011N/m2, then the energy stored in the wire is
View SolutionA copper wire of length 4.0mm and area of cross-section 1.2cm2 is stretched with a force of 4.8×103N. If Young's modulus for copper is 1.2×1011N/m2, the increases in the length of the wire will be
View SolutionThe length of a wire is 1.0m and the area of cross-section is 1.0×10−2cm2. If the work done for increase in length by 0.2cm is 0.4 joule, then Young's modulus of the material of the wire is
View SolutionA wire of area of cross section 1×10−6m2 and length 2 m is stretched through 0.1×10−3m. If the Young's modulus of a wire is 2×1011N/m2, then the work done to stretch the wire will be
View Solution
