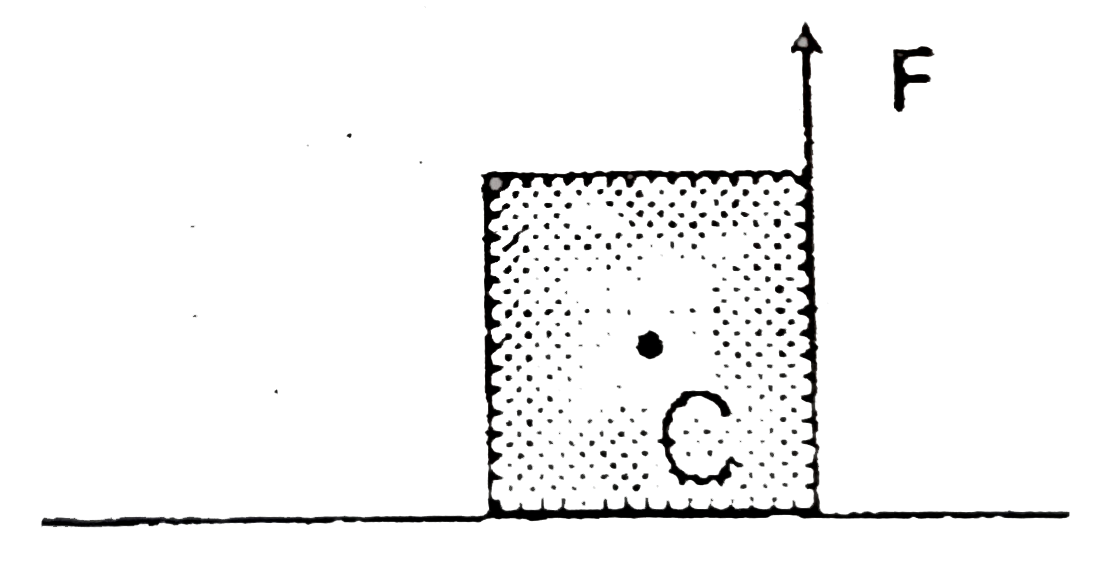
A uniform cube of mass m and side a is placed on a frictionless horizontal surface. A vertical force F is applied to the edge as shown in Fig. Match the following (most appropriate choice) :
(a)mg/4<F<mg/2 (i) Cube will move up.
(b) F>mg/2 (ii) Cube will not exhibit motion.
(c ) F>mg (iii) Cube will begin to rotate and slip at A.
(d) F=mg/4 (iv) Normal reaction effectively at a/3 from A, no motion.
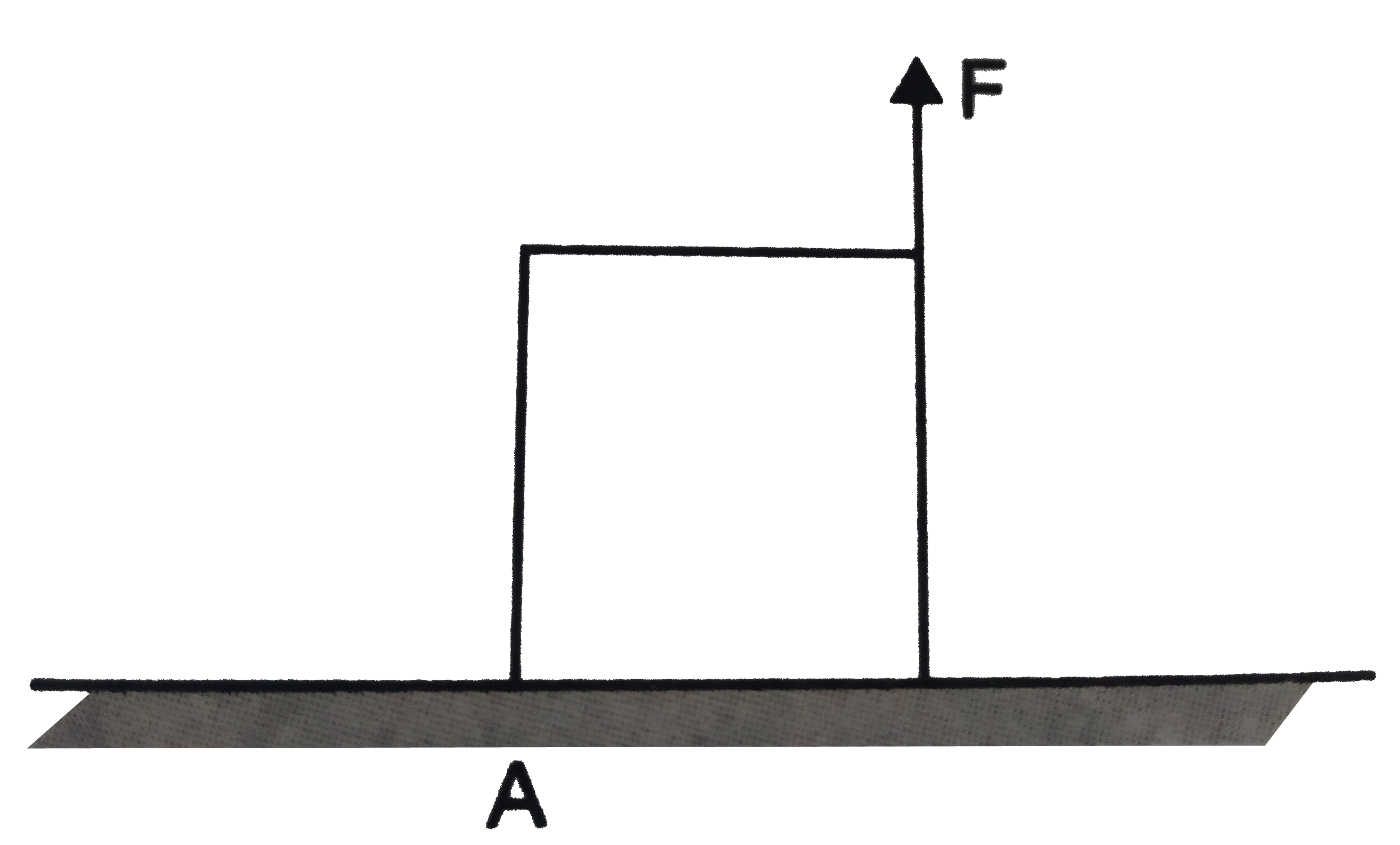
Consider the below diagram
Moment of the force F about point A τ1=F×a (anti - clockwise )
moment of weight mg of the cube about point A ,
τ2=mg×a2 (clock wise )
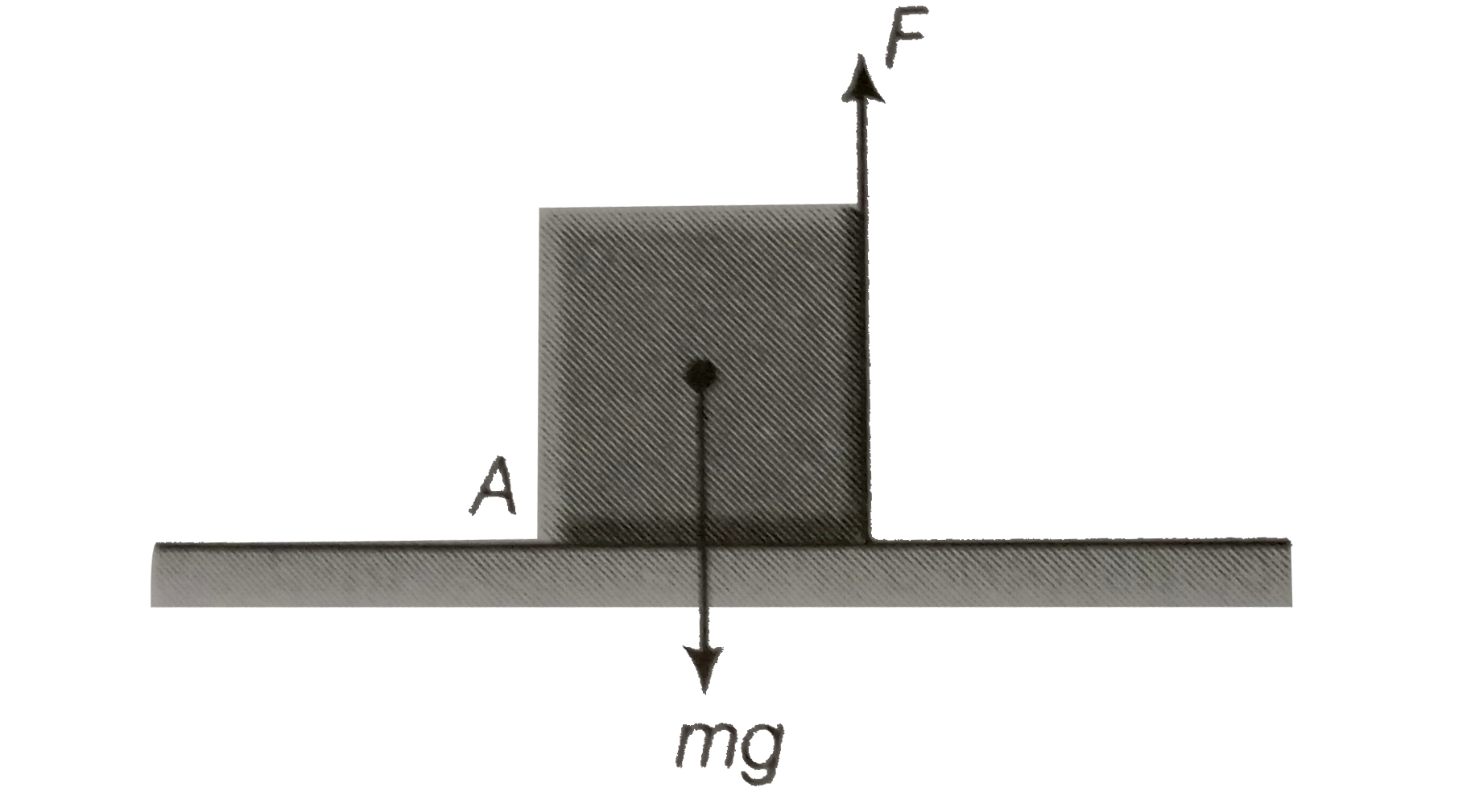
Cube will not exhibit motion ,if τ1=ta2
(∵ in this case , both the torque will cancel the effect the effect of each other )
theteforef×a=mg×a2⇒F=mg2
Cube will roate only when ,τ1>τ2
F×a>mg×a2⇒F>mg2
Let normal reaction is acting at a3 from point A , then
mg×a3=F×aorF=mg3
when F=mg4 which is less than mg3 ""(F<mg3)
there will be no motion .
∴(a)→(ii)(b)→(iii)(c)→(i)(d)→(iv)
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - Long answer type questions12videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - NCERT EXEMPLARCHAPTER - OSCILLATIONSEXERCISE - MULTIPLE CHOICE QUESTIONS (MCQs)40videos
OSCILLATIONS
- BOOK - NCERT EXEMPLARCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Very short Answer type Questions15videos
THERMAL PROPERTIES OF MATTER
Similar Questions
A uniform cube of mass m and side a is placed on a frictionless horizontal surface. A vertical force Fis applied to the edge as shown in figure. Match the following (most appropriate choice).

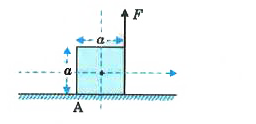 View Solution
View SolutionA unifrom cube of mass m and side a is placed on a frictionless horizontal surface .A vertical force F is applied to the edge as shown in figure ,match the following (most appropriate choice )
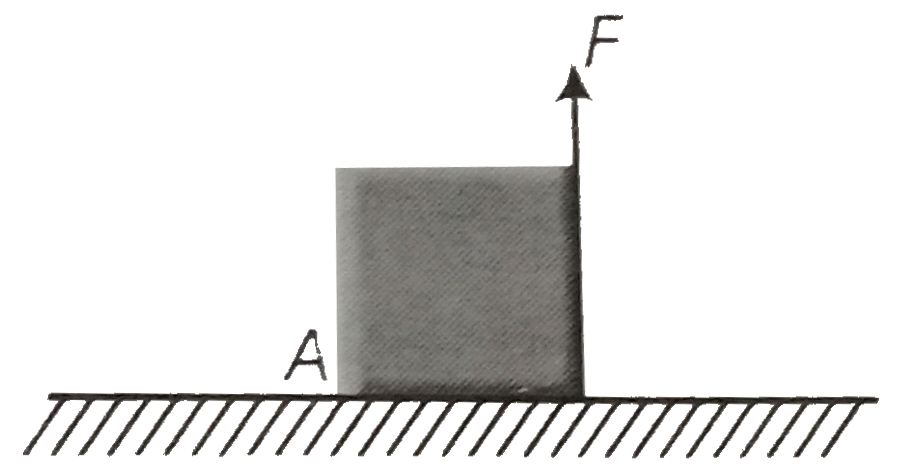 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A uniform cube of mass 4kg and side 30cm is placed on a fricitionless horizontal surface. A vertical force F is applied to the edge as shown in the figure. Choose the most appropriate option(s). (take g=10m/s2)
 ACube will move in the upward direction if F>40NBCube will not perform any type of translation or roataion motion if 10N<F<20NCCube will begin to perform rotational motion if 20N<F<40NDNormal reaction applied by the surface shifts to a distance 5cm from centre C of the cube if F=10N
ACube will move in the upward direction if F>40NBCube will not perform any type of translation or roataion motion if 10N<F<20NCCube will begin to perform rotational motion if 20N<F<40NDNormal reaction applied by the surface shifts to a distance 5cm from centre C of the cube if F=10N - Question 2 - Select One
A uniform cube of mass m and edge a moves on a horizontal surface along the positive x-axis, with initial velocity v0. Then
ADuring motion , NgtmgBDuring motion, normal reactions acts on the center of massCduring motion, the normal reaction shifts towards positive x-axis from the center of massDduring motion, normal reaction shifts in the direction of the force of friction. - Question 3 - Select One
A block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
A12B√3C1√3DNone
A cube of edge 2 cm and mass 10 g is placed on the surface of a table. What is the pressure exerted by the cube on the table?
View SolutionA cubical block of side a and of mass m rests on a rough horizontal surface. A horizontal force F is applied to normal to one of the faces at height h above the base h>a/2. What is the minimum value of F for which the cube begins to tip about an edge?
View SolutionTwo particle A & B each of mass m are in equilibrium in a vertical plane under action of a horizontal force F=mg on particle B, as shown in figure Then :
 View Solution
View SolutionA uniform cube of side and mass m rests on a rough horizontal surface. A horizontal force F is applied normal to one face at point that is directly above the centre of the face at a height a4 above the centre. The minimum value of F for which the cube begins to topple above an edge without sliding is
View SolutionA uniform cube of side a and mass m rests on a rough horizontal table. A horizontal force F is applied normal to one of the faces at a point directly above the centre of the face, at a height 3a4 above the base. What is the minimum value of F for which the cube begins to tip about an edge?
View Solution

