The area bounded by the curves $y = sin$ x, y= cos x and y-axis in 1 quadrant is -

A

$\sqrt{2}$

B

$\sqrt{2} + 1$

C

$\sqrt{2} - 1$

D

$\sqrt{2} + 2$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

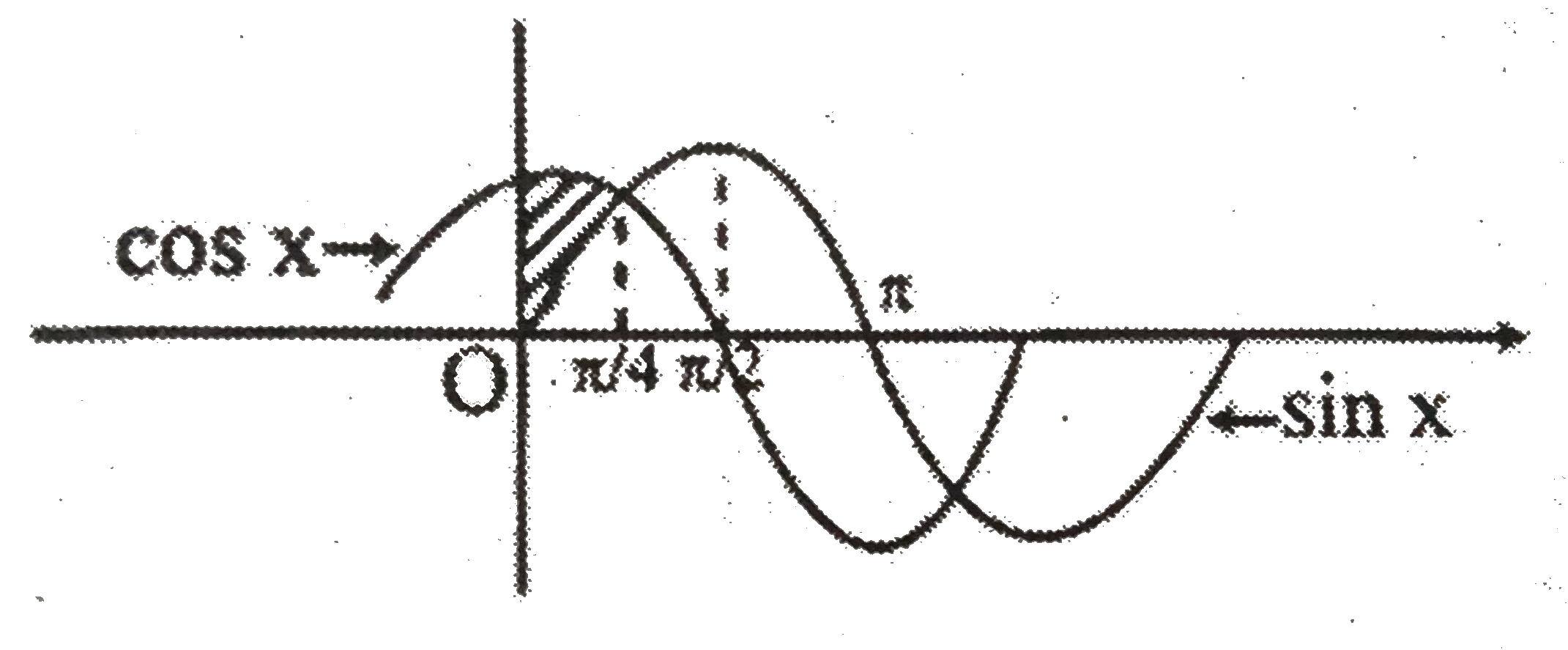
Required area $= π / 4 \int 0 (cosx -sin x) dx$
${(sin x + cos x)}_{0}^{π / 4}$
$(\frac{1}{\sqrt{2}} + \frac{1}{\sqrt{2}}) = (0 + 1) = \sqrt{2} - 1$

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

MOCK TEST 3BOOK - CAREER POINTCHAPTER - MOCK TEST 3EXERCISE - MATHS
30 Videos
MOCK TEST 5BOOK - CAREER POINTCHAPTER - MOCK TEST 5EXERCISE - Part (C) (MATHS)
30 Videos

Similar Questions

Knowledge Check

The area bounded by the curve $y = sin 2 x,$ axis and $y = 1,$ is
A1
B $1 / 4$
C $π / 4$
D $π / 4 - 1 / 2$
The area bounded by the curves $x + y = 2$ and $y = x^{2}$ above x-axis in the first quadrant is ,
A $\frac{1}{2}$
B $\frac{2}{3}$
C $\frac{10}{12}$
D $\frac{6}{5}$

CAREER POINT-MOCK TEST 4-MATHS

Home
Ask Doubt
Profile