If two sides of a triangle are unequal, the longer
side has greater angle opposite to it.
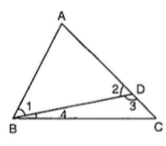
GIVEN : A ABC
in which AC>AB.
TO PROVE : ∠ABC>∠ACB
CONSTRUCTION : Mark a point D
on AC
such that AB=AD.
Joint BD.
Given: A △ABC in which AC>AB (say)
To prove: ∠ABC>∠ACB
Construction: Mark a point D on AC such that AB=AD. Join BD.
Proof: In △ABD
AB=AD (by construction)
∠1=∠2 …(i) (angles opposite to equal sides are equal)
Now in △BCD
∠2>∠DCB (ext. angle is greater than one of the opposite interior angles)
∠2>∠ACB …(ii) [since∠ACB=∠DCB]
From (i) and (ii), we get
∠1>∠ACB …(iii)
But ∠1 is a part of ∠ABC
∠ABC>∠1 …..(iv)
Now from (iii) and (iv), we get
∠ABC>∠ACB
Hence proved.
Topper's Solved these Questions
Similar Questions
Prove that any two sides of a triangle are together greater than twice the median drawn to the third side. GIVEN : ABC in which AD is a median. PROVE : AB+AC>2AD CONSTRUCTION : Produce AD to E such that AD=DE. Join EC.
View SolutionShow that the difference of any two sides of a triangle is less than the third side. GIVEN : ABC TO PROVE : (i) AC−AB<BC BC−AC<AB (iii) BC−AB<AC CONSTRUCTION : Take a point D on AC such that AD=AB. Join BD.
 View Solution
View SolutionThe sum of any two sides of a triangle is greater than the third side. GIVE : A ABC
View SolutionIn a right angled triangle, one acute angle is double the other. Prove that the hypotenuse is double the smallest side. GIVEN : A ABC in which ∠B=900 and ∠ACB=2∠CAB . to prove : AC=2BC CONSTRUCTION : Produce CB to D such that BD=CB. Join AD.
View SolutionShow that a median of a triangle divides it into two triangles of equal area. GIVEN : A ABC in which AD is the median. TO PROVE : ar(ABD)=ar(ADC) CONSTRUCTION : Draw AL⊥BC
View SolutionAngles opposite to two equal sides of a triangle are equal. GIVEN : ABC in which AB=AC TO PROVE : ∠C=∠B CONSTRUCTION : Draw the bisector AD of ∠A which meets BC in D Figure
View SolutionThe area of a triangle is half the product of any of its sides and the corresponding altitude. GIVEN : A ABC in which AL is the altitude to the side BC. TO PROVE : ar(ABC)=12(BC×AL) CONSTRUCTION : Through C and A draw CDBA and ADBC respectively, intersecting each other at D.
View SolutionShow that the sum of the three altitudes of a triangle is less than the sum of three sides of the triangle. GIVEN : A ABC in which AD⊥BC,BE⊥AC and CF⊥AB. PROVE : AD+BE+CF<AB+BC+AC
View SolutionShow that the difference of any two sides of a triangle is less than the third side. Given : A ABC To Prove: (i) AC−AB<BC BC−AC<AB (iii) BC−AB<AC Construction: Take point D on AC such that AD=AB . Join BD
View SolutionProve that the area of an equilateral triangle is equal to √34a2, where a is the side of the triangle. GIVEN : An equilateral triangle ABC such that AB=BC=CA=a. TO PROVE : ar(ABC)=√34a2 CONSTRUCTION : Draw AD⊥BC.
View Solution

