ABCD is a rhombus with ∠ABC=560. Determine ∠ACD.
To solve the problem, we need to determine the angle ∠ACD in the rhombus ABCD given that ∠ABC=56∘.
1. Understand the properties of a rhombus: In a rhombus, opposite angles are equal, and adjacent angles are supplementary.
2. Identify the given angle: We are given ∠ABC=56∘.
3. Determine the opposite angle: Since opposite angles in a rhombus are equal, we have:
∠ADC=∠ABC=56∘
4. Find the adjacent angle: The adjacent angles in a rhombus are supplementary, which means:
∠ABC+∠BCD=180∘
Substituting the known value:
56∘+∠BCD=180∘
Therefore,
∠BCD=180∘−56∘=124∘
5. Use the diagonal property: The diagonals of a rhombus bisect the angles. Thus, the angle ∠BCD is bisected by diagonal AC:
∠BCD=2×∠ACD
Hence,
∠ACD=∠BCD2=124∘2=62∘
6. Final answer: Therefore, the angle ∠ACD is:
∠ACD=62∘
Summary:
The angle ∠ACD in rhombus ABCD is 62∘.
∠ADC=∠ABC=56∘
∠ABC+∠BCD=180∘
Substituting the known value:
56∘+∠BCD=180∘
Therefore,
∠BCD=180∘−56∘=124∘
∠BCD=2×∠ACD
Hence,
∠ACD=∠BCD2=124∘2=62∘
∠ACD=62∘
The angle ∠ACD in rhombus ABCD is 62∘.
Topper's Solved these Questions
Similar Questions
If ABCD is a rhombus with ∠ABC=56∘, find the measure of ∠ACD
View SolutionABCD is a parallelogram in which diagonal AC bisects ∠BAD. If ∠BAC=35∘ , then ∠ABC= 70∘ (b) 110∘ (c) 90∘ (d) 120∘
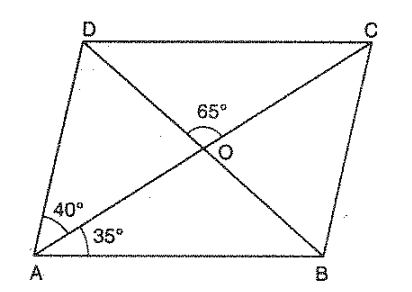
View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
 View Solution
View SolutionThe diagonals AC and BD of a rectangle ABCD intersect each other at P. If ∠ABD=50∘, then ∠DPC= 70∘ (b) 90∘ (c) 80∘ (d) 100∘
View SolutionIn a ΔABC; if 2∠A=3∠B=6∠C; Determine ∠A;∠Band∠C
View SolutionIn a ABC, if 2∠A=3∠B=6∠C, determine ∠A,∠B and ∠C.
View SolutionIn Figure, AOB is a straight line. If ∠AOC+∠BOD=850, then ∠COD= (a)850 (b) 900 (c) 950 (d) 1000
View SolutionIn a ABC, if 2∠A=3∠b=6 ∠c, determine ∠A, ∠B and ∠C
View SolutionABCD is a cyclic quadrilateral in which: BCAD, ∠ADC=1100 and ∠BAC=500. Find ∠DAC ∠DBC=800 and ∠BAC=400. Find ∠BCD ∠BCD=1000and ∠ABD=700 find ∠ADB
View SolutionABCD is a rhombus. Show that diagonal AC bisects ∠Aas well as ∠Cand diagonal BD bisects ∠B as well as ∠D.
View Solution

