ABC is a triangle. D is a point on AB such that AD=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC.
To prove that DE=14BC given that AD=14AB and AE=14AC, we can follow these steps:
Step 1: Define Points and Ratios
Let A,B,C be the vertices of triangle ABC. We know:
- D is a point on AB such that AD=14AB.
- E is a point on AC such that AE=14AC.
Step 2: Express Lengths in Terms of Vectors
We can express the positions of points A,B,C,D,E using vectors:
- Let →A be the position vector of point A.
- Let →B be the position vector of point B.
- Let →C be the position vector of point C.
Using the section formula, we can find the position vectors of points D and E:
- The position vector of D is given by:
→D=→A+14(→B−→A)=34→A+14→B
- The position vector of E is given by:
→E=→A+14(→C−→A)=34→A+14→C
Step 3: Find the Vector DE
Now, we can find the vector →DE:
→DE=→E−→D=(34→A+14→C)−(34→A+14→B)
Simplifying this gives:
→DE=14→C−14→B=14(→C−→B)
Step 4: Relate DE to BC
From the above expression, we see that:
→DE=14(→C−→B)=14BC
Thus, we have shown that:
DE=14BC
Conclusion
We have proved that DE=14BC.
---
Let A,B,C be the vertices of triangle ABC. We know:
- D is a point on AB such that AD=14AB.
- E is a point on AC such that AE=14AC.
We can express the positions of points A,B,C,D,E using vectors:
- Let →A be the position vector of point A.
- Let →B be the position vector of point B.
- Let →C be the position vector of point C.
- The position vector of D is given by:
→D=→A+14(→B−→A)=34→A+14→B
- The position vector of E is given by:
→E=→A+14(→C−→A)=34→A+14→C
Now, we can find the vector →DE:
→DE=→E−→D=(34→A+14→C)−(34→A+14→B)
Simplifying this gives:
→DE=14→C−14→B=14(→C−→B)
From the above expression, we see that:
→DE=14(→C−→B)=14BC
Thus, we have shown that:
DE=14BC
We have proved that DE=14BC.
Topper's Solved these Questions
Similar Questions
ABC is a triangle. D is a point on AB such that D=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC
View SolutionABCD is a parallelogram. P is a point on AD such that AP=13 AD and Q is a point on BC such that: CQ=13BC. Prove that AQCP is a parallelogram.
View SolutionABCD is a parallelogram. E is a point on BA such that BE=2 EA and F is a point on DC such that DF=2 FC. Prove that AE CF is a parallelogram whose area is one third of the area of parallelogram AB CD.
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE and F is the point of BC such that BF=2FC. Prove that : ar(ADEG)=ar(GBCE) ar(EGB)=16ar(ABCD)
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE andF is the point of BC such that BF=2FC. Prove that: ar(EBG)=ar(EFC)
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE and F is the point of BC such that BF=2FC. Prove that: arEGB=16ar(ABCD)
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE and F is the point of BC such that BF=2FC. Prove that: ar(EFC)=12ar(EBF)
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE andF is the point of BC such that BF=2FC. Prove that: ar(ADEG)=ar(GBCE)
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB ,E is a point of DC such that CE=2DE and F is the point of BC such that BF=2FC. Prove that: Find what portion of the area of parallelogram is the area of EFG
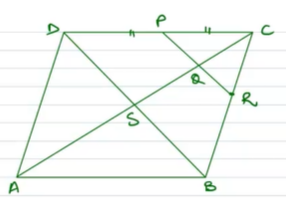
View SolutionIn Figure, ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that CQ=14AC. If PQ produced meets BC at R. Prove that R is a mid-point of BC.
 View Solution
View Solution

