A non-homogeneous cylinder of radius "R" carries a current I whose current density varies with the radial "distance "r" from the centre of the rod as J=σr where σ is a constant.The magnetic field at a point "P" at a distance r<R from the centre of the rod is
Step by step video & image solution for A non-homogeneous cylinder of radius "R" carries a current I whose current density varies with the radial "distance "r" from the centre of the rod as J=sigma r where sigma is a constant.The magnetic field at a point "P" at a distance r<R from the centre of the rod is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A long cylindrical conductor of radius R carries a current i as shown in the figure. The current density J varies across the cross-section as J=kr2, where, k is a constant. Find an expression for the magnetic field B at a distance r(<R) from the axis
 View Solution
View SolutionA long cylidrical conductor of radius R carries a current i as shown in figure. The current desity J is a function of radius according to J=br, where b is a constant. Find an expression for the magnetic field B
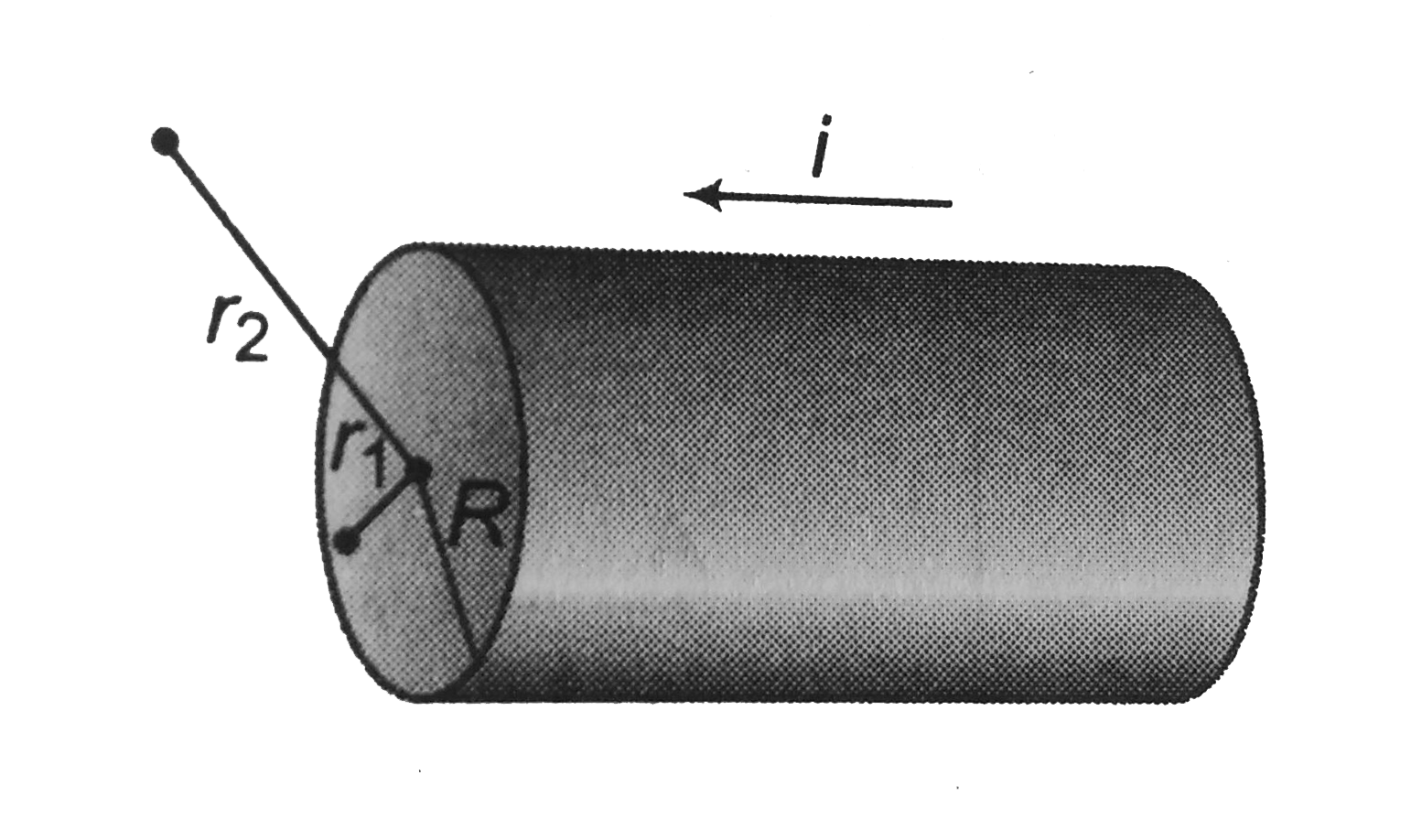
a. at a distasnce r1<R and
b.at a distance r2>R, measured from the axis.View Solution
Knowledge Check
- Question 1 - Select One
A sphere of radius R has a charge density σ. The electric intensity at a point at a distance r from its centre is
AE=σR2∈0kr2BE=σ∈0kCE=R2DE=∈0kr2σ2 - Question 2 - Select One
A circular coil of radius R carries a current i. The magnetic field at its centre is B. The distance from the centre on the axis of the coil where the magnetic field will be B/8 is
A√2RB√3RC2RD3R - Question 3 - Select One
Magnetic field due to an infinite current carrying wire varies with the distance (r) from the wire as
ArBr2C1rD1r2
A cylindrical metal rod of radius "R" carries a current l .The velocity of free electrons drifting at a distance r(
View SolutionThe current density in a wire of radius a varies with radial distance r as J=J0r2a, where J0 is a constant. Choose the incorrect statement.
View SolutionA long cylindrical conductor of radius R carries I as shown. The current density is a function of radius r as j = br, where b is a constant. The magnetic field at a distance r1(r1<R) is
 View Solution
View SolutionThe magnetic field at a distance r from a long wire carryimg current I is 0.4 T. The magnetic field at a distance 2r is
View SolutionAn infinitely long cylinderical wire of radius R is carrying a current with current density j=αr3 (where α is constant and r is the distance from the axis of the wire). If the magnetic fixed at r=R2 is B1 and at r=2R is B2 then the ratio B2B1 is
View Solution
