A ring of radius R is rotating about axis of ring such that angular velocity is given as = 5t. Find acceleration of a point P on rim after 5 sec
5R
25R
√650R
None of these
The correct Answer is:D
To solve the problem of finding the acceleration of a point P on the rim of a rotating ring after 5 seconds, we can break down the solution into several steps:
Step 1: Understand the given information
We know that the angular velocity ω of the ring is given by:
ω=5t
where t is the time in seconds. The radius of the ring is R.
Step 2: Calculate the angular velocity at t=5 seconds
To find the angular velocity after 5 seconds, we substitute t=5 into the equation for ω:
ω=5×5=25rad/s
Step 3: Find the tangential acceleration
The tangential acceleration at can be calculated using the formula:
at=αR
where α is the angular acceleration. Angular acceleration α is the derivative of angular velocity with respect to time:
α=dωdt=d(5t)dt=5rad/s2
Now substituting α into the equation for at:
at=5×R=5R
Step 4: Calculate the centripetal acceleration
Centripetal acceleration ac is given by:
ac=ω2R
Substituting the value of ω we found earlier:
ac=(25)2R=625R
Step 5: Calculate the resultant acceleration
The resultant acceleration a is the vector sum of the tangential and centripetal accelerations. Since these two accelerations are perpendicular to each other, we can use the Pythagorean theorem:
a=√a2t+a2c
Substituting the values we calculated:
a=√(5R)2+(625R)2
=√25R2+390625R2
=√390650R2
=R√390650
Step 6: Final result
The acceleration of point P on the rim after 5 seconds is:
a≈625.02R
We know that the angular velocity ω of the ring is given by:
ω=5t
where t is the time in seconds. The radius of the ring is R.
To find the angular velocity after 5 seconds, we substitute t=5 into the equation for ω:
ω=5×5=25rad/s
The tangential acceleration at can be calculated using the formula:
at=αR
where α is the angular acceleration. Angular acceleration α is the derivative of angular velocity with respect to time:
α=dωdt=d(5t)dt=5rad/s2
Now substituting α into the equation for at:
at=5×R=5R
Centripetal acceleration ac is given by:
ac=ω2R
Substituting the value of ω we found earlier:
ac=(25)2R=625R
The resultant acceleration a is the vector sum of the tangential and centripetal accelerations. Since these two accelerations are perpendicular to each other, we can use the Pythagorean theorem:
a=√a2t+a2c
Substituting the values we calculated:
a=√(5R)2+(625R)2
=√25R2+390625R2
=√390650R2
=R√390650
The acceleration of point P on the rim after 5 seconds is:
a≈625.02R
Similar Questions
Knowledge Check
A thin circular ring of mass M and radius R is rotating about its axis with constant angular velocity ω. The objects each of mass m are attached gently to the ring. The wheel now rotates with an angular velocity.
AωM(m+M)Bω(M−2M)(M+2m)CωM(M+2m)Dω(M+2m)MA ring of radius r is rotating about a vertical axis along its diameter with constant angular velocity ω.A read of mass m remains at rest w.r.t. ring at the position shown in figure. Then w2 is:
_2019_CBT8_E01_015_Q01.png) Ag√3B2g√3rC√3grDgr
Ag√3B2g√3rC√3grDgrA uniform circular ring of radius R is first rotated about its horizontal axis with an angular velocity ω0 and then carefully placed on a rough horizontal surface as shown. The coefficient of friction between the surface and the rings μ. Time after which its angular speed is reduced to 0.5ω0 is
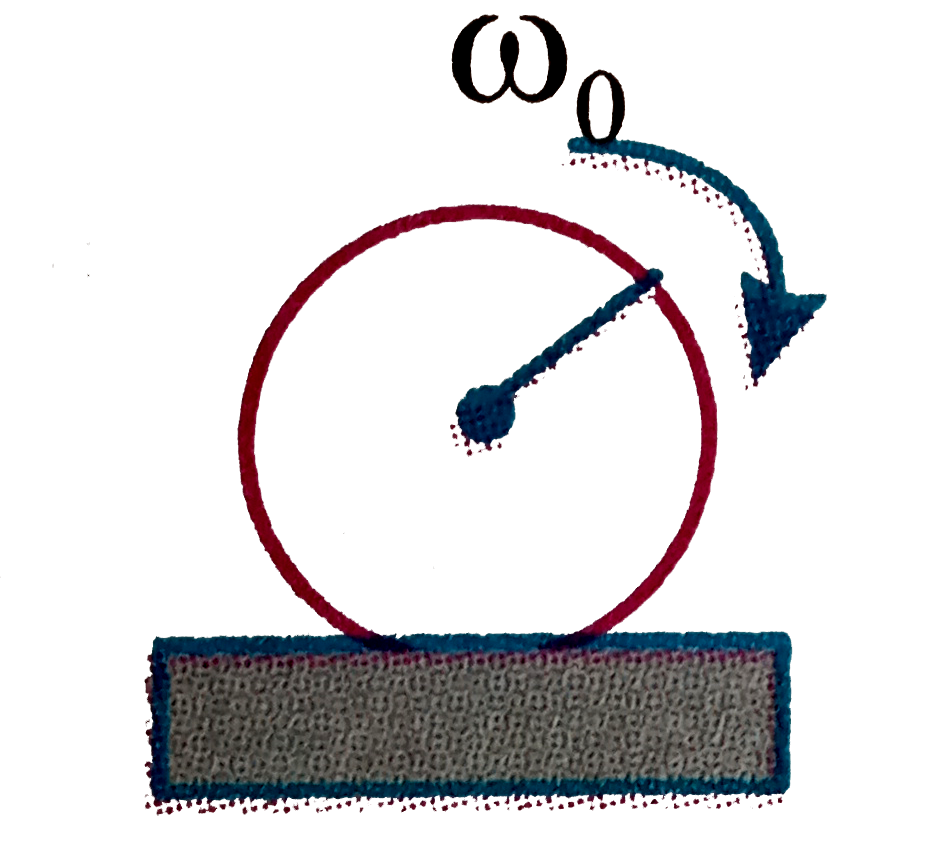 Aω0μR2gBω0g2μRC2ω0RμgDω0R2μg
Aω0μR2gBω0g2μRC2ω0RμgDω0R2μg
A disc of radius 10 cm is rotating about its axis at an angular speed of 20 rad/s. Find the linear speed of
a. a point on the rim,
b. the middle point of a radius.
View SolutionA ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA thin circular ring of mass m and radius R is rotating about its axis with a constant angular velocity ω. Two objects each of mass M are attached gently to the opposite ends of a diameter of the ring. The ring now rotates with an angular velocity ω'=
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with a constant angular velocity omega. Four objects each of mass m, are kept gently to the opposite ends of two perpendicular diameters of the ring. The angular velocity of the ring will be
View SolutionA ring of radius 0.5 m and mass 10 kg is rotating about its diameter with angular velocity of 20 rad/s. Its KE is :-
View Solution
