A thin smooth tube of length 2 m containing a small pallet of mercury in it is rapidly inverted 50 times. If capillary effect is neglected find the approximate increase in temperature of the mercury. Given that the specific heat of mercury is 30 cal/kg K.
The correct Answer is:7.8∘C
To solve the problem, we need to calculate the increase in temperature of the mercury after it has been inverted 50 times in a thin tube of length 2 m. We will use the principles of potential energy and specific heat.
1. Identify the potential energy change:
When the tube is inverted, the mercury moves up and down. The change in height (h) for each inversion is the length of the tube, which is 2 m. The potential energy change (PE) for one inversion can be calculated using the formula:
PE=m⋅g⋅h
where:
- m = mass of mercury (in kg)
- g = acceleration due to gravity (approximately 9.81m/s2)
- h = height change (2 m)
2. Calculate total potential energy change after 50 inversions:
Since the mercury is inverted 50 times, the total potential energy change (PE_total) will be:
PEtotal=50⋅(m⋅g⋅h)=50⋅(m⋅9.81⋅2)
Simplifying this gives:
PEtotal=50⋅m⋅19.62
3. Relate potential energy to temperature change:
The change in thermal energy (Q) of the mercury can be expressed using the specific heat formula:
Q=m⋅s⋅ΔT
where:
- s = specific heat of mercury (30 cal/kg K)
- ΔT = change in temperature (in K)
4. Set the potential energy equal to thermal energy:
Since the energy gained by the mercury is equal to the potential energy lost, we can set the two equations equal:
50⋅m⋅19.62=m⋅30⋅ΔT
5. Cancel mass (m):
Since mass appears on both sides of the equation, we can cancel it out:
50⋅19.62=30⋅ΔT
6. Solve for ΔT:
Rearranging the equation to solve for ΔT gives:
ΔT=50⋅19.6230
Calculating this:
ΔT=98130≈32.7K
7. Final approximation:
The approximate increase in temperature of the mercury is about 32.7 K.
When the tube is inverted, the mercury moves up and down. The change in height (h) for each inversion is the length of the tube, which is 2 m. The potential energy change (PE) for one inversion can be calculated using the formula:
PE=m⋅g⋅h
where:
- m = mass of mercury (in kg)
- g = acceleration due to gravity (approximately 9.81m/s2)
- h = height change (2 m)
Since the mercury is inverted 50 times, the total potential energy change (PE_total) will be:
PEtotal=50⋅(m⋅g⋅h)=50⋅(m⋅9.81⋅2)
Simplifying this gives:
PEtotal=50⋅m⋅19.62
The change in thermal energy (Q) of the mercury can be expressed using the specific heat formula:
Q=m⋅s⋅ΔT
where:
- s = specific heat of mercury (30 cal/kg K)
- ΔT = change in temperature (in K)
Since the energy gained by the mercury is equal to the potential energy lost, we can set the two equations equal:
50⋅m⋅19.62=m⋅30⋅ΔT
Since mass appears on both sides of the equation, we can cancel it out:
50⋅19.62=30⋅ΔT
Rearranging the equation to solve for ΔT gives:
ΔT=50⋅19.6230
Calculating this:
ΔT=98130≈32.7K
The approximate increase in temperature of the mercury is about 32.7 K.
Topper's Solved these Questions
- HEAT AND THERMAL EXPANSIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT AND THERMAL EXPANSIONEXERCISE - ADVANCE MCQs WITH ONE OR MORE OPTIONIS CORRECT
24 Videos
- GEOMETRICAL OPTICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GEOMETRICAL OPTICSEXERCISE - Unsolved Numerical Problems
107 Videos
- HEAT TRANSFER BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT TRANSFER EXERCISE - Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO
66 Videos
Similar Questions
A tube of length 2m containing a little mercury and closed at both ends is rapidly inverted 50 times. What is the maximum rise in temperature expected? (Specific heat capacity of mercury =30calkg−1K−1 and J=4.2 joules//cal.)
View SolutionIn a mixture of 35g of ice and 35g of water in equilibrium, 4gm steam is passed. The whole mixture is in a copper calorimeter of mass 50g. Find the equilibrium temperature of the mixture. Given that specific heat of water is 4200 J/kg K and that of copper is 420 J/Kg K and latent heat of fusion of ice is 3.36×105 J/kg and latent heat of vaporization of water is 2.25×106 J/kg.
View Solution
Knowledge Check
Two identical glass spheres filled with air are connected by a thin horizontal glass tube the glass tube contains a pellet of mercury at its mid-point Air in one sphere is at 0∘C and the other is at 20∘C if temperature of both the vessels are increased by 10∘C then neglecting the expansions of the bulbs and the tube
Athe mercury pellet gets displaced towards the sphere at lower temperatureBthe mercury pellet gets displaced towards the sphere at higher temperatureCthe mercury pellet does not get displaced at allDthe temperature rise causes the pellet to expand without any displacement
A copper calorimeter of mass 190 g contains 300 g of water at 0∘C and 50 g of ice at 0∘C.Find the amount of steam at 100∘C required to raise the temperature of this mixture by 10∘C. Given that the specific heat of copperis 420J/kg∘C, latent heat of vaporization of water is 2.25×105×J/kg and latent heat of fusion of ice is 3.36×105J/kg.
View SolutionMercury has an angle of contact equal to 140∘ with soda lime galss. A narrow tube of radius 1.00mm made of this glass is dipped in a through containing mercury. By what amount does the mercury dip down in the tube relative to the mercury surface outside? Surface tension of mercury at the temperature of the experiment is 0.465Nm−1. Density of mercury = 13.6×103kgm−3.
View SolutionWhen 20×10−3 kg of water at 15∘C is placed in the tube of an ice calorimeer, it si found that the mercury thread mvoes through 29cm. If a metal of mass 12g and 100∘C is placed in the tube, the mercury thread contracts by 12cm. Find the specific heat capacity of metal. (Specific heat capacity of water =4200Jkg−1K−1)
View SolutionA closed card board tube is 50 cm in length an contains 200g of lead shots which occupy 2 cm of the length of the tube when it is held vertically. The tube is inverted quickly so that the end which was previously above is now below. This process is repeated 400 times. If the specific heat of lead is 0.32calg−1.∘C−1, calculate the rise in temperature of the lead shots.
View SolutionFigure-4.13 shows a water tank at a constant temperature. T0 and a small bodyof mass m, and specific heat S at a temperature T1 . Given that T1<T0. A metal rod of length L, cross-sectional area A whose thermal conductivity is K is placed between the tank and the body to connect than. Find the temperature of body as a function of time. Given that the heat capacity of rod is negligible.
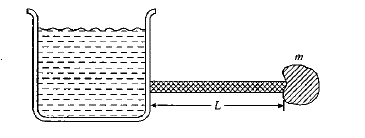
View SolutionIn given figure, A uniform cylindrical tube closed at one end, contains a pallet of mercury 5cm long. When the tube is kept verticaly with the closed end downward, the length of the air column trapped is 30cm. Now the tube is inverted so that the closed end goes up find the new length of the air column trapped. atmospheric pressure =75cm of mercury. (Assume temperature remains constant in this process)

View SolutionA glass tube of 1 mm bore is dipped vertically into a container of mercury, with its lower end 5 cm below the mercury surface. What must be the gauge pressure of air in the tube to a hemispherical bubble at its lower end? Given density of mercury =13.6×103kg/m3, surface tension of mercury = 440×10−3Nm−1 and g=10m/s2
View Solution
