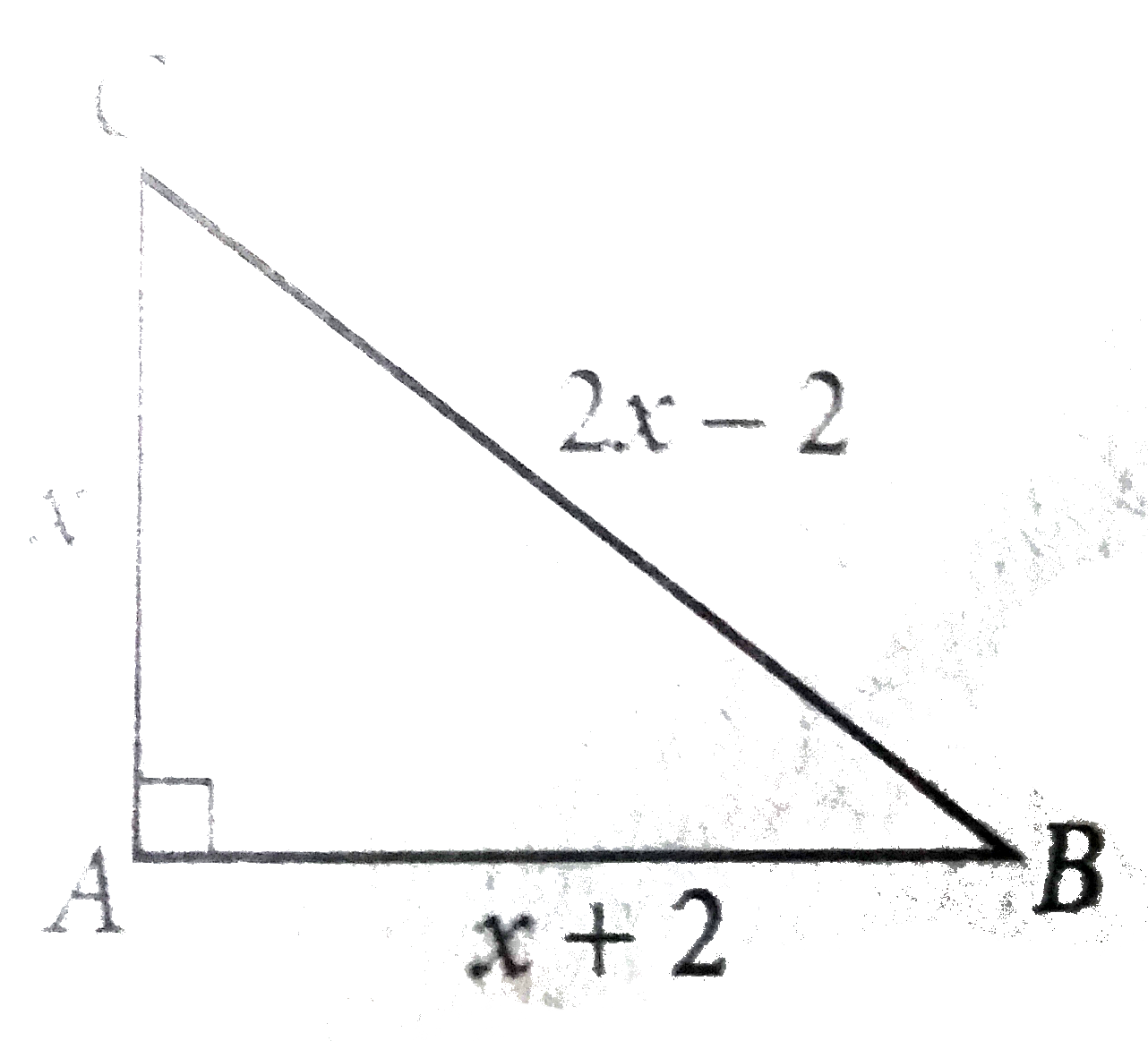Which three-dimensional figure is formed by rotating a right triangle about a leg ?
Prism
sphere
cone
cylinder
The correct Answer is:C
To determine which three-dimensional figure is formed by rotating a right triangle about one of its legs, we can follow these steps:
Step 1: Understand the Right Triangle
A right triangle consists of one right angle (90 degrees) and two other angles that add up to 90 degrees. The two legs of the triangle are perpendicular to each other.
Hint: Visualize the right triangle and label the legs as 'a' and 'b', with the hypotenuse being 'c'.
Step 2: Identify the Axis of Rotation
When we rotate the right triangle, we need to choose one of its legs as the axis of rotation. Let's assume we rotate the triangle around one of the legs, say leg 'a'.
Hint: Think about how the triangle will move in space when it is rotated around leg 'a'.
Step 3: Visualize the Rotation
As we rotate the triangle around leg 'a', the other leg 'b' will sweep out a circular path. The rotation creates a three-dimensional shape.
Hint: Imagine the leg 'b' moving in a circular motion around leg 'a' while maintaining its length.
Step 4: Determine the Shape Formed
The shape formed by this rotation is a cone. The leg 'a' becomes the height of the cone, and the leg 'b' becomes the radius of the base of the cone.
Hint: Recall the definition of a cone: it has a circular base and a pointed top (vertex).
Step 5: Conclusion
Thus, when a right triangle is rotated about one of its legs, the resulting three-dimensional figure is a cone.
Final Answer: The three-dimensional figure formed is a cone.
A right triangle consists of one right angle (90 degrees) and two other angles that add up to 90 degrees. The two legs of the triangle are perpendicular to each other.
When we rotate the right triangle, we need to choose one of its legs as the axis of rotation. Let's assume we rotate the triangle around one of the legs, say leg 'a'.
As we rotate the triangle around leg 'a', the other leg 'b' will sweep out a circular path. The rotation creates a three-dimensional shape.
The shape formed by this rotation is a cone. The leg 'a' becomes the height of the cone, and the leg 'b' becomes the radius of the base of the cone.
Thus, when a right triangle is rotated about one of its legs, the resulting three-dimensional figure is a cone.
Similar Questions
Knowledge Check
In the figure, ABC is a right triangle. What is the value of y?
 A20B30C50D90
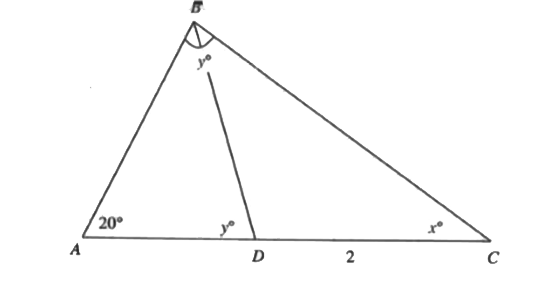
A20B30C50D90In the figure, triangle ABC and ABD are right triangle , what is the value of x?
 A20B30C50D70
A20B30C50D70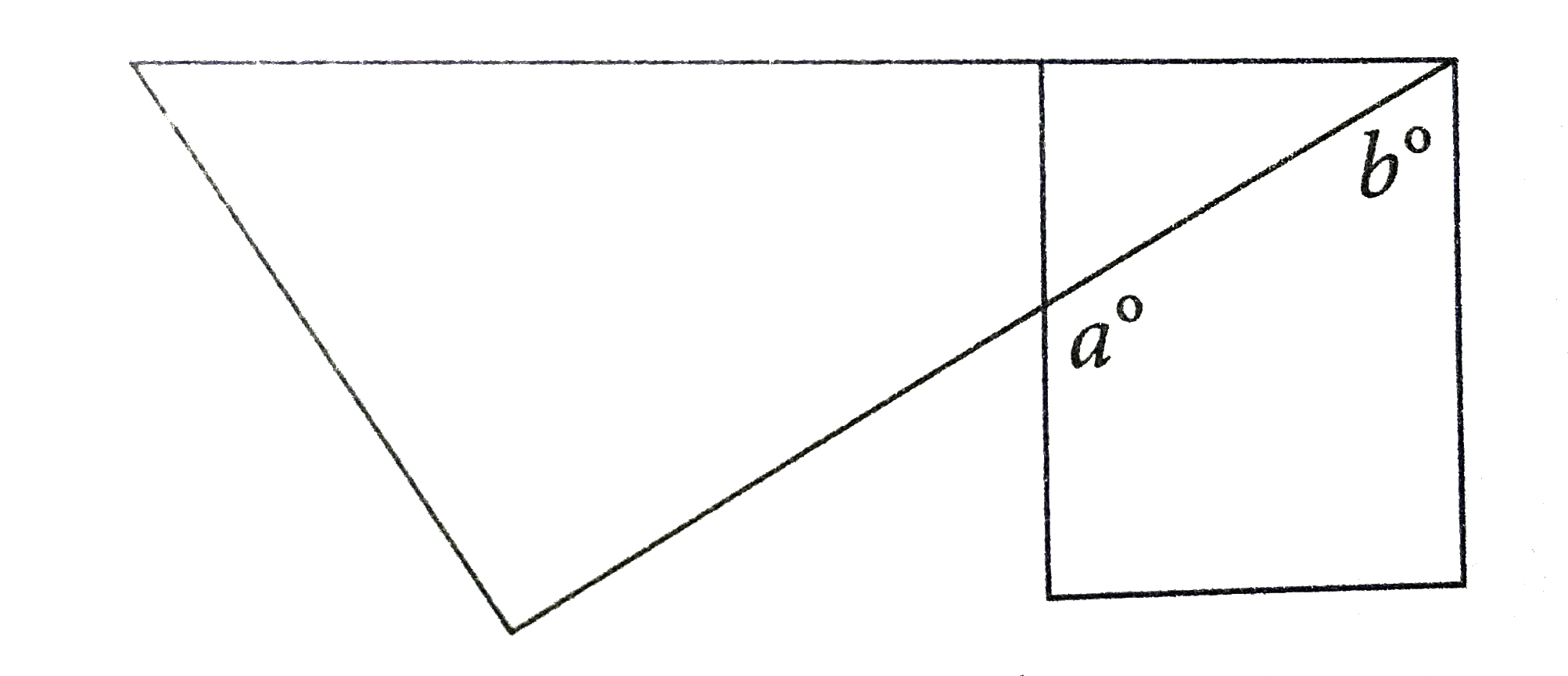
The figure above is formed by a triangle overlapping a rectangle. What is the value of a+b?A90∘B150∘C180∘D270∘
The volume of the greatest cone obtained by rotating a right - angled triangle of hypotenuse 2 units about a side is kπ9√3 cubic units, then the value of k is equal to
View Solutionx+y=7 and ax2+2hxy+ay2=0,(a≠0) , are three real distinct lines forming a triangle. Then the triangle is (a) isosceles (b) scalene equilateral (d) right angled
View SolutionIn figure ABC is an isosceles triangle , right angled at C . Therefore

View SolutionIn the adjoining figure , ΔABC is a right - angled triangle , right - angled at B. If ∠A=30∘andAC=8cm, Find (i)BC (ii)AB.

View SolutionWhich of the following are the dimensions of a right triangle ?
View Solution