Find the height a 17 foot ladder can reach on the side of a building when it hits the ground at a 65∘ angle.
7.2 ft.
15.4 ft.
36.5 ft.
48 ft.
The correct Answer is:B
To find the height that a 17-foot ladder can reach on the side of a building when it hits the ground at a 65-degree angle, we can use trigonometric functions. Here’s a step-by-step solution:
Step 1: Understand the Problem
The ladder forms a right triangle with the ground and the wall of the building. The length of the ladder is the hypotenuse of the triangle, and we need to find the height (the opposite side) when the ladder is positioned at a 65-degree angle with the ground.
Step 2: Identify the Relevant Trigonometric Function
In a right triangle, the sine function relates the opposite side (height) to the hypotenuse (length of the ladder). The formula is:
sin(θ)=oppositehypotenuse
Where:
- θ is the angle with the ground (65 degrees).
- The opposite side is the height we want to find.
- The hypotenuse is the length of the ladder (17 feet).
Step 3: Set Up the Equation
Using the sine function, we can express the height (h) as:
h=L⋅sin(65∘)
Where L is the length of the ladder (17 feet).
Step 4: Substitute the Values
Now, substitute the known values into the equation:
h=17⋅sin(65∘)
Step 5: Calculate sin(65∘)
Using a calculator, we find:
sin(65∘)≈0.9063
Step 6: Calculate the Height
Now, substitute sin(65∘) into the equation:
h=17⋅0.9063≈15.4 feet
Final Answer
The height that the ladder can reach on the side of the building is approximately 15.4 feet.
---
The ladder forms a right triangle with the ground and the wall of the building. The length of the ladder is the hypotenuse of the triangle, and we need to find the height (the opposite side) when the ladder is positioned at a 65-degree angle with the ground.
In a right triangle, the sine function relates the opposite side (height) to the hypotenuse (length of the ladder). The formula is:
sin(θ)=oppositehypotenuse
Where:
- θ is the angle with the ground (65 degrees).
- The opposite side is the height we want to find.
- The hypotenuse is the length of the ladder (17 feet).
Using the sine function, we can express the height (h) as:
h=L⋅sin(65∘)
Where L is the length of the ladder (17 feet).
Now, substitute the known values into the equation:
h=17⋅sin(65∘)
Using a calculator, we find:
sin(65∘)≈0.9063
Now, substitute sin(65∘) into the equation:
h=17⋅0.9063≈15.4 feet
The height that the ladder can reach on the side of the building is approximately 15.4 feet.
Similar Questions
A football is kicked with a velocity of 20 m/s at an angle of 450 with the horizontal. A. Find the time taken by the ball to strike the ground. find the maximum height it reaches. C. How far away from the kick downs it hit the ground? Take g=10ms2.
View SolutionA ladder 65 feet long is placed against a building and reaches a point that is 56 feet above the ground on one side of a street. Keeping the foot of the ladder at the same point, the ladder is turned to a building on the other side of the street. It now touches this building at a point 63 feet above the ground. find the width of the street.
View Solution
Knowledge Check
- Question 1 - Select One
A 25 foot ladder is placed against the side of a building at an angle of 70∘ from the ground. How far away is the base of the ladder from the building?
A25cos70∘B8.5sin70∘C25tan70∘D8.5cos70∘ - Question 2 - Select One
A painter needs to reach the top of a tail sign in the middle of a flat and level field .He uses a ladder of length x to reach a point on the sign 15 feet above the ground . The angle formed where the ladder meets the ground is noted in the figure below as θ , which of the following relation - ships must be true ?
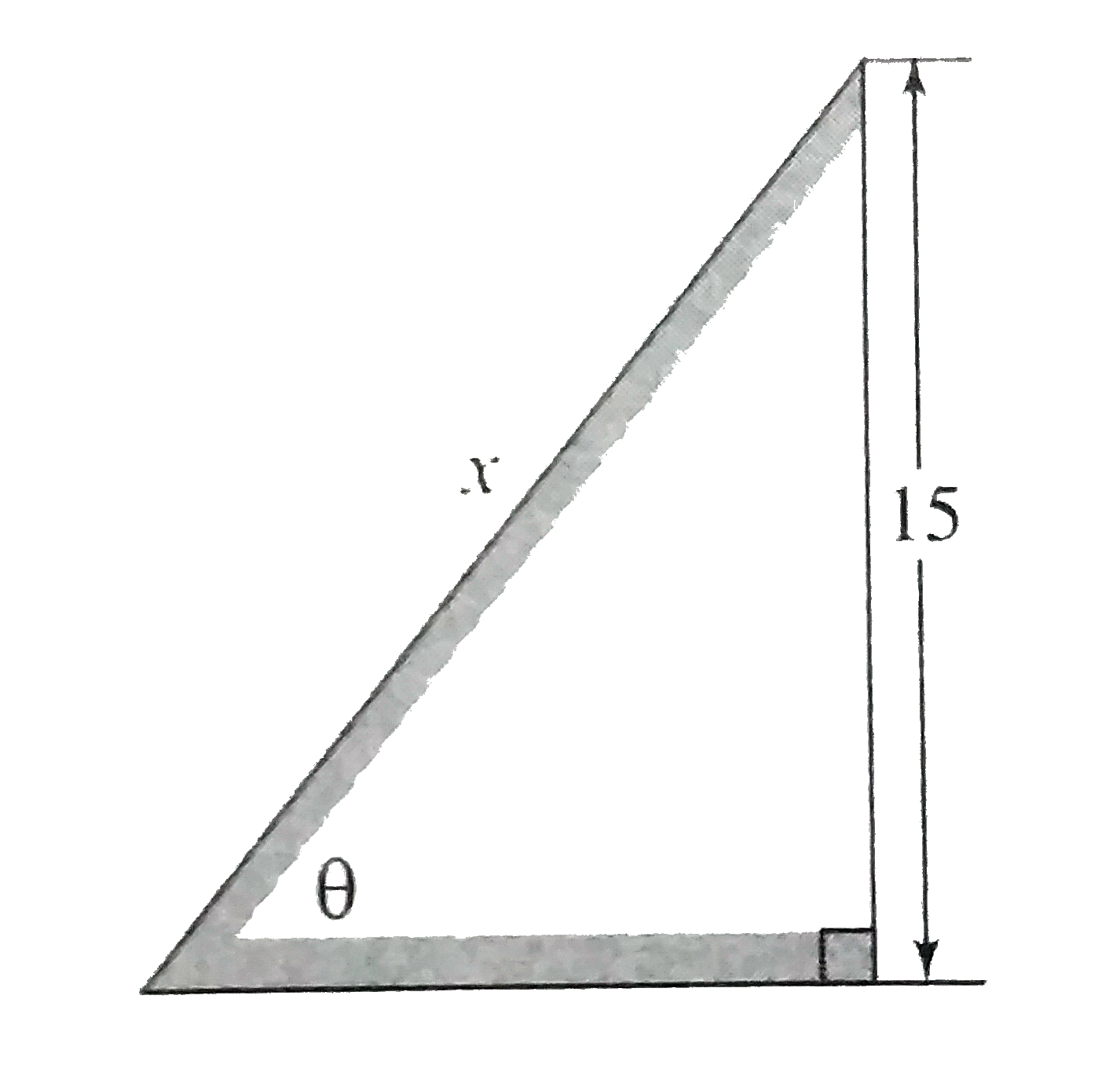 Asinθ=15xBcosθ=15xCtanθ=15xDθ=15x
Asinθ=15xBcosθ=15xCtanθ=15xDθ=15x
A stone is dropped from a height h . It hits the ground with a certain momentum P . If the same stone is dropped from a height 100% more thanthe preyiious height, the momentum when it hits the ground will change by
View SolutionFind the gravitational potential energy of 1 kg mass kept at a height of 5 m above the ground Calculate its kinetic energy when it falls and hits the ground. Take 10 ms−2
View SolutionA body is projected horizontally from the top of a tower with a velocity of 10m/s.If it hits the ground at an angle 45∘, then vertical component of velocity when it hits ground in m/s is
View SolutionA body is projected horizontally from the top of a tower with a velocity of 10m/s. If it hits the ground at an angle of 45∘, the vertical component of velocity when it hits ground in m/s is
View SolutionA bullet fired at an angle of 60∘ with the vertical hits the levelled ground at a distance of 200 m . Find the distance at which the bullet will hit the ground when fired at angle of 30∘. (with same speed).
View SolutionA stone is thrown downwards with velocity 10 m/s from the roof of a builng and it reaches the ground with speed 40 m/s. the height of the building would be
View Solution

