For torsional oscillator, √I/C has dimensions of ? Where C is torsional constant and I is moment of inertia :
M0L0T−1
M0L0T1
M0L1T−1
M0L0T2
The correct Answer is:B
Step by step video, text & image solution for For torsional oscillator, sqrt(I//C) has dimensions of ? Where C is torsional constant and I is moment of inertia : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A circular disc of mass 12 kg is suspended by a wire attached to its centre. The wire is twisted by rotating the disc and then released, so that the time period of torsional oscillation is 2 s. If the radius of the disc is 10 cm, then calculate the torsional spring constant of the wire.
View Solution(a) A uniform disc of radius 5⋅0cm and mass 200g is fixed at its centre to a metal wire, the other end of which is fixed with a clamp. The hanging disc is rotated about the wire through an angle and is released. If the disc makes torsional oscillations with time period 0.20s, find the torsional constant of the wire.
(b) In the previous part, another disc is placed over the first one and the time period of the system become 0.65. Find the M.I. of the second disc about the wire.View Solution
Knowledge Check
- Question 1 - Select One
The dimensions of gravitational constant G and the moment of inertia are, respectively
A[ML3T−2],[ML2T0]B[M−1L3T−2],[ML2T0]C[M−1L3T−2],[M−1L2T]D[ML3T−2],[M−1L2T] - Question 2 - Select One
S. I unit of moment of Inertia is
AKgm,BKgm−1CKgm−2DKgm2 - Question 3 - Select One
The ratio of the dimensions of plank's constant and that of the moment of inertia is the dimension of
AfrequencyBvelocityCangular momentionDtime
The pulley shown in figure has a moment of inertias I about its xis and mss m. find the tikme period of vertical oscillastion of its centre of mass. The spring has spring constant k and the string does not slip over the pulley.
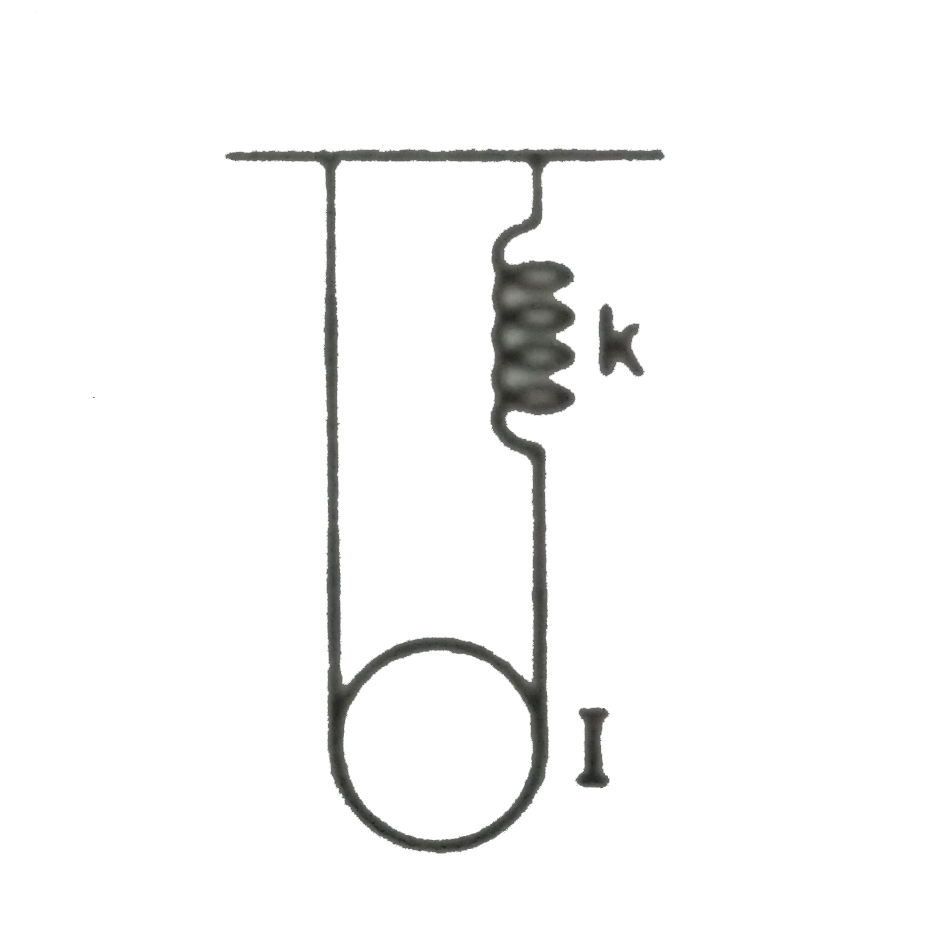View SolutionFind the period of small torsional oscillational of a system consisting of two discs slipped oon a thin rod with rod with torsional coefficient k. The moments of inertia of discs relative to the rod's axis are equal to I1 and I2.
View SolutionThe ratio of the dimension of Planck's constant and that of moment of inertia is the dimension of
View SolutionThe ratio of the dimensions of Planck's constant and that of moment of inertia has the dimensions of
View SolutionWhich one of the following statements concerning the moment of inertia I is false?
View Solution