A particle is projected up a 45∘ rough incline with a velocity v. The coeffciient of friciton is 0.5. The speed with which it returns back to the starting point is v'. Then v' /v is :
√2/√3
1/√3
1/2
1/√2
The correct Answer is:2
To solve the problem, we will analyze the motion of the particle projected up a rough incline and use the work-energy theorem to find the relationship between the initial velocity v and the final velocity v′ when it returns to the starting point.
1. Identify the Forces Acting on the Particle:
- When the particle is projected up the incline, it experiences two main forces:
- The gravitational force acting downwards, which can be resolved into two components: one parallel to the incline mgsin(45∘) and one perpendicular to the incline mgcos(45∘).
- The frictional force acting down the incline, which is given by f=μN=μmgcos(45∘), where μ=0.5.
2. Calculate the Work Done Against Gravity and Friction:
- The work done against gravity when the particle moves a distance S up the incline is:
Wg=−mgsin(45∘)⋅S=−mg⋅1√2⋅S
- The work done against friction is:
Wf=−f⋅S=−μmgcos(45∘)⋅S=−0.5⋅mg⋅1√2⋅S
3. Apply the Work-Energy Theorem:
- The work-energy theorem states that the change in kinetic energy is equal to the work done by all forces:
ΔKE=Wg+Wf
- The initial kinetic energy is 12mv2 and the final kinetic energy at the highest point (where the particle momentarily stops) is 0. Thus:
0−12mv2=−mg⋅1√2⋅S−0.5⋅mg⋅1√2⋅S
- Simplifying gives:
−12mv2=−mg⋅32√2⋅S
- Canceling m and rearranging gives:
S=v2√23g
4. Calculate the Work Done When Returning:
- When the particle returns, the work done by gravity is zero (as it moves back to the same height), but the work done by friction will act against the motion:
W′f=−2⋅(0.5⋅mg⋅1√2⋅S)=−mg⋅1√2⋅S
- The change in kinetic energy when returning is:
12mv′2−0=−mg⋅1√2⋅S
5. Substituting the Value of S:
- Substitute S from step 3 into the work done equation:
12mv′2=mg⋅1√2⋅v2√23g
- Simplifying gives:
12mv′2=13mv2
- Canceling m from both sides leads to:
v′2=23v2
6. Finding the Ratio v′v:
- Taking the square root gives:
v′v=√23=v′v=1√3
Final Answer:
The ratio v′v is 1√3.
- When the particle is projected up the incline, it experiences two main forces:
- The gravitational force acting downwards, which can be resolved into two components: one parallel to the incline mgsin(45∘) and one perpendicular to the incline mgcos(45∘).
- The frictional force acting down the incline, which is given by f=μN=μmgcos(45∘), where μ=0.5.
- The work done against gravity when the particle moves a distance S up the incline is:
Wg=−mgsin(45∘)⋅S=−mg⋅1√2⋅S
- The work done against friction is:
Wf=−f⋅S=−μmgcos(45∘)⋅S=−0.5⋅mg⋅1√2⋅S
- The work-energy theorem states that the change in kinetic energy is equal to the work done by all forces:
ΔKE=Wg+Wf
- The initial kinetic energy is 12mv2 and the final kinetic energy at the highest point (where the particle momentarily stops) is 0. Thus:
0−12mv2=−mg⋅1√2⋅S−0.5⋅mg⋅1√2⋅S
- Simplifying gives:
−12mv2=−mg⋅32√2⋅S
- Canceling m and rearranging gives:
S=v2√23g
- When the particle returns, the work done by gravity is zero (as it moves back to the same height), but the work done by friction will act against the motion:
W′f=−2⋅(0.5⋅mg⋅1√2⋅S)=−mg⋅1√2⋅S
- The change in kinetic energy when returning is:
12mv′2−0=−mg⋅1√2⋅S
- Substitute S from step 3 into the work done equation:
12mv′2=mg⋅1√2⋅v2√23g
- Simplifying gives:
12mv′2=13mv2
- Canceling m from both sides leads to:
v′2=23v2
- Taking the square root gives:
v′v=√23=v′v=1√3
The ratio v′v is 1√3.
Similar Questions
A particle is projected vertically upwards with a velocity v. It returns to the ground in time T. Which of the following graphs correctly represents the motion.
View SolutionThe fig. shows the v−t graph of a particle moving in straight line. Find the time when particle returns to the starting point.
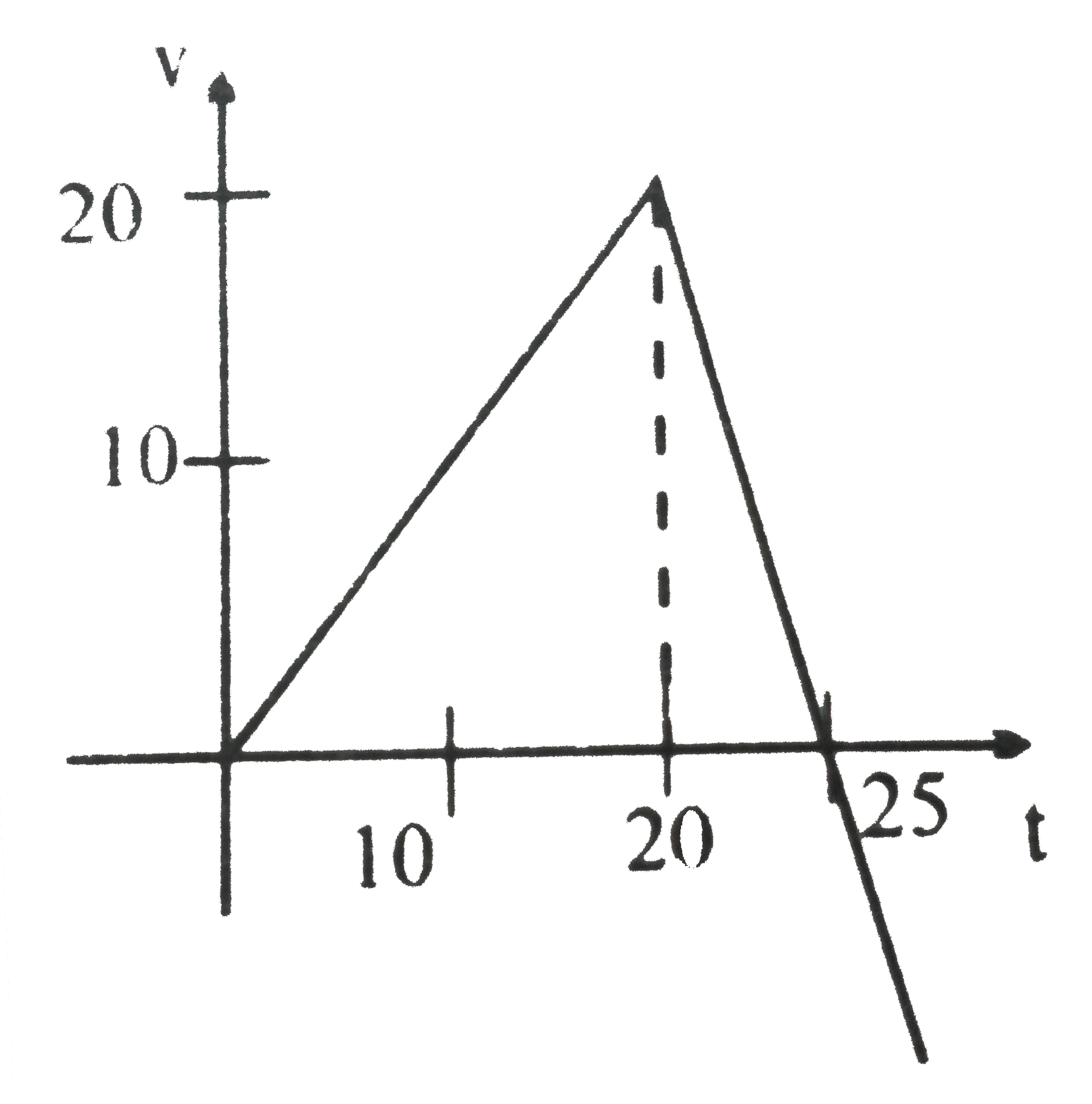
View Solution
Knowledge Check
A particle is projected up a 37∘ rough incline with velocity v0. If μ=1/2, the speed with which it returns back to the starting point is v. Then v/v0 is
A12B1√3C1√5D1√2A body is projected up along a rough inclined plane of inclination 45∘ . The coefficient of friction is 0.5. Then the retardation of the block is
Ag2√2Bg2C3g2√2Dg√2A particle is projected directly along a rough plane of inclination θ with velocity u. If after coming to the rest the particle returns to the starting point with velocity v, the coefficient of friction between the partice and the plane is
Au2v2tanθBu2−v2u2+v2tanθCv2u2tanθDu2+v2u2−v2tanθ
If a charged particle is projected on a rough horizontal surface with speed v0. Find the value of dynamic coefficient of friction, if the kinetic energy of system is constant.
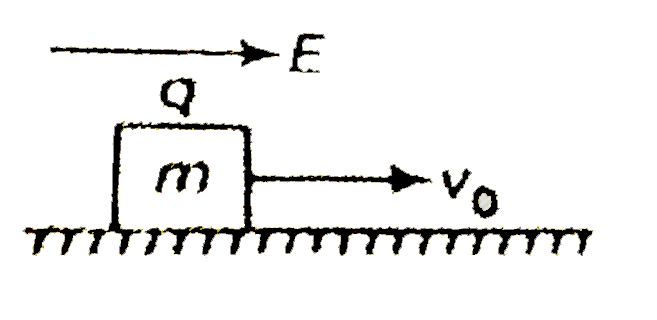
View SolutionA particle of mass m is projected upword with velocity v=ve2 (ve) escape of the particle is
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. At any instant its velocity becomes v which is perpendicular to the initial velocity u. Then v is
View SolutionA body is projected up along the rough inclined plane from the bottom with some velocity. It travels up the incline and then returns back. If the time of ascent is ta and time of descent is td then
View SolutionA particle is projected with velocity V for point O of particle makes 10 revolutions before coming out. Find l.
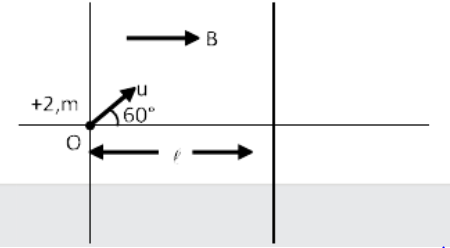
View Solution
