The mid points of the sides of a triangle are (5,7,11),(0,8,5)and(2,3,−1)Find its vertices and hence find centroid.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

Let vertices of the ΔABC are A(x1,y1,z1),B(x2,y2,z3)andC(x3,y3,z3) then the mid-point of BC ( 5,7,11)
5=x2+x32⇒x2+x3=10
7=y2+y32⇒y2+y3=14
11=z2+z32⇒z2+z3=22
Similarly for the sides AB and AC.
2=x1+x22⇒x1=x2=4
3=y1+y22⇒y1=y2=6
−1=z1+z22⇒z1=z2=−2
0=x1+x32⇒x1+x3=0
8=y1+y32⇒y1+y3=16
5=z1+z32⇒z1+z3=10
Form Eqs. (i) and (iv)
x1+2x2+x2=14
from Eqs. (ii) and (vi)
y1+2y2+y3=20
from Eqs. (iii) and (vi)
z1+2z2+z3+20
from Eqs. (vii) and (x)
2x2=14⇒x2=7
x2=7,then x3=10−7=3
x3=3,thenx1=−3
x1=−3,x2=7,x3=12
from Eqs. (viii) and (xi),
2y2=4⇒y2=5
y2=2,theny1=4
y(1)=4,theny3=12
y1=4,y2=2,y3=12
From Eqs. (ix) and (xii)
2z2=10⇒z2=5
z2=5, thenz1=−7
z1=−7,thenz3=17
z1=−7,z2=5,z3=17
So, the vertices are A (-3,4,-7) B (7,2,5) and C ( 3,12,17)
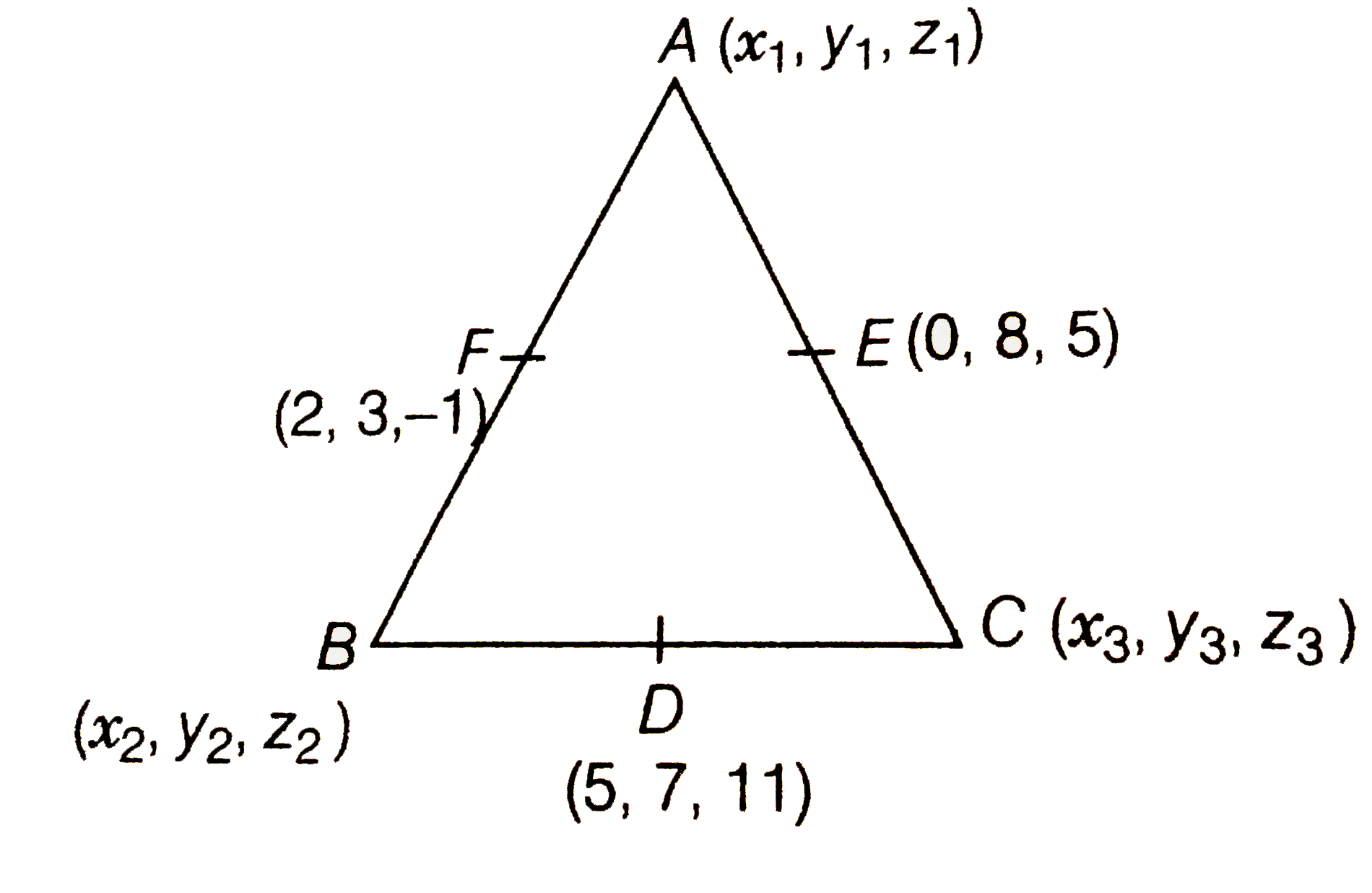
ocr_image

Updated on:21/07/2023
|
Class 11MATHSINTRODUCTION TO THREE DIMENSIONAL GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • It the mid-points of the sides of triangle are (1, 2, -3),
    (3, 0, 1) and (2, -2, 5), then the centroid is

    A(0, 0, 0)
    B(1, 2, 3)
    C(2, 0, 1)
    D(2, 3, 0)