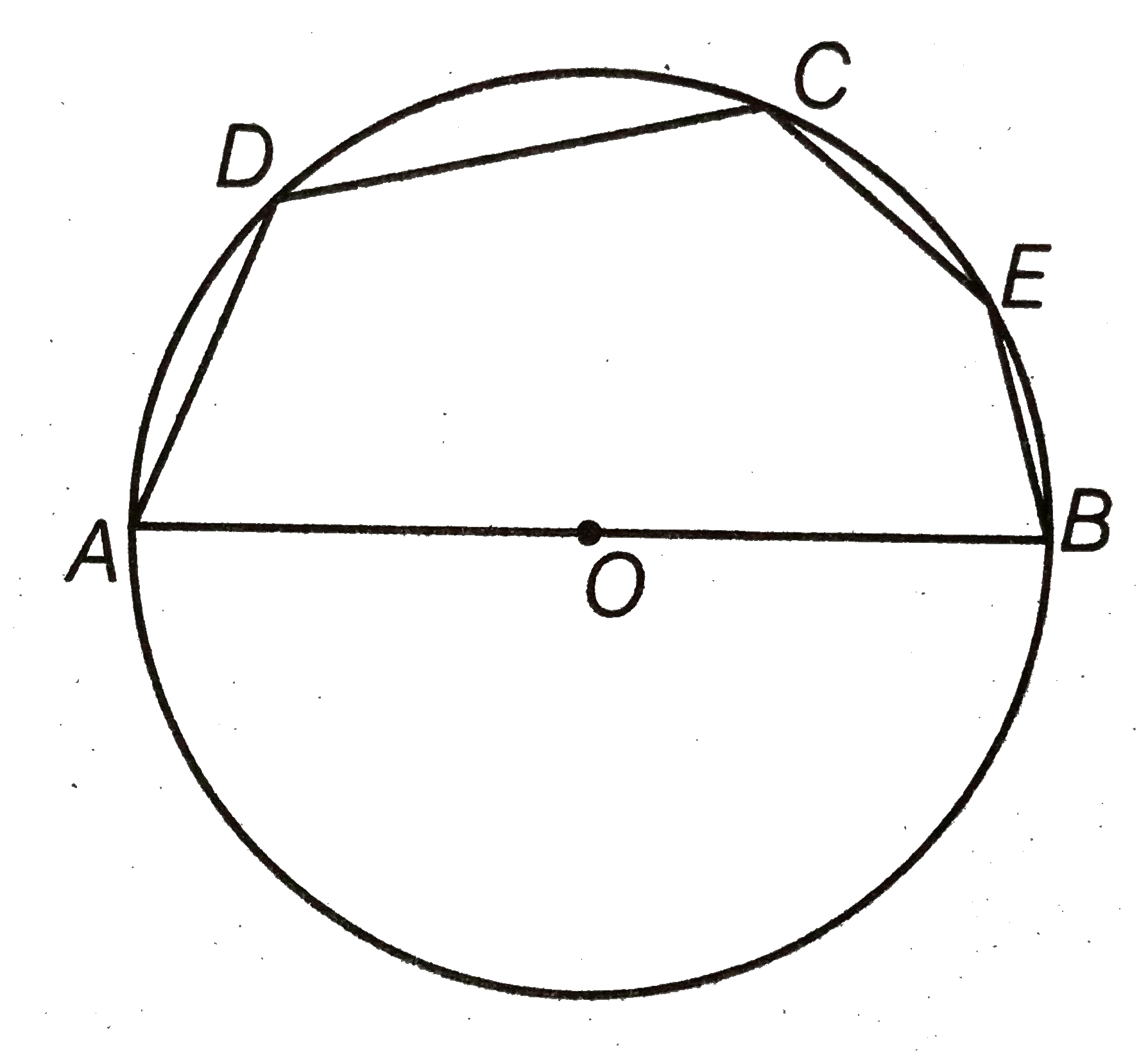
In figure, BC is a diameter of the circle and ∠BAO=60∘. Then,∠ADCis equal to
A
60∘
B
45∘
C
50∘
D
120∘
Video Solution
Text Solution
The correct Answer is:A
|
Answer
Step by step video, text & image solution for In figure, BC is a diameter of the circle and angleBAO=60^(@)." Then", angleADCis equal to by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Exercise 10.42videos
CIRCLES
- BOOK - NCERT EXEMPLARCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Areas Of Parallelograms And Triangles34videos
Areas of Parallelograms and Triangles
- BOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions5videos
CONSTRUCTIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC=140∘, then ∠BAC is equal to
A80∘B50∘C40∘D30∘ - Question 2 - Select One
In the adjoining figure, BP is the diameter of the circle. If ∠ABP=60∘, then find ∠AQB.
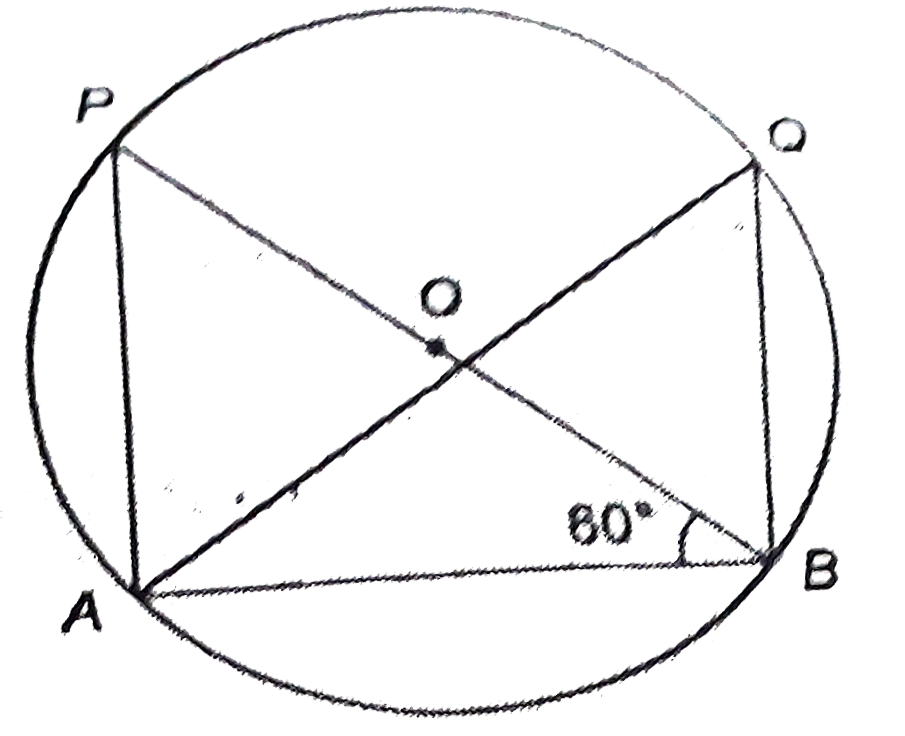 A60∘B45∘C30∘D50∘
A60∘B45∘C30∘D50∘ - Question 3 - Select One
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC=140∘, than ∠BAC is equal to
A80∘B50∘C40∘D30∘
In the given figure, AB is the diameter of the circle, ∠ADC=120∘. Find ∠CAB:
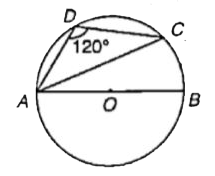 View Solution
View SolutionIf AOB is a diameter of the circle and AC=BC, then ∠CAB is equal to
View SolutionIn the given figure, O is the centre of the circle and ∠AOC=100∘. Find the ratio of ∠ADC:∠ABC :
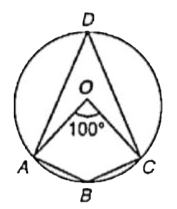 View Solution
View SolutionIn the given figure, PAQ is the tangent. BC is the diameter of the circle. ∠BAQ=60∘, find ∠ABC :
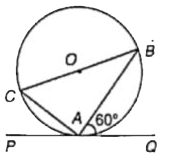 View Solution
View SolutionIn the given figure, BOD is the diameter of the circle with centre O. ∠COD=92∘and∠ABD=65∘. Then y equals
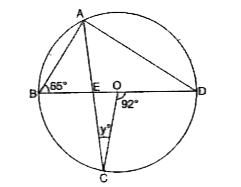 View Solution
View Solution