If BM and CN are the perpendiculars drawn on the sides AC and BC of the ΔABC, prove that the points B, C, M and N are concyclic.
Given In ΔABC,BM⊥ACandCN⊥AB.
To prove Points B, C, M and N are con-cyclic.
Construction Draw a circle passing through the points B, C, M and N.
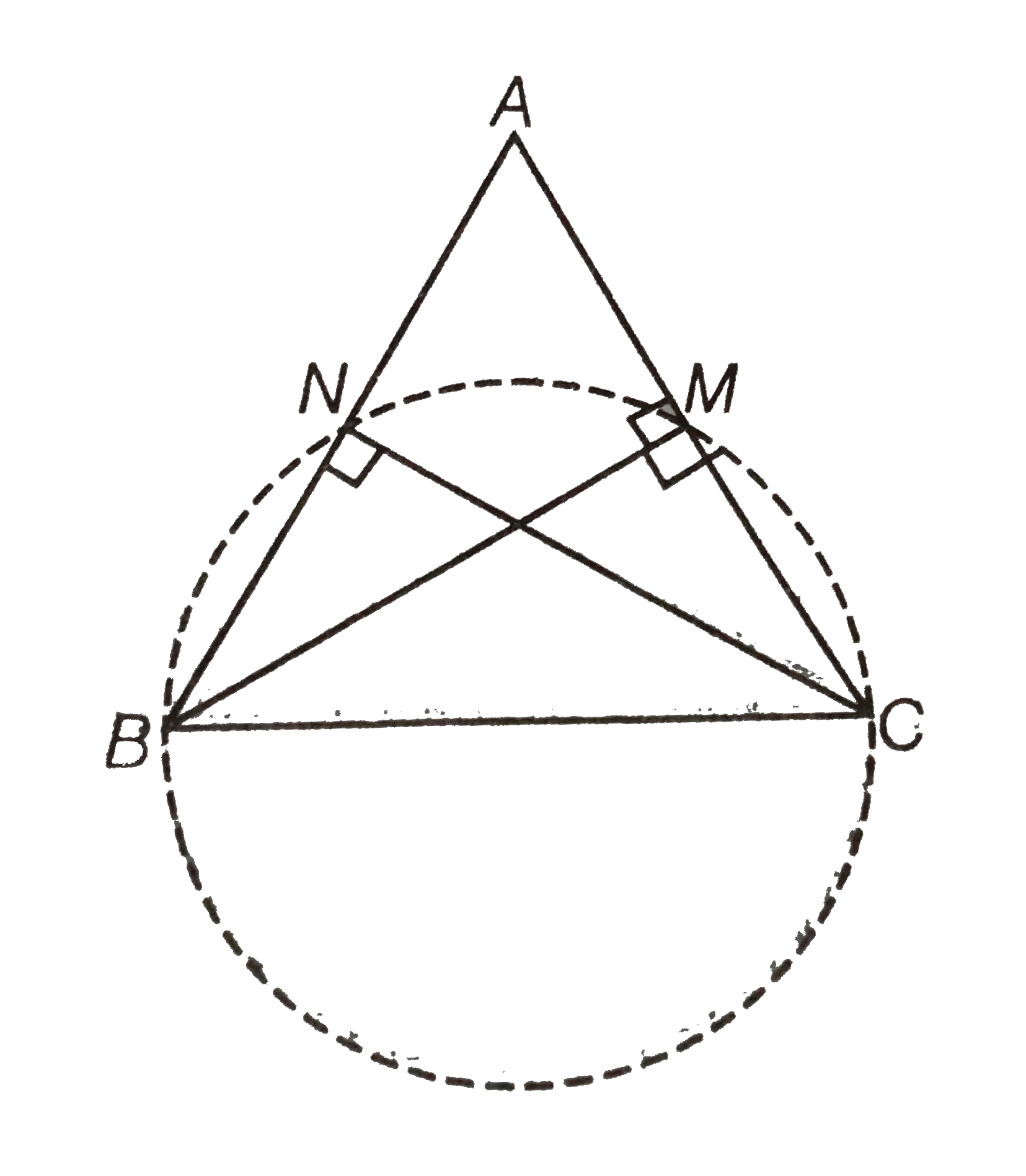
Proof Suppose, we consider BC as a diameter of the circle. Also, we know that BC subtends a 90∘ to the circle.
So, the points M and N should be on a circle.
Hence, BCMN form a con-cyclic quadrilateral.
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Exercise 10.4
2 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLARCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Areas Of Parallelograms And Triangles
34 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
In Figure, ABC is a triangle in which AB=AC. Point DandE are points onthe sides ABandAC respectively such that AD=AE. Show that the points B,C,EandD are concyclic.
View SolutionABC is a triangle is which BE and CF are, respectively, the perpendiculars to the sides AC and AB . If BE=CF , prove that ABC is isosceles.
View Solution
Knowledge Check
In ΔABC,ADandBE are perpendiculars from A and B to the sides BC and AC, then
ACA. CE = CB. CDBCB. CA = CE. CDCADBE=ABDEDNone of theseAL, BM and CN are perpendicular from angular points of a triangle ABC on the opposite sides BC, CA and AB respectively. Δ is the area of triangle ABC, (r ) and R are the inradius and circumradius.
If radis of the incircle of ΔLMNisr', then the valur of r' sec A sec B sec C isA4RB3RC2RDRAL, BM and CN are perpendicular from angular points of a triangle ABC on the opposite sides BC, CA and AB respectively. Δ is the area of triangle ABC, (r ) and R are the inradius and circumradius.
If perimeters of ΔLMNandΔABCanλandμ, then the value of λμ isArRBRrCrRΔDΔrR
ABC is an isosceles triangle in which AB=AC. If D and E are the mid-points of AB and AC respectively, prove that the points B, C, D and E are concyclic.
View SolutionFrom the point P(5,0) perpendiculars PL, PM and PN are drawn to the sides AB, BC and CA of triangle ΔABC respectively, where L, M and N are the feet of the perpendiculars. If A(4,3),B(−4,3)andC(0,−5), which of the following is/are true?
View SolutionBM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If Lis the mid-point of BC, prove that LM = LN.
View SolutionAL, BM and CN are perpendicular from angular points of a triangle ABC on the opposite sides BC, CA and AB respectively. Δ is the area of triangle ABC, (r ) and R are the inradius and circumradius.
If area of ΔLMNisΔ', then the value of Δ'Δ is
View SolutionAL, BM and CN are perpendicular from angular points of a triangle ABC on the opposite sides BC, CA and AB respectively. Δ is the area of triangle ABC, (r ) and R are the inradius and circumradius.
Radius os the circum circle of ΔLMN is
View Solution
