Find the area of a parallelogram given in the figure. Also, find the length of the altitude from vertex A on the side DC.
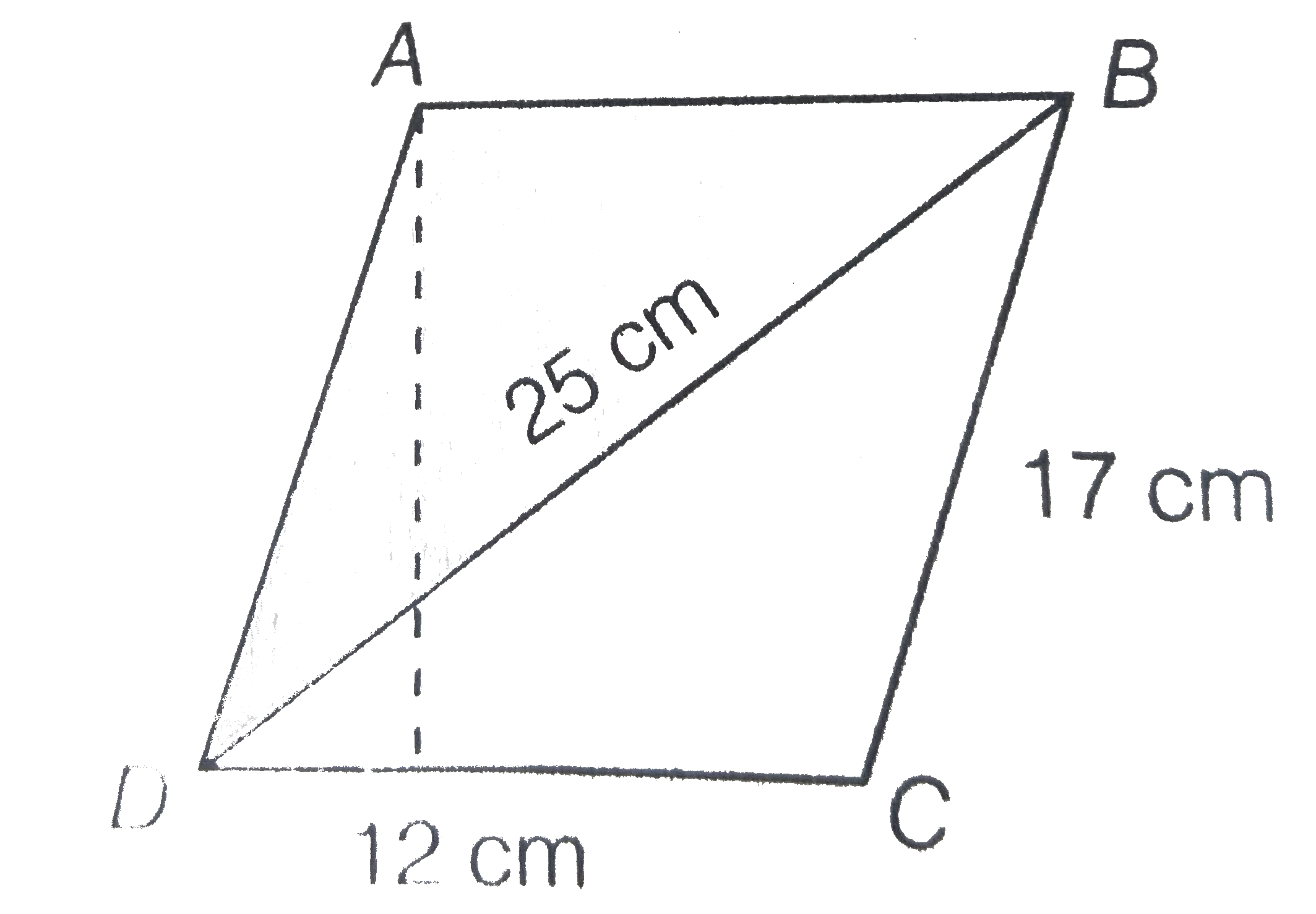
Area of parallelogram ABCD=2(Area of △BCD)
Now, the sides of a △BCD are a=12cm,b=17cm and c=25cm.
∴Semi-perimeter of △BCD,s=a+b+c2=12+17+252=542=27cm
∴Area of △BCD=√s(s−a)(s−b)(s−c) [by Heron's formula]
=√27(27−12)(27−17)(27−25)
=√27×15×10×2
=√9×3×3×5×5×2×2
=3×3×5×2=90cm2
From Eq.(i),
Area of parallelogram ABCD=2×90
=180cm2
Let altitude of parallelogram be h.
Also, area of parallelogram ABCD=Base×A<itude
⇒ 180=Dc×h
⇒ 180=12×h
∴ h=18012=15cm
Hence, the area of parallelogram is 180 cm2 and the length of altitude is 15cm.
Topper's Solved these Questions
Similar Questions
Area of a parallelogram = Base x Altitude
View SolutionFind the area of a parallelogram with base 8 cm and altitude 4.5 cm.
View SolutionFind the area of a parallelogram with base 5 cm and altitude 4.2 cm.
View SolutionFind the area of a parallelogram with base 32 cm and altitude 16.5 cm.
View SolutionIf the area of a parallelogram ABCD is 30cm2,then the length of altitude AQ is
View SolutionFind the length of the altitude of an equilateral triangle with side 6 cm.
View SolutionFind the length of the altitude of an equilateral triangle with side 6 cm.
View SolutionFind the length of the altitude of an equilateral triangle of side 2a cm.
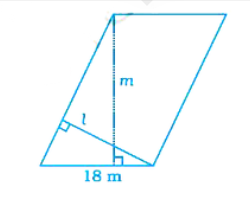
View SolutionPerimeter of a parallelogram shaped land is 96 m and its area is 270 square metres। If one of the sides of this parallelogram is 18 m, find the length of the other side। Also, find the lengths of altitudes 1 and m (Fig. 9.42).
 View Solution
View SolutionIf the sides of a triangle are 17,25and28, then find the greatest length of the altitude.
View Solution

