The moment of inertia of a solid flywheel about its axis is .A tangential force of 2 kg-wt. is applied round the circumference of the flywheel with the help of a string and mass arrangement as shown in the figure. If the radius of the wheel is 0.1 m, find the acceleration of the mass :
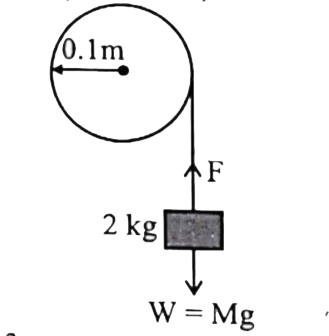
163.3
16.3
81.66
8.16
The correct Answer is:B
Topper's Solved these Questions
Similar Questions
The moment of inertia of a flywheel is 4kgm2. What angular acceleration will be produced in it by applying a torque of 10 Nm on it?
View SolutionThe rotational inertia of a disc about its axis is 0.70 kg m2. When a 2.0 kg mass is added to its rim, 0.40 m from the axis, the rotational inertia becomes
View Solution
Knowledge Check
If moment of inertia of a solid sphere of mass 5kg about its diameter is 50kgm2 . Its moment of inertia about its tangent in kg m2 is
A260B250C240D175The moment of inertia of a disc of radius 0.5m about its geometric axis is 2kg-m2 . If a string is tied to its circumference and a force of 10 Newton is applied, the value of torque with respect to this axis will be:
A2.5 N-mB5 N-mC10 N-mD20 N-mthe angular acceleration of flywheel having moment of inertia 50 kg m2 when a torque of 5 N−m is applied on the flywheel is
A20.1rads−2B250rads−2C10rads−2D1.25rads−2
Calculate the moment of inertia of a solid sphere of mass 10 kg and radius 0.5 m, rotating about an axis 0.2 m from the centre of the sphere .
View SolutionThree identical solid discs, each of mass M and radius R, are arranged as shown in figure. The moment of inertia of the system about an axis AB will be
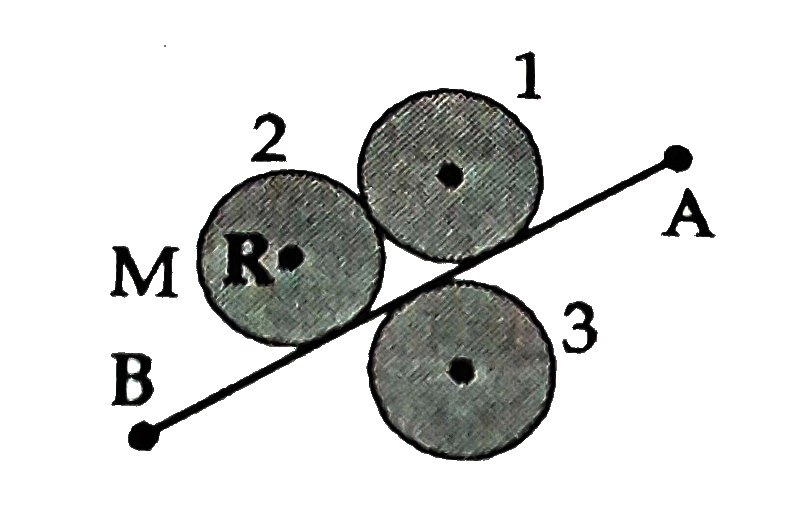
View Solutionthe moment of inertia of a uniform rod of mass 0.50 kg and length 1 m is 0.10 kg m^2 about a line perpendicular to the rod. Find the distance of this line from the middle point of the rod.
View SolutionThe moment of inertia of a disc of mass M and radius R about an axis. Which is tangential to sircumference of disc and parallel to its diameter is.
View SolutionFind the moment of inertia of a hemisphere of mass M and radius R shown in the figure, about an axis AA' tangential to the hemisphere.
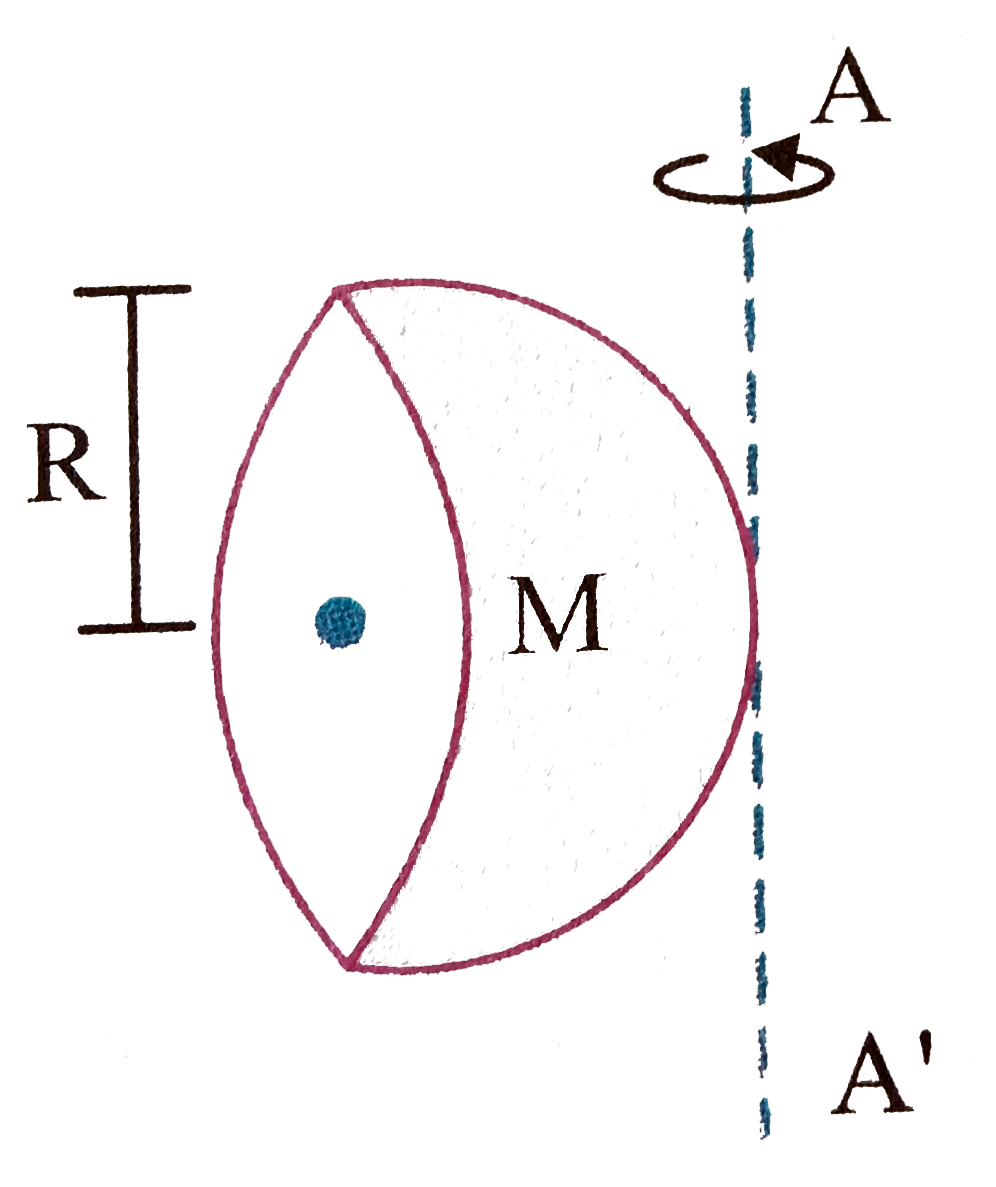
View Solution
