If the distance between H and Cl ions in HCl molccule is x, then its moment of incrtia about an axis passing through the centre of mass and perpendicular to the bond length, is-
35x2
36x2/35
35x2/36
x2/35
The correct Answer is:C
I=m1m2m1+m2.x2
I=mHmClmH+mCl.x2
I=35x236
Topper's Solved these Questions
Similar Questions
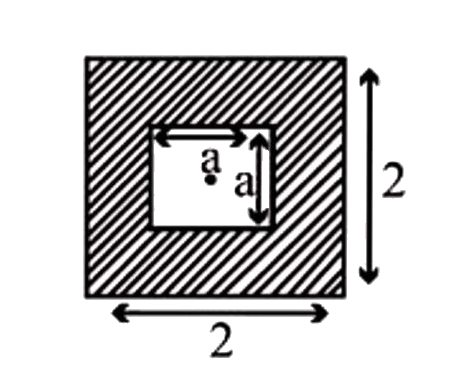
A square of a side a is cut from a square of side 2a as shown in the figure. Mass of this square with a hole is M. Then its moment of inertia about an axis passing through its centre of mass and perpendicular to its plane will be
View SolutionFor the same total mass, which of the following will have the largest moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the body
View Solution
Knowledge Check
The radius of gyration of an uniform rod of length L about an axis passing through its centre of mass and perpendicular to its length is.
AL√2BL2√12CL√3DL√2A uniform thin bar of mass 6m and length 12L is bent to make a regular hexagon. Its moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of hexagon is
A20mL2B30mL2C(125)mL2D6mL2A uniform thin bar of mass 6m and length 12L is bend to make a regular hexagon. Its moment of inertia about an axis passing through the centre of mass and perpendicular to the plane of the hexagon is :
A20mL2B6mL2C125mL2D30mL2
A solid cylinder has mass M radius R and length / its moment of inertia about an axis passing through its centre and perpendicular to its own axis is
View SolutionA solid cylinder has mass M radius R and length / its moment of inertia about an axis passing through its centre and perpendicular to its own axis is
View SolutionFor the same total mass which of the following will have the largest moment of inertia about an axis passing through its centre of mass and perpendicular to the plane of the body.
View SolutionThe moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View Solution