A hollow sphere of charge does not produce an electric field at any-
Interior point
Outer point
Surface point
None of the above
The correct Answer is:A
To solve the question regarding the electric field produced by a hollow sphere of charge, we can follow these steps:
1. Understanding the Problem: We need to determine at which point (interior, surface, or exterior) a hollow sphere of charge does not produce an electric field.
2. Applying Gauss's Law: Gauss's law states that the electric flux through a closed surface is proportional to the charge enclosed by that surface. Mathematically, it is given by:
ΦE=∮→E⋅d→A=Qencϵ0
3. Analyzing the Interior Point:
- Consider a point inside the hollow sphere (interior point).
- For a Gaussian surface inside the hollow sphere, the enclosed charge Qenc=0 (since all the charge resides on the surface).
- Therefore, by Gauss's law:
∮→E⋅d→A=0⟹E=0(inside the hollow sphere)
4. Analyzing the Surface Point:
- Now consider a point on the surface of the hollow sphere.
- For a Gaussian surface that coincides with the surface of the hollow sphere, the enclosed charge Qenc=Q (the total charge on the surface).
- Applying Gauss's law:
∮→E⋅d→A=Qϵ0
- This leads to a non-zero electric field at the surface, calculated as:
E_{\text{surface}} = \frac{Q}{4\pi \epsilon_0 R^2} \quad \text{(where R is the radius of the sphere)}
5. Analyzing the Exterior Point:
- Now consider a point outside the hollow sphere.
- For a Gaussian surface outside the hollow sphere, the enclosed charge Qenc=Q.
- Again applying Gauss's law:
∮→E⋅d→A=Qϵ0
- This results in a non-zero electric field outside the sphere:
E_{\text{outside}} = \frac{Q}{4\pi \epsilon_0 r^2} \quad \text{(where r is the distance from the center)}
6. Conclusion: The electric field is zero only at interior points of the hollow sphere. At the surface and outside the sphere, the electric field is non-zero.
Final Answer:
The correct option is interior point.
ΦE=∮→E⋅d→A=Qencϵ0
- Consider a point inside the hollow sphere (interior point).
- For a Gaussian surface inside the hollow sphere, the enclosed charge Qenc=0 (since all the charge resides on the surface).
- Therefore, by Gauss's law:
∮→E⋅d→A=0⟹E=0(inside the hollow sphere)
- Now consider a point on the surface of the hollow sphere.
- For a Gaussian surface that coincides with the surface of the hollow sphere, the enclosed charge Qenc=Q (the total charge on the surface).
- Applying Gauss's law:
∮→E⋅d→A=Qϵ0
- This leads to a non-zero electric field at the surface, calculated as:
E_{\text{surface}} = \frac{Q}{4\pi \epsilon_0 R^2} \quad \text{(where R is the radius of the sphere)}
- Now consider a point outside the hollow sphere.
- For a Gaussian surface outside the hollow sphere, the enclosed charge Qenc=Q.
- Again applying Gauss's law:
∮→E⋅d→A=Qϵ0
- This results in a non-zero electric field outside the sphere:
E_{\text{outside}} = \frac{Q}{4\pi \epsilon_0 r^2} \quad \text{(where r is the distance from the center)}
The correct option is interior point.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - Exercise - 2 (Objective Problems | NEET)
64 Videos
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - Exercise -3 Section (A)
41 Videos
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - SELF PRACTICE PROBLEM
27 Videos
- ELECTRONICS - SEMI CONDUCTORBOOK - MOTIONCHAPTER - ELECTRONICS - SEMI CONDUCTOREXERCISE - EXERCISE - 3
29 Videos
- ELECTROSTATICS - I BOOK - MOTIONCHAPTER - ELECTROSTATICS - I EXERCISE - EXERCISE - 4 (Level -II) PREVIOUS YEAR - JEE ADVANCED
13 Videos
Similar Questions
Knowledge Check
If the potential at the centre of a uniformly charged hollow sphere of radus R is V, then electric field at a distance r from the centre of sphere will be (r>R).
 AVRr2BVrRCVRrDVRR2+r2
AVRr2BVrRCVRrDVRR2+r2The charges given to a hollow sphere of radius 10 cm is 10 n C . Electric field at a point situated at a distance of 4 cm from its centre is
A2250NC−1B56250NC−1C9000NC−1DzeroA hollow metal ball carrying an electric charge produces no electric field at points
Aoutside the sphereBon its surfaceCinside the sphereDonly at the centre
As the electric change on the surface of a hollow metal sphere increases, the electric field intensity inside the sphere
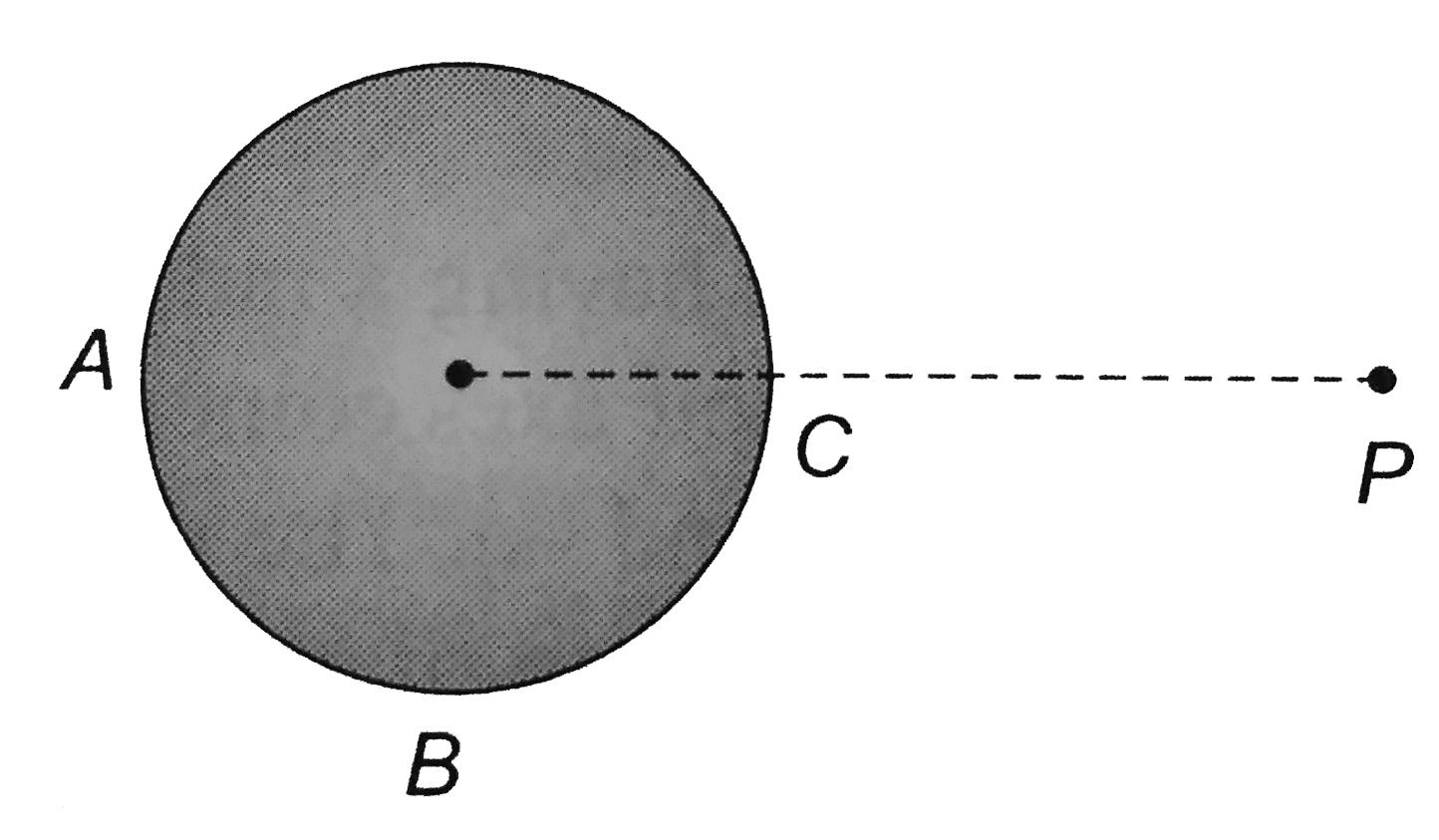
View SolutionA hollow conducting sphere is placed in an electric field produced by a point charge placed at P as shown in figure.
Let VA,VB,VC be the potentials at points A,BandC respectively. Then
View SolutionSTATEMENT -1 : Induced charge does not contribute to electric field or potential at a give point .
STATEMENT -2 : A point cahrge q0 is kept outside a solid metallic sphere , the electric field inside the sphere is zero.
View SolutionTwo isolated charged conducting spheres of radii a and b produce the same electric field near their surface. The ratio of electric potentials on their surfaces is
View SolutionIn a conducting hollow sphere of inner and outer radii 5 cm and 10 cm, respectively, a point charge 1 C is placed at point A, that is 3 cm from the center C of the hollow sphere. An external uniform electric field to magnitude 20NC−1 is also applied. Net electric force on this charge is 15 N, away from the center of the sphere as shown. Find the magnitude of net force exerted on the charge placed at point A by the induced charges on the sphere
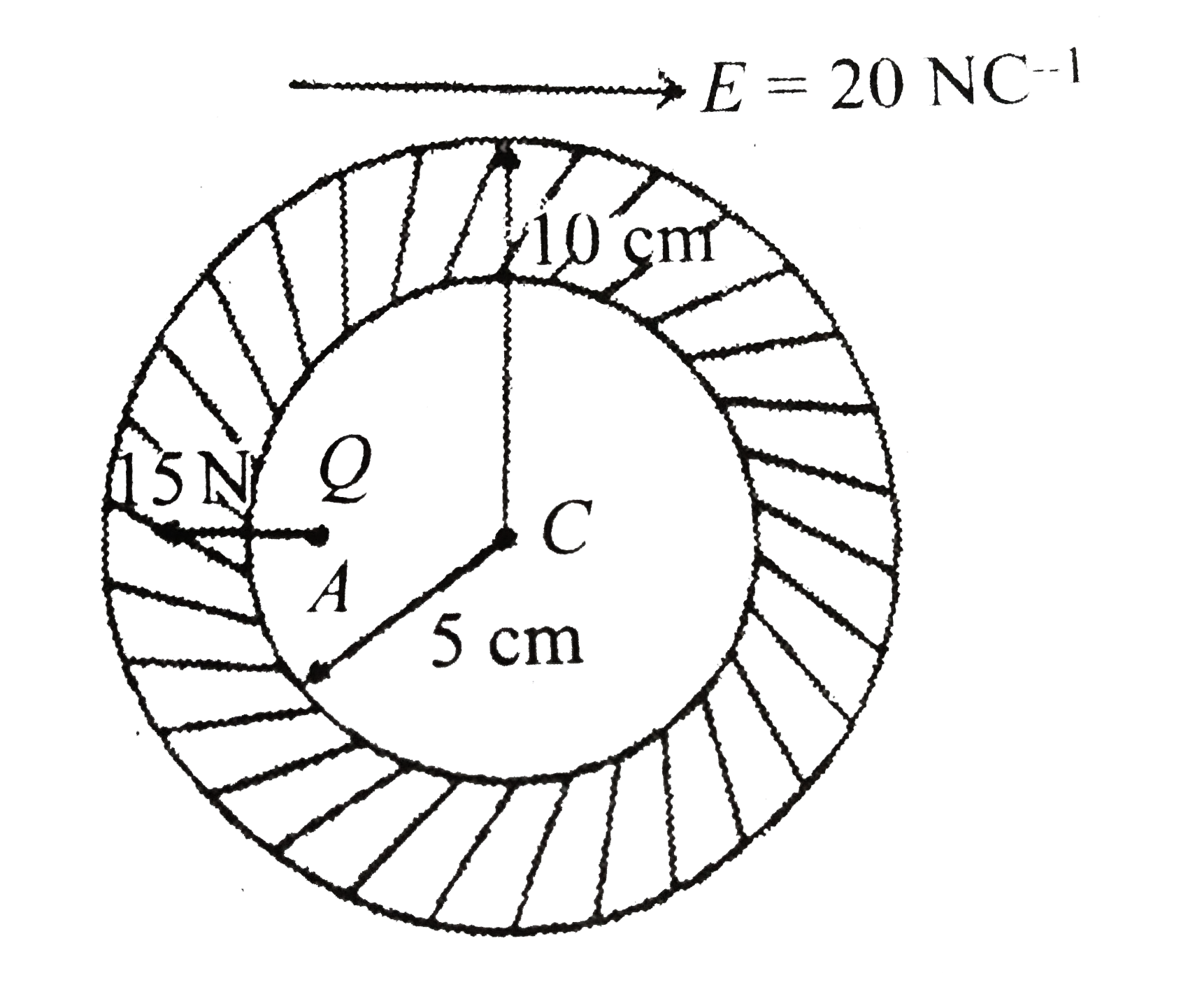
View Solution
