Which statement is true :-
(i) A ring of radius R carries a uniformly distributed charge +Q. A point charge -q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a smiple harmonic motion along the axis of the ring.
(ii) Electrons move from a region of higher potential to that of lower potential
only (i)
only (ii)
(i), (ii)
none of them
The correct Answer is:D
To solve the question, we need to analyze both statements provided in the question:
Statement (i):
"A ring of radius R carries a uniformly distributed charge +Q. A point charge -q is placed on the axis of the ring at a distance 2R from the center of the ring and released from rest. The particle executes a simple harmonic motion along the axis of the ring."
Step 1: Understanding the Setup
- We have a ring with a radius R that has a uniformly distributed positive charge +Q.
- A negative point charge −q is placed on the axis of the ring at a distance 2R from the center of the ring.
Step 2: Analyzing Forces on the Charge
- When the charge −q is released, it will experience a force due to the electric field created by the ring.
- The symmetry of the ring means that the horizontal components of the forces from opposite sides will cancel out, leaving only the axial component of the force acting on the charge.
Step 3: Direction of the Force
- Since the charge −q is negative and the ring has a positive charge, the force acting on −q will be directed towards the ring (attractive force).
- As the charge moves towards the center of the ring, it will accelerate due to the force acting on it.
Step 4: Motion Analysis
- When the charge −q crosses the center of the ring, it will continue to move due to inertia, but the direction of the force will change, pulling it back towards the center.
- This back-and-forth motion around the center of the ring is characteristic of simple harmonic motion (SHM).
Conclusion for Statement (i):
- The charge −q will indeed execute simple harmonic motion along the axis of the ring. Thus, statement (i) is true.
Statement (ii):
"Electrons move from a region of higher potential to that of lower potential."
Step 1: Understanding Electric Potential
- Electric potential is defined such that positive charges move from higher potential to lower potential.
- However, electrons are negatively charged particles.
Step 2: Direction of Electron Movement
- Since electrons are negatively charged, they will move in the opposite direction of the electric field.
- Therefore, electrons actually move from regions of lower potential to regions of higher potential.
Conclusion for Statement (ii):
- The statement is incorrect. Electrons do not move from higher potential to lower potential; they move from lower potential to higher potential. Thus, statement (ii) is false.
Final Answer:
- The correct statement is (i) only.
"A ring of radius R carries a uniformly distributed charge +Q. A point charge -q is placed on the axis of the ring at a distance 2R from the center of the ring and released from rest. The particle executes a simple harmonic motion along the axis of the ring."
- We have a ring with a radius R that has a uniformly distributed positive charge +Q.
- A negative point charge −q is placed on the axis of the ring at a distance 2R from the center of the ring.
- When the charge −q is released, it will experience a force due to the electric field created by the ring.
- The symmetry of the ring means that the horizontal components of the forces from opposite sides will cancel out, leaving only the axial component of the force acting on the charge.
- Since the charge −q is negative and the ring has a positive charge, the force acting on −q will be directed towards the ring (attractive force).
- As the charge moves towards the center of the ring, it will accelerate due to the force acting on it.
- When the charge −q crosses the center of the ring, it will continue to move due to inertia, but the direction of the force will change, pulling it back towards the center.
- This back-and-forth motion around the center of the ring is characteristic of simple harmonic motion (SHM).
- The charge −q will indeed execute simple harmonic motion along the axis of the ring. Thus, statement (i) is true.
"Electrons move from a region of higher potential to that of lower potential."
- Electric potential is defined such that positive charges move from higher potential to lower potential.
- However, electrons are negatively charged particles.
- Since electrons are negatively charged, they will move in the opposite direction of the electric field.
- Therefore, electrons actually move from regions of lower potential to regions of higher potential.
- The statement is incorrect. Electrons do not move from higher potential to lower potential; they move from lower potential to higher potential. Thus, statement (ii) is false.
- The correct statement is (i) only.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - Exercise -3 Section (A)
41 Videos
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - Exercise -3 Section (B) Previous Year Problems | JEE Main
30 Videos
- ELECTROSTATICSBOOK - MOTIONCHAPTER - ELECTROSTATICSEXERCISE - Exercise -1 (Objective Problems | NEET)
105 Videos
- ELECTRONICS - SEMI CONDUCTORBOOK - MOTIONCHAPTER - ELECTRONICS - SEMI CONDUCTOREXERCISE - EXERCISE - 3
29 Videos
- ELECTROSTATICS - I BOOK - MOTIONCHAPTER - ELECTROSTATICS - I EXERCISE - EXERCISE - 4 (Level -II) PREVIOUS YEAR - JEE ADVANCED
13 Videos
Similar Questions
A ring of radius R is with a uniformly distributed charge Q on it .A charge q is now placed in the centre of the ring .Find the increment in tension in the ring.
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View Solution
Knowledge Check
An electron released on the axis of a positively charged ring at a large distance from the centre will
ANot moveBDo oscillatory motionCDo SHMDDo non-periodic motionA ring of radius a contains a charge q distributed uniformly over its length. Find the electric field at a point on the axis of the ring at a distance x from the centre.
Akq2x(a2+x2)32B2kq2x(a2+x2)32Ckq2x(a2+x2)32Dkq22x(a2+x2)32A ring of radius R is charged uniformly with a charge +Q. The potential at a point on its axis at a distance 'r' from any point on ring will be-
AkQ√R2+r2BkQrCkQ(r2+R2)3/2DkQr2(r2−R2)1/2
A ring of radius R with a uniformly distributed charge q as shown in figure. A charge q0, is now placed at the centre of the ring. Find the increment in-the tension in ring.
View SolutionPotential on the axis of ring of radius 3R and at distance 4R from centre of ring is (charge on the ring is Q )
View SolutionThe maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R is at a distance x from the centre of the ring.The value of x is
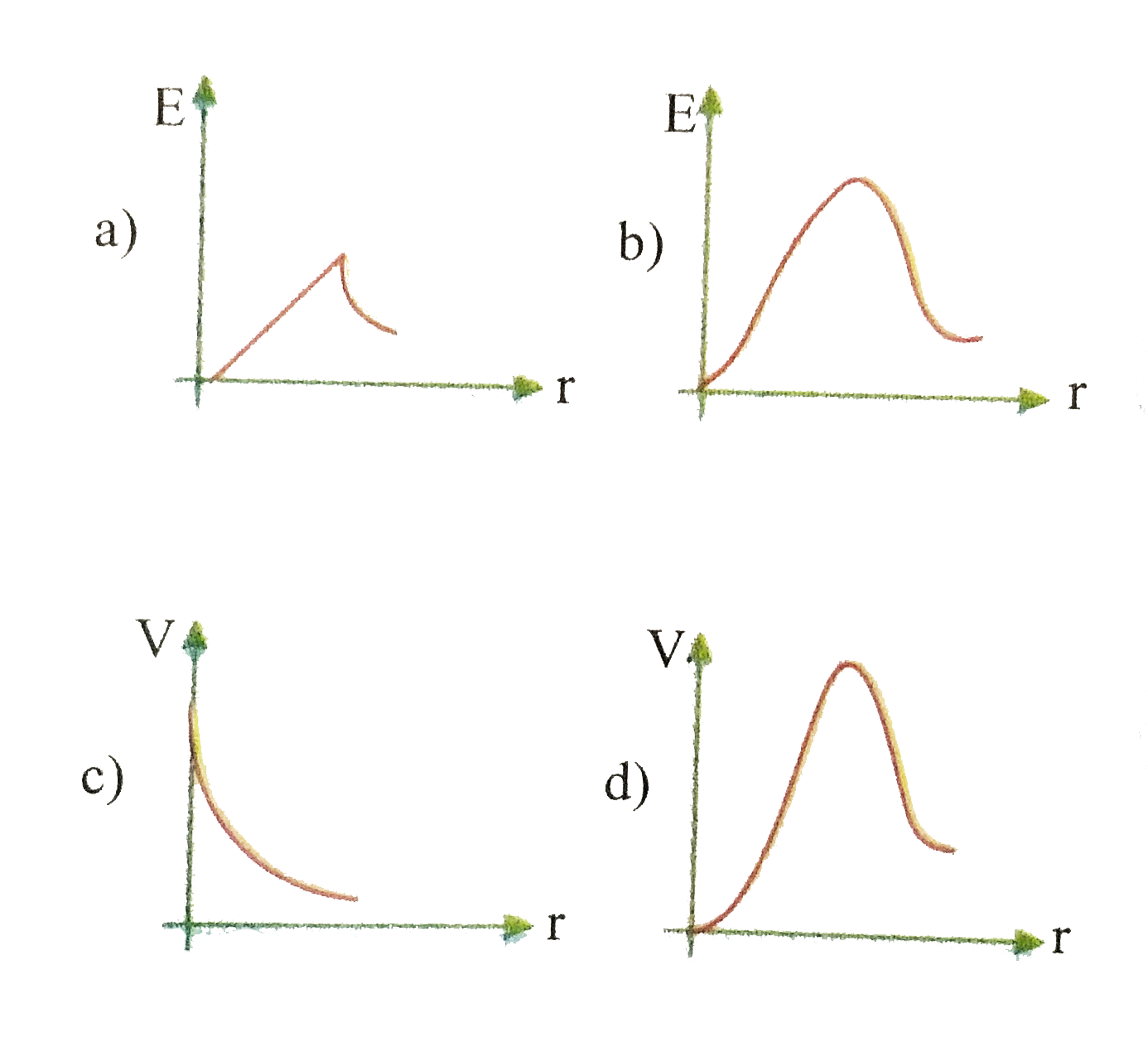
View SolutionA circular ring carries a uniformly distributed positive charge .The electric field(E) and potential (V) varies with distance (r ) from the centre of thr ring along its axis as
View SolutionA ring of radius R carries a charge +q. A test charge −q0 is released on its axis at a distance √3R from its centre. How much kinetic energy will be acquired by the test charge when it reaches at the centre of the ring ?
View Solution
