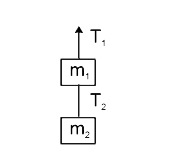
Two blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
the blocks are at rest
The correct Answer is:5 N, 3 N
To solve the problem of finding the tensions in the strings when the blocks are at rest, we will analyze the forces acting on each block separately.
1. Identify the Masses and Gravitational Force:
- Mass of block 1, m1=0.2 kg
- Mass of block 2, m2=0.3 kg
- Acceleration due to gravity, g=10m/s2
2. Calculate the Weight of Each Block:
- Weight of block 1, W1=m1⋅g=0.2kg⋅10m/s2=2N
- Weight of block 2, W2=m2⋅g=0.3kg⋅10m/s2=3N
3. Analyze the Forces on Block 2:
- Let T2 be the tension in the string supporting block 2.
- Since block 2 is at rest, the forces acting on it are balanced:
T2=W2
- Therefore, substituting the weight of block 2:
T2=3N
4. Analyze the Forces on Block 1:
- Let T1 be the tension in the string supporting block 1.
- The forces acting on block 1 are the tension T1 upwards and the combined weight of block 2 and its own weight downwards:
T1=T2+W1
- Substituting the values we have:
T1=3N+2N=5N
5. Final Results:
- The tension in the string supporting block 2 is T2=3N.
- The tension in the string supporting block 1 is T1=5N.
Summary of Tensions:
- T1=5N
- T2=3N
- Mass of block 1, m1=0.2 kg
- Mass of block 2, m2=0.3 kg
- Acceleration due to gravity, g=10m/s2
- Weight of block 1, W1=m1⋅g=0.2kg⋅10m/s2=2N
- Weight of block 2, W2=m2⋅g=0.3kg⋅10m/s2=3N
- Let T2 be the tension in the string supporting block 2.
- Since block 2 is at rest, the forces acting on it are balanced:
T2=W2
- Therefore, substituting the weight of block 2:
T2=3N
- Let T1 be the tension in the string supporting block 1.
- The forces acting on block 1 are the tension T1 upwards and the combined weight of block 2 and its own weight downwards:
T1=T2+W1
- Substituting the values we have:
T1=3N+2N=5N
- The tension in the string supporting block 2 is T2=3N.
- The tension in the string supporting block 1 is T1=5N.
- T1=5N
- T2=3N
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Questions For Practice
25 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 1 (SECTION-A:- String Constrained, Wedge Constrained, Newtons Law theory Question, Equillibrium Questions (Normal and Tension), Problems with Acceleration (F=ma), Wedge problems)
27 Videos
- NEWTON'S LAWS OF MOTION & FRICTION BOOK - MOTIONCHAPTER - NEWTON'S LAWS OF MOTION & FRICTION EXERCISE - Exercise - 3 Section-B
12 Videos
- MODERN PHYSICS -1BOOK - MOTIONCHAPTER - MODERN PHYSICS -1EXERCISE - EXERCISE-4 ( LEVEL- II)
39 Videos
- NLM & FRICTIONBOOK - MOTIONCHAPTER - NLM & FRICTIONEXERCISE - EXERCISE-4 ( LEVEL-II)
15 Videos
Similar Questions
Two blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
they move upward at 5 m/s
View SolutionTwo blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
they accelerate downward at 2m/s2
View Solution
Knowledge Check
The block of mass m is at rest. Find the tension in the string A .
 AmgB2 mgC3 mgD4 mg
AmgB2 mgC3 mgD4 mgThree blocks A,B and C of mass 10 kg each are hanging on a string passing over a fixed frictionless pulley as shown in figure. The tension in the string connecting blocks B and C is (g=10m/s2)
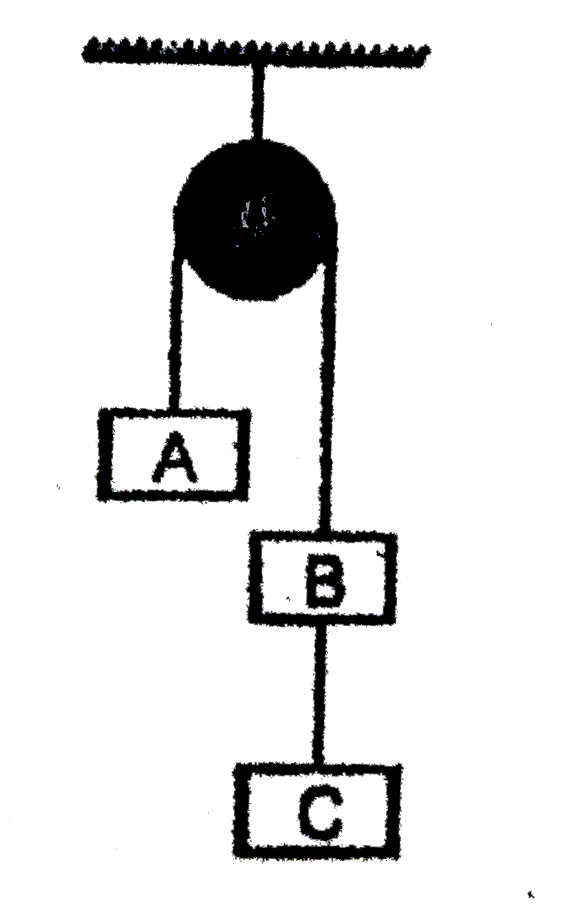 A20003NB4003NC100 ND2003N
A20003NB4003NC100 ND2003NTwo blocks of masses mi=2kg and m2=4kg hang, over a massless pulley as shown in the figure. A force F0=100N acting at the axis of the pulley accelerates the system upwards Then :
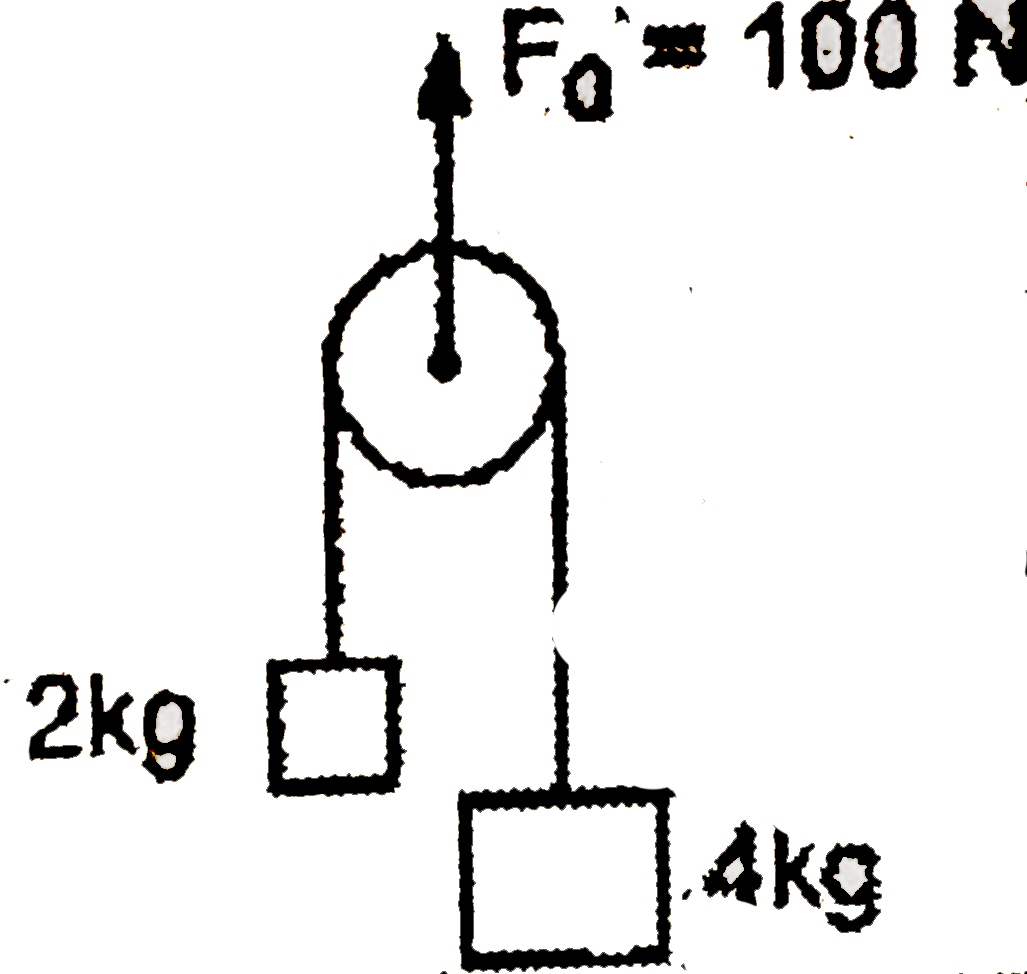 Aacceleration of 2kg mass is 15m/s2Bacceleration of 4kg mass is 2.5m/s2Cacceleration of both masses is sameDacceleration of both the masses is upward
Aacceleration of 2kg mass is 15m/s2Bacceleration of 4kg mass is 2.5m/s2Cacceleration of both masses is sameDacceleration of both the masses is upward
Two blocks with masses m1=0.2 kg and m2=0.3 kg hang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
if maximum allowable tension is 10 N. What is maximum possible upward acceleration ?
View SolutionTwo blocks with masses m1=0.2 kg and m2=0.3 kghang one under other as shown in figure. Find the tensions in the strings (massless) in the following situations :
(g=10m/s2)
they accelerate upward at 2m/s2
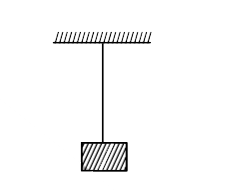
View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
View SolutionIn the figure (g=10m/s2 ) .Acceleration of 2kg block is :

View SolutionTwo blocks of masses m1=2kg and m2=5kg hang over a massless pulley as shown in the figure. A force F0=100N acting at the axis of the pulley accelerates the system upwards. Then :( Take g=9.8m/s2)

View Solution
