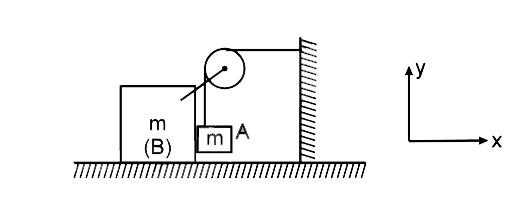
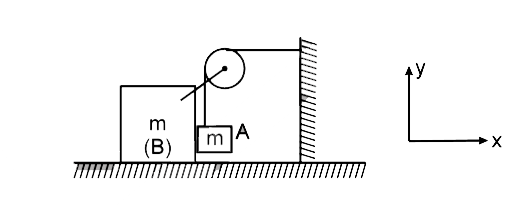
In the system shown in figure, the block A is released from rest. Find
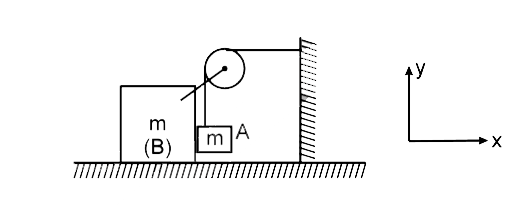
Tension in the string.
The correct Answer is:2mg3
T=2mb=2mg3
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION & FRICTION Book:MOTIONChapter:NEWTON'S LAWS OF MOTION & FRICTION Exercise:Questions For Practice
Explore 25 Videos
- NEWTON'S LAWS OF MOTION & FRICTION Book:MOTIONChapter:NEWTON'S LAWS OF MOTION & FRICTION Exercise:Exercise - 1 (SECTION-A:- String Constrained, Wedge Constrained, Newtons Law theory Question, Equillibrium Questions (Normal and Tension), Problems with Acceleration (F=ma), Wedge problems)
Explore 27 Videos
- NEWTON'S LAWS OF MOTION & FRICTION Book:MOTIONChapter:NEWTON'S LAWS OF MOTION & FRICTION Exercise:Exercise - 3 Section-B
Explore 12 Videos
- MODERN PHYSICS -1Book:MOTIONChapter:MODERN PHYSICS -1Exercise:EXERCISE-4 ( LEVEL- II)
Explore 39 Videos
- NLM & FRICTIONBook:MOTIONChapter:NLM & FRICTIONExercise:EXERCISE-4 ( LEVEL-II)
Explore 15 Videos
Similar Questions
Knowledge Check
The block of mass m is at rest. Find the tension in the string A .
 AmgB2 mgC3 mgD4 mg
AmgB2 mgC3 mgD4 mg
Consider the system shown in fig. the system os released from rest, find the tension in the cord connected between 1kg and 2 kg blocks.

View SolutionThe system shown in figure is released is released from rest . Find acceleration of different string blocks and tenson in different strings.
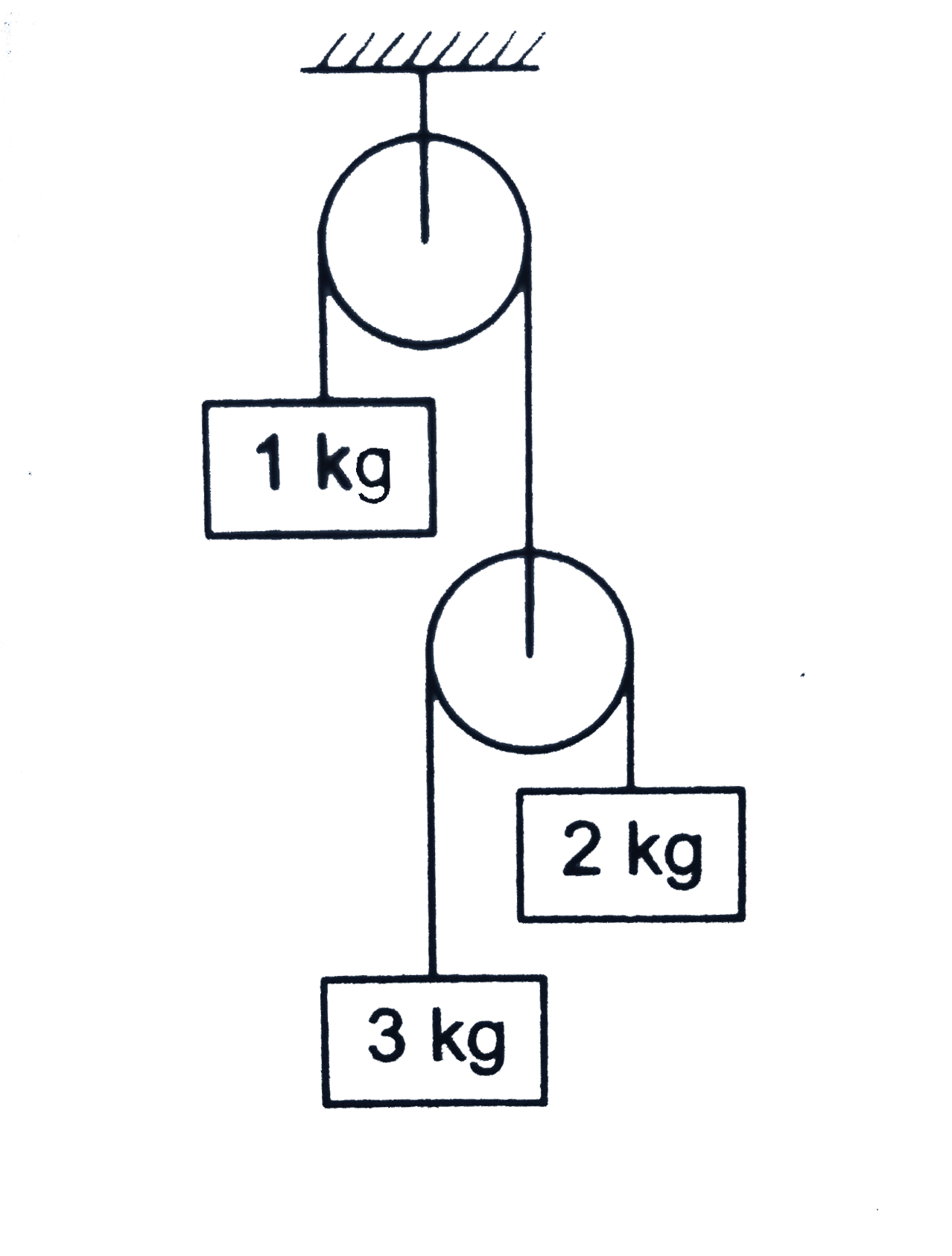
View SolutionThe system shown in fig, is released from rest. Calculate the tension in the string and the force exerted by the string on the pulleys, assuming pulleys and strings are massless.

View SolutionThe system as shown in fig is released from rest. Calculate the tension in the strings and force exerted by the strings on the pulley. Assuming pulleys and strings are massless
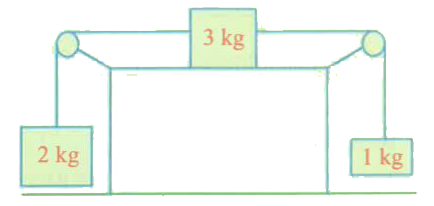
View SolutionFor the system shown in the figure,the pulleys are light and frictionless.The tension in the string will be
View SolutionFor the system shown in figure, the tensions in the string
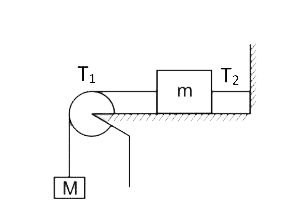
View SolutionIf the system shown in released from rest. Find the net workdone by tension in first one second (g=10m/s2).
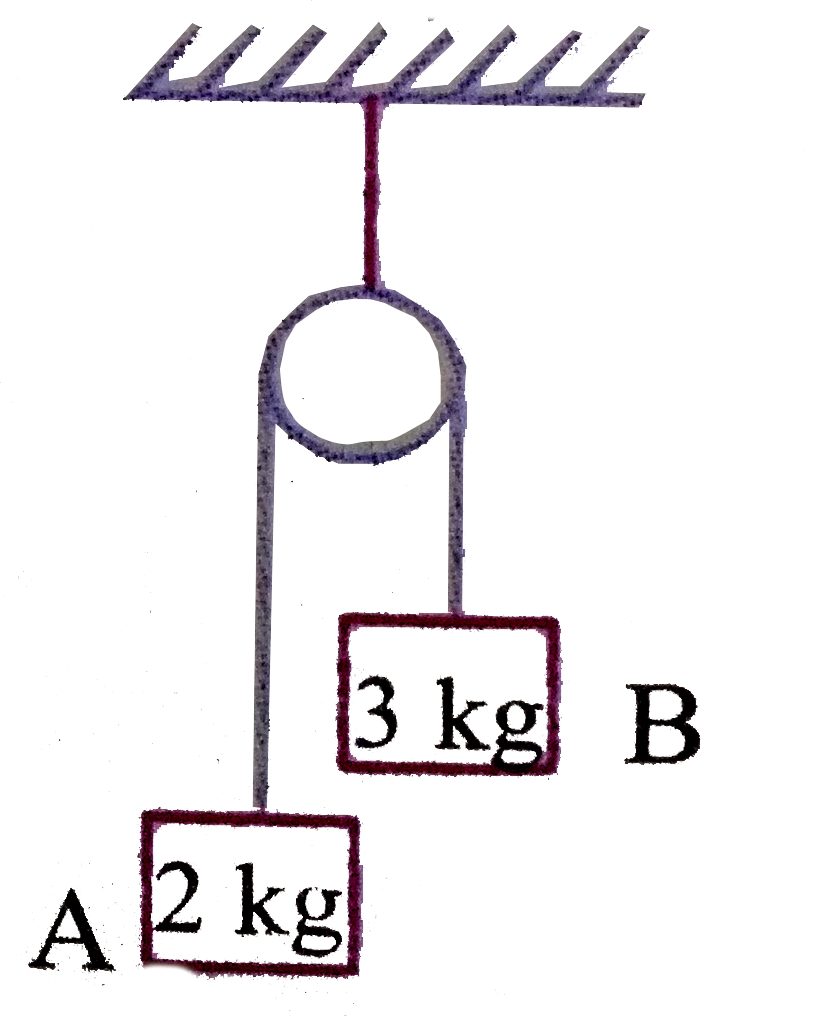 .
.
View Solution