To divide a line segment AB in the ratio 5:6, draw a ray AX such that is an acute angle, the draw a ray BY parallel to AX and the points are located to equal distances on ray AX and BY, respectively. Then, the points joined are
The correct Answer is:A
(a) Given , a line segment AB and we have to divide it in the ratio 5:6.
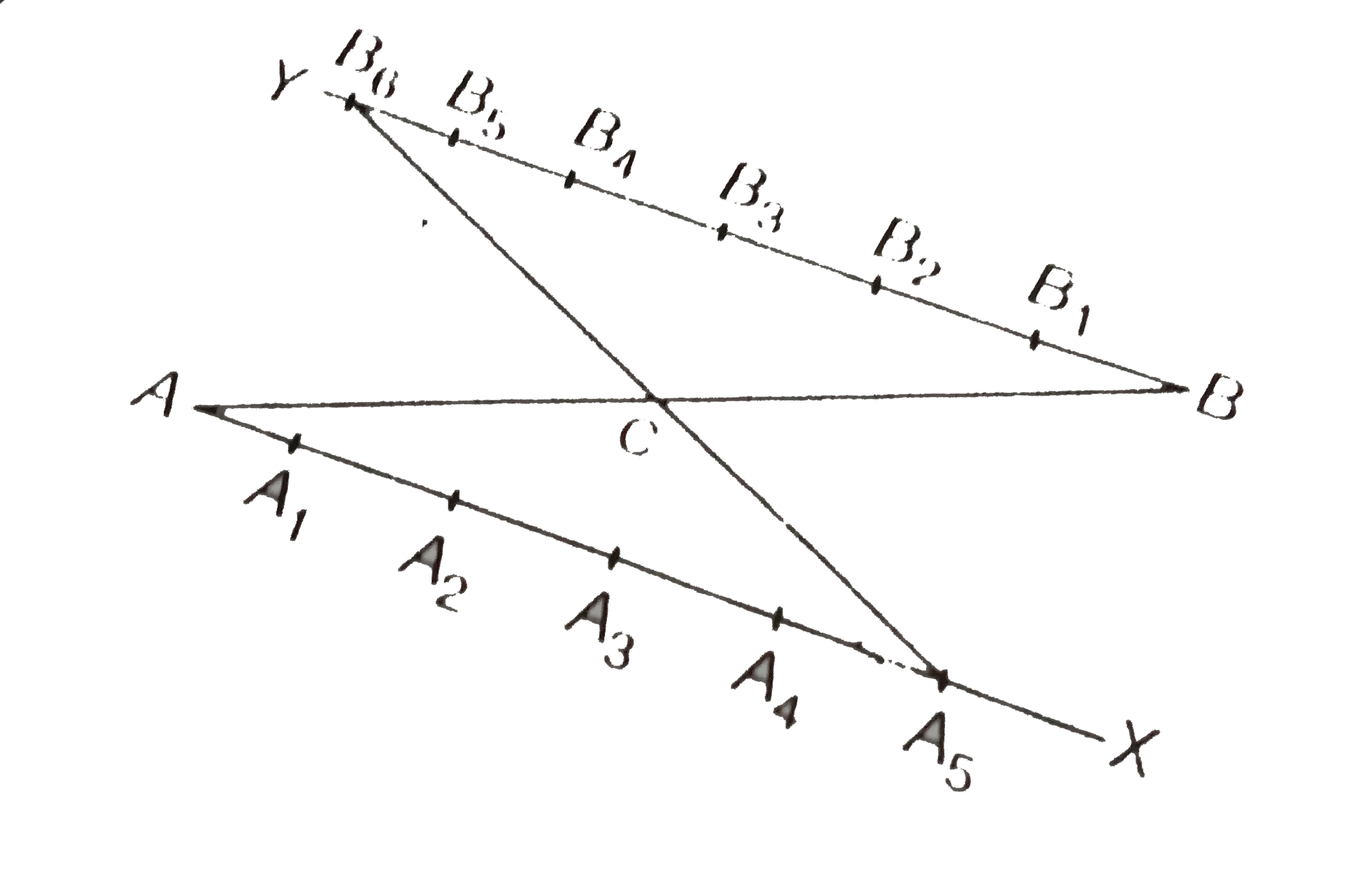
Steps of construction
1. Draw a AX making an acute .
2. Draw a ray BY parallel to AX by making equal to .
3. Now, locate the points (m=5) on AX and s(n=6) such that all the points are at equal distance from each other.
4. Join . Let it intesect AB at a point C.
Then, AC:BC=5:6
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.3 short Answer type Questions4videos
CONSTRUCTIONS
- BOOK - NCERT EXEMPLARCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.4 Long Answer type Questions7videos
CONSTRUCTIONS
- BOOK - NCERT EXEMPLARCHAPTER - CIRCLESEXERCISE - Circles44videos
CIRCLES
- BOOK - NCERT EXEMPLARCHAPTER - COORDINATE GEOMETRYEXERCISE - Coordinate Geometry58videos
COORDINATE GEOMETRY
Similar Questions
Divide a line segment AB in the ratio 4:5, the points A1,A2,A3,....andB1,B2,B3,.... are located at equal distances on the ray AX and BY respectively. Which two points should be joined to divide a line segment?
View SolutionDivide a line segment AB in the ratio 4:5, a ray AX is drawn first such that ∠BAX is an acute angle and then points A1,A2,A3, ........ are located at equal distances on the ray AX which should be joined to B?
View Solution
Knowledge Check
- Question 1 - Select One
To divide a line segment AB in the ratio 4:7, a ray AX is drawn first such that ∠BAX is an acute angle and then points A1,A2,A3,….. are located at equal distance on the ray AX and the point B is joined to
AA12BA11CA10DA9 - Question 2 - Select One
To divide a line segment AB in the ratio 2:5, first a ray AX is drawn, so that BAX is an acute angle and then at equal distance points are marked on the ray AX such that the minimum number of these point is:
A2B5C4D7 - Question 3 - Select One
To divide a line segment AB in the ratio 5:7, first a ray AX is drawn, so that ∠BAX is an acute angle and then at equal distances point are marked on the ray AX such that the minimum number of these points is
A8B10C11D12
In the figure, if B1,B2,B3, and A1,A2,A3,….. have been marked at equal distances. In what ratio C divides AB?
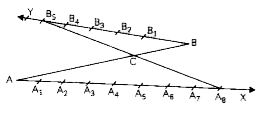 View Solution
View SolutionTo divide a line segment BC internally in the ratio 3 : 5, we draw a ray BX such that ∠ CBX is an acute angle. What will be the minimum number of points to be located at equal distances, on ray BX?
View SolutionDivide a line segment AB in the ratio 3:7, What is the minimum number of points marked on a ray AX at equal distances?
View SolutionTo constuct a triangle similar to a given ΔABC with its sides 73 of the corresponding side of ΔABC, draw a ray BX making acute angle with BC and X lies on the opposite side of A with respect of BC. The points B1,B2,…..,B7 are located at equal distances on BX, B3 is joined to C and then a line segment B6C' is drawn parallel to B3C, where C' lines on BC produced. Finally line segment A'C' is drawn parallel to AC.
View SolutionTo construct a triangle similar to a given ΔABC with its sides 37 of the corresponding sides of ΔABC, first draw a ray BX such that ∠CBX is an acute angle and X lies on the opposite side of A with respect to BC. Then, locate points B1,B2,B3,..... on BX at equal distances and next step is to join
View Solution

