State which of the following are not the probability distributions of a random variable. Give reasons for your answer.
We know, P(X) should be between 0and1.
Also, sum of all the probablities in a probablity distribution should be 1.
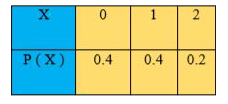
(i) Here, sum of all probablities is 1 and all probablities are between 0 and 1.
So, this is a probablity distribution.
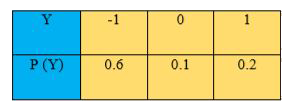
(ii) Here, P(X=3) is negative. So, this is not a probablity distribution.
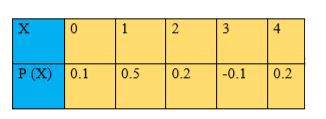
(iii) Here, sum of all probablities is 0.9 which is less than 1.So, this is not a probablity distribution.
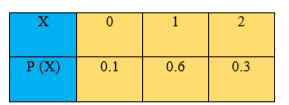
(iv)Here, sum of all probablities is 1.05 which is greater than 1.So, this is not a probablity distribution.
Topper's Solved these Questions
- PROBABILITYBook:NCERTChapter:PROBABILITYExercise:Solved Examples
Explore 36 Videos
- PROBABILITYBook:NCERTChapter:PROBABILITYExercise:EXERCISE 13.1
Explore 17 Videos
- PROBABILITYBook:NCERTChapter:PROBABILITYExercise:EXERCISE 13.2
Explore 18 Videos
- MATRICESBook:NCERTChapter:MATRICESExercise:EXERCISE 3.3
Explore 12 Videos
- RELATIONS AND FUNCTIONSBook:NCERTChapter:RELATIONS AND FUNCTIONSExercise:EXERCISE 1.3
Explore 14 Videos
Similar Questions
State which of the following are not the probability distributions of a random variable. Given reasons for your answer.

View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
View SolutionState which of the following are not probability mass function of random variable.Give reasons of your answers.
View SolutionState whether the following is not the probability mass function of random variable. Give reasons for your answer.

View SolutionWhich of the following probability distribution does not exist for randam variable? Give reason?
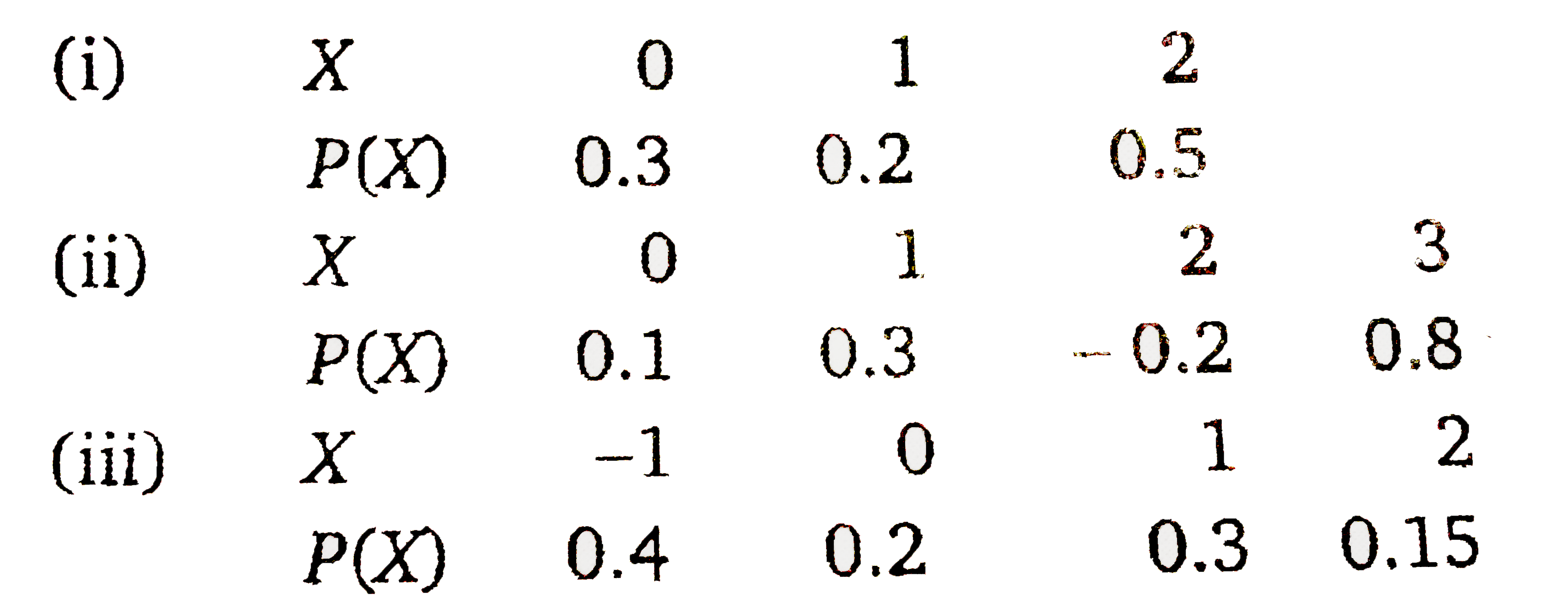
View SolutionConsider the probability distribution of a random variable X.

Variance of X
View SolutionThe following is the probability distribution of a random vaiable X.
The value of k is
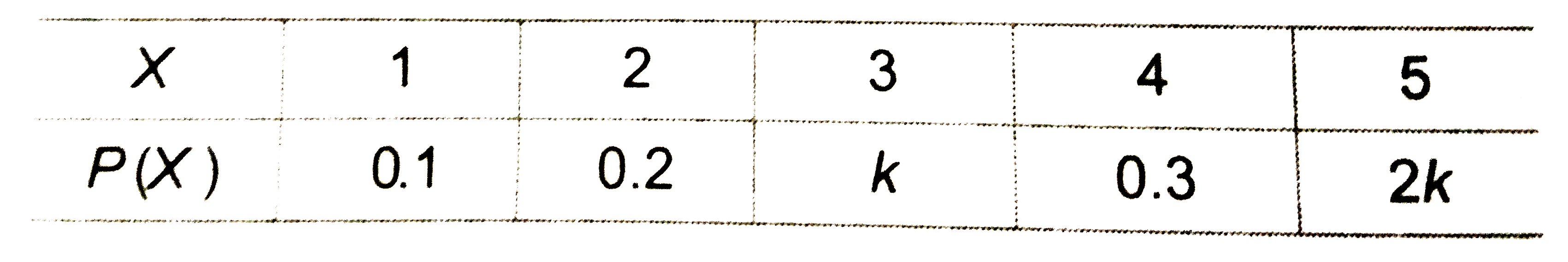
View SolutionThe probability distribution of a random variable is as follows: The variance of x is
View Solution
