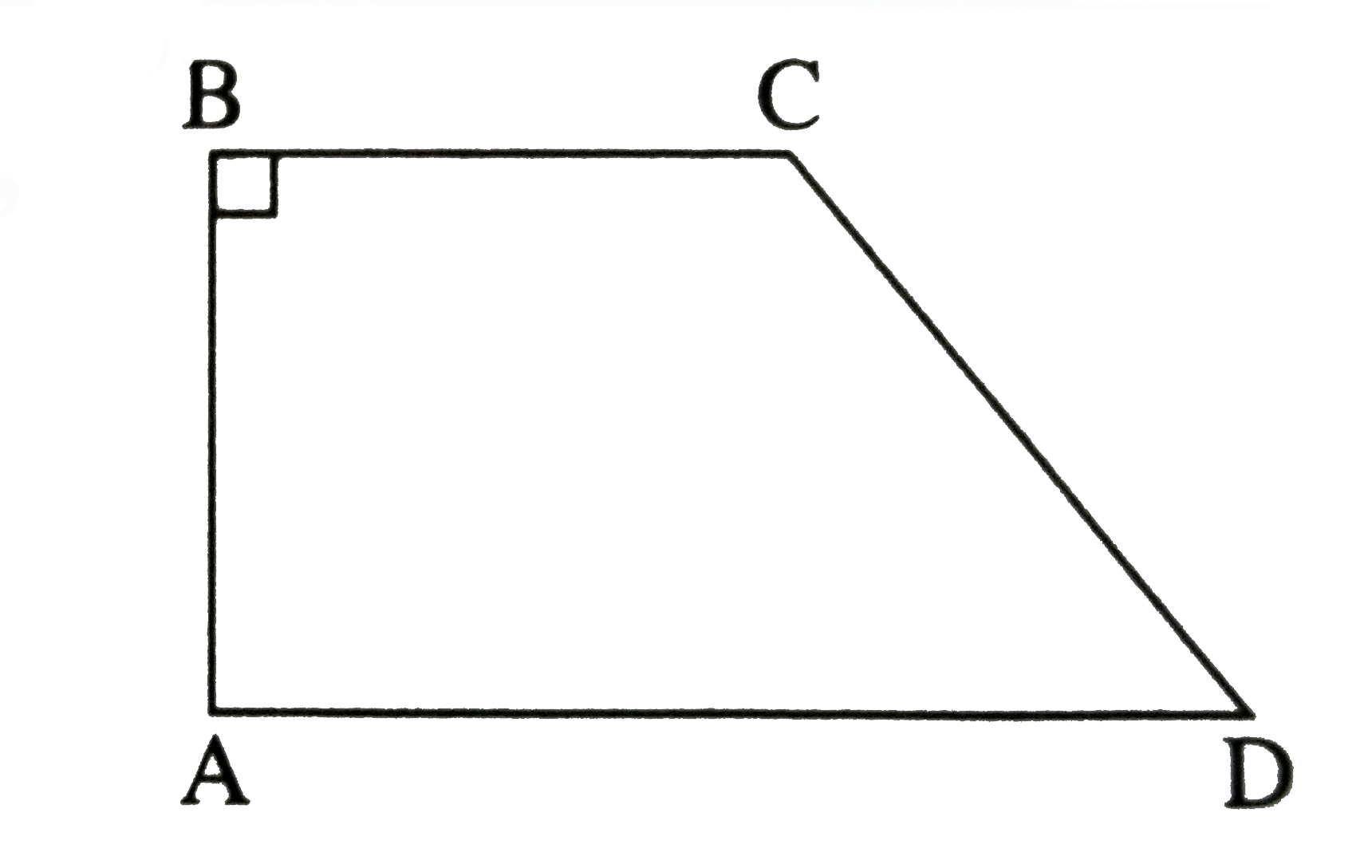
In a quadrilateral ABCD, ∠B=90∘andAD2=AB2+BC2+CD2 prove that ∠ACD=90∘ .
Step by step video & image solution for In a quadrilateral ABCD, angleB=90^(@) and AD^(2)= AB^(2) + BC^(2)+CD^(2) prove that angleACD= 90^(@) . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
In a quadrilateral ABCD, ∠B=90o . If AD2=AB2+BC2+CD2 then prove that ∠ACD=90o .
View SolutionIn the figure ∠B=90∘,
AD2=AB2+BC2+CD2.
Prove ∠ACD=90∘ .
 View Solution
View SolutionIn a quadrilateral ABCD , ∠B=90o , AD2=AB2+BC2+CD2 , prove that ∠ACD=90o
View SolutionIn a quadrilateral ABCD, ∠A+∠D=90∘. Prove that
AC2+BD2=AD2+BC2View SolutionIn a quadrilateral ABCD, ∠A+∠D=90∘. Prove that AC2+BD2=AD2+BC2.
View SolutionIn a ΔABC,∠B=90∘andAC=8√2. If AB=BC, then find AB.
View SolutionIn a quadrilateral ABCD, show that (AB+BC+CD+DA)<2(BD+AC).
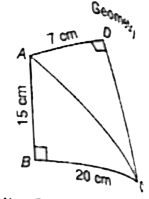
View SolutionIn the given quadrilateral ABCD,AB=15cm,BC=20cmandAD=7cm∠ABC=∠ADC=90∘. Find the length of side CD:
 View Solution
View SolutionIn a quadrilateral ABCD, prove that AB2+BC2+CD2+DA2=AC2+BD2+4PQ2, where P and Q are middle points of diagonals AC and BD.
View SolutionIn a quadrilateral ABCD prove that AB2+BC2+CD2+DA2=AC2+BD2+4PQ2 where P and Q are middle points of diagonals AC and BD.
View Solution

