In figure ABDC is a quadrant of a circle of a radius 28 cm and a semi circles BEC is drawn with BC as diameter find the area of shaded region:
To find the area of the shaded region in the given problem, we will follow these steps:
Step 1: Identify the components
We have a quadrant of a circle (ABDC) with a radius of 28 cm and a semicircle (BEC) with BC as the diameter. We need to find the area of the shaded region, which is the area of the quadrant minus the area of the semicircle.
Step 2: Calculate the area of the quadrant
The area of a quadrant of a circle is given by the formula:
Area of quadrant=14πr2
Substituting r=28 cm:
Area of quadrant=14×227×(28)2
Calculating (28)2=784:
Area of quadrant=14×227×784=22×78428=22×28=616 cm2
Step 3: Find the length of BC using Pythagoras theorem
In triangle ABC, we apply the Pythagorean theorem:
BC2=AB2+AC2
Given AB=28 cm and AC=28 cm:
BC2=282+282=784+784=1568
Thus,
BC=√1568=28√2 cm
Step 4: Calculate the radius of the semicircle
The radius of the semicircle BEC is half of BC:
Radius of semicircle=BC2=28√22=14√2 cm
Step 5: Calculate the area of the semicircle
The area of a semicircle is given by:
Area of semicircle=12πr2
Substituting r=14√2:
Area of semicircle=12×227×(14√2)2
Calculating (14√2)2=392:
Area of semicircle=12×227×392=22×39214=22×28=616 cm2
Step 6: Calculate the area of the shaded region
The area of the shaded region is:
Area of shaded region=Area of quadrant−Area of semicircle
Substituting the areas we calculated:
Area of shaded region=616−392=224 cm2
Final Answer
The area of the shaded region is 224 cm2.
---
We have a quadrant of a circle (ABDC) with a radius of 28 cm and a semicircle (BEC) with BC as the diameter. We need to find the area of the shaded region, which is the area of the quadrant minus the area of the semicircle.
The area of a quadrant of a circle is given by the formula:
Area of quadrant=14πr2
Substituting r=28 cm:
Area of quadrant=14×227×(28)2
Calculating (28)2=784:
Area of quadrant=14×227×784=22×78428=22×28=616 cm2
In triangle ABC, we apply the Pythagorean theorem:
BC2=AB2+AC2
Given AB=28 cm and AC=28 cm:
BC2=282+282=784+784=1568
Thus,
BC=√1568=28√2 cm
The radius of the semicircle BEC is half of BC:
Radius of semicircle=BC2=28√22=14√2 cm
The area of a semicircle is given by:
Area of semicircle=12πr2
Substituting r=14√2:
Area of semicircle=12×227×(14√2)2
Calculating (14√2)2=392:
Area of semicircle=12×227×392=22×39214=22×28=616 cm2
The area of the shaded region is:
Area of shaded region=Area of quadrant−Area of semicircle
Substituting the areas we calculated:
Area of shaded region=616−392=224 cm2
The area of the shaded region is 224 cm2.
Topper's Solved these Questions
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - LONG ANSWER TYPE QUESTIONS
10 Videos
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - PRACTICE-TEST ( AREAS RELATED TO CIRCLES ) SECTION-A
4 Videos
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - SHORT ANSWER TYPE I QUESTIONS
10 Videos
- ARITHMETIC PROGRESSION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ARITHMETIC PROGRESSION EXERCISE - PRACTICE TEST (SECTION D)
1 Video
Similar Questions
In the given figue, ABCD is a quadrant of a circle of radius 28 cm and a semicircle ABECA is drawn with AC as diameter. Find the area of the shaded region.
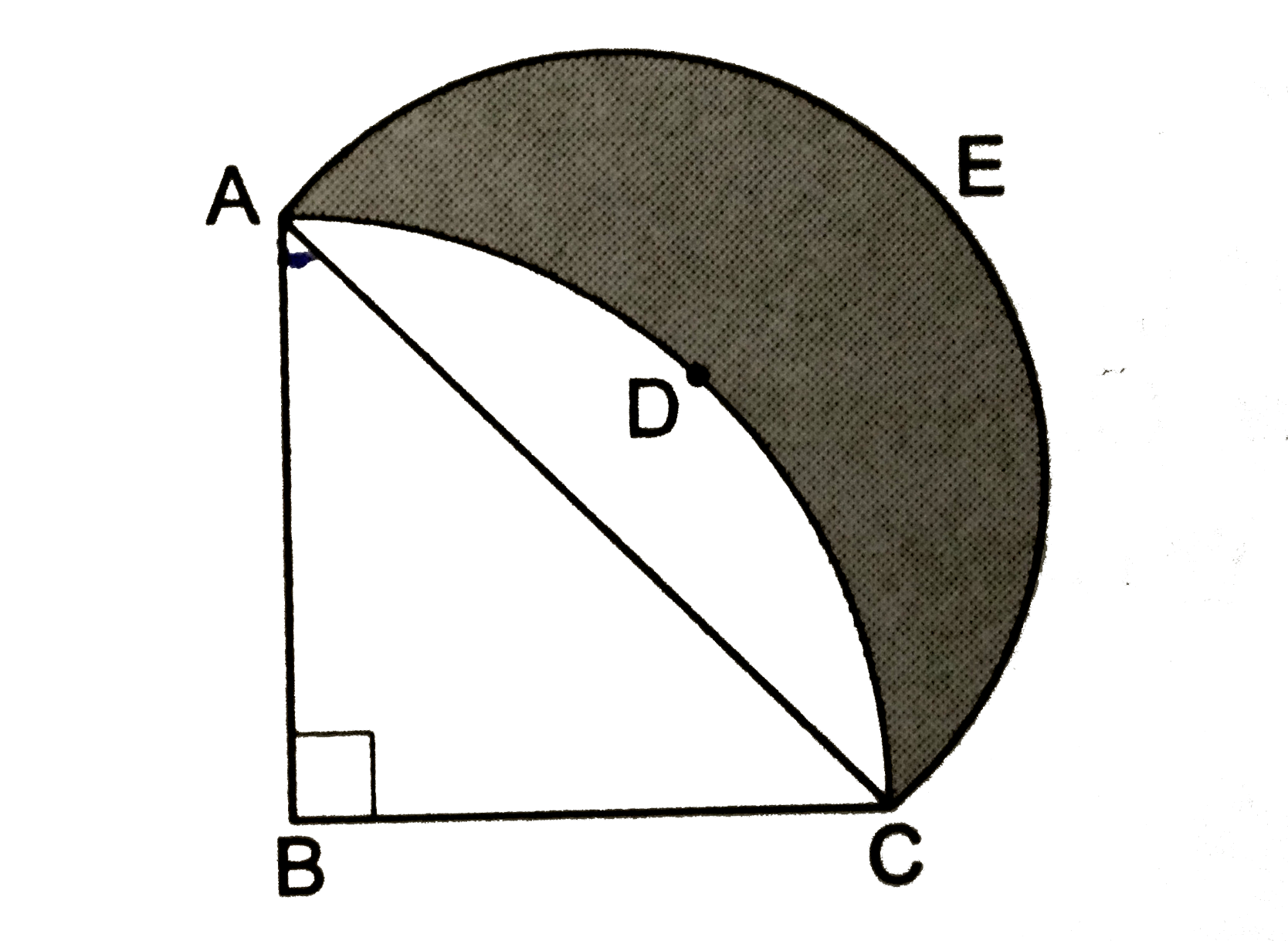
View SolutionIn the given figure, ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region.
View Solution
Knowledge Check
In the adjoining figure ACB is a quadrant with radius 'a'. A semicircle is drawn outside the quadrant taking AB as a diameter . Find the area of shaded
 Aa. 14(π−2a2)Bb. (14)(πa2−a2)Cc. a22Dd. can't be determined
Aa. 14(π−2a2)Bb. (14)(πa2−a2)Cc. a22Dd. can't be determined
In Fig. 12.33, ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region.

View SolutionABCP is a quadrant of a circle of radius 14 cm. with AC as diameter, a semicircle is drawn. Find the area of the shaded portion
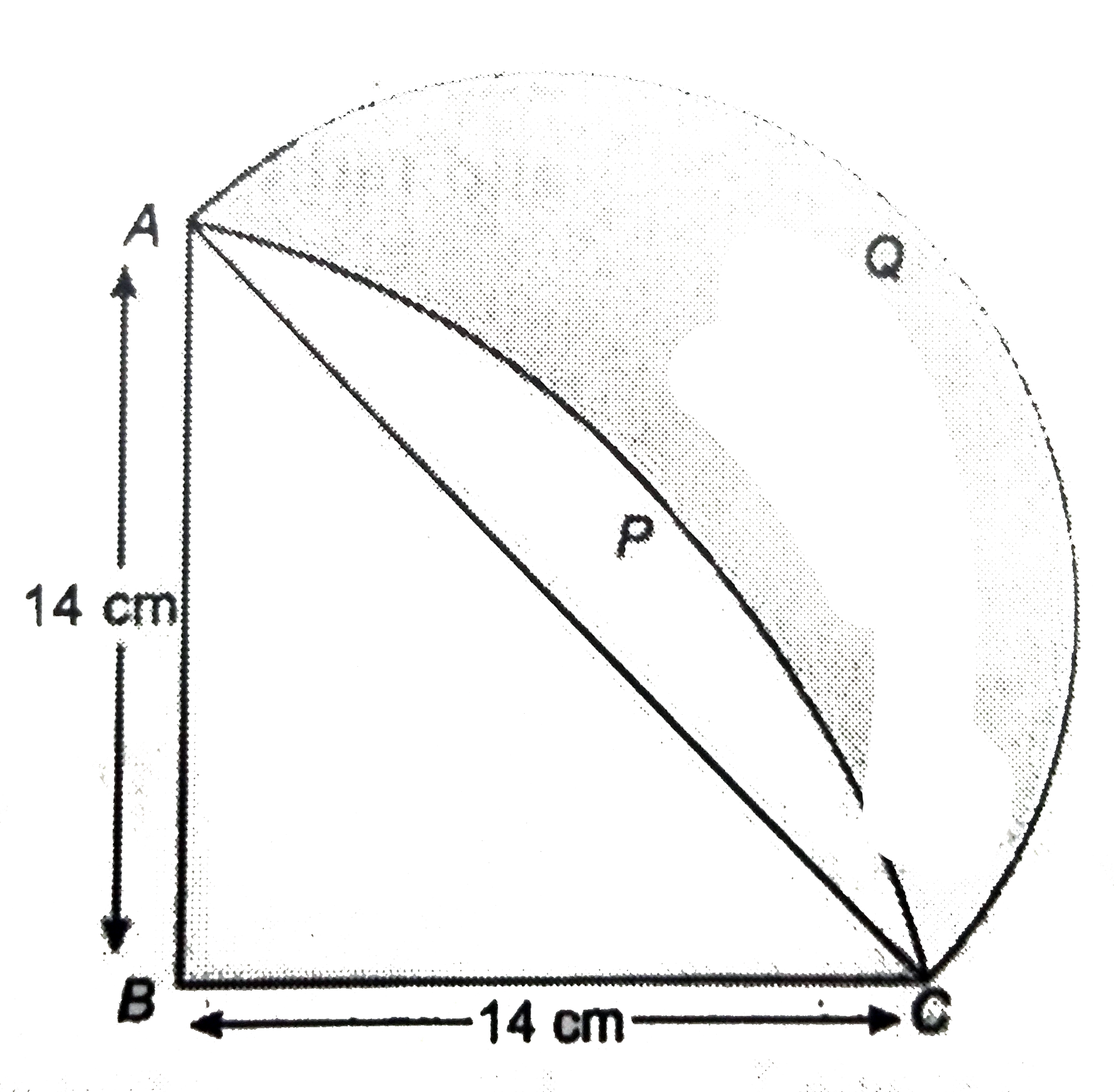
View SolutionBCP is a quadrant of a circle of radius 14cm. With AC as diameter, a semi-circle is drawn. Find the area of the shaded portion.
View SolutionABCP is a quadrant of a circle of radius 14 cm. With AC as diameter, a semi-circle is drawn. Find the area of the shaded portion.
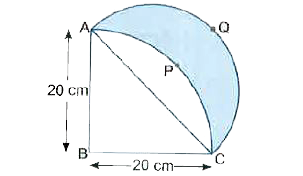
View SolutionIn Fig. 12.64, ABCP is a quadrant of a circle of radius 20 cm. With AC as diameter, a semi-cirde is drawn. Find the area of the shaded portion.
View SolutionIn the figure, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
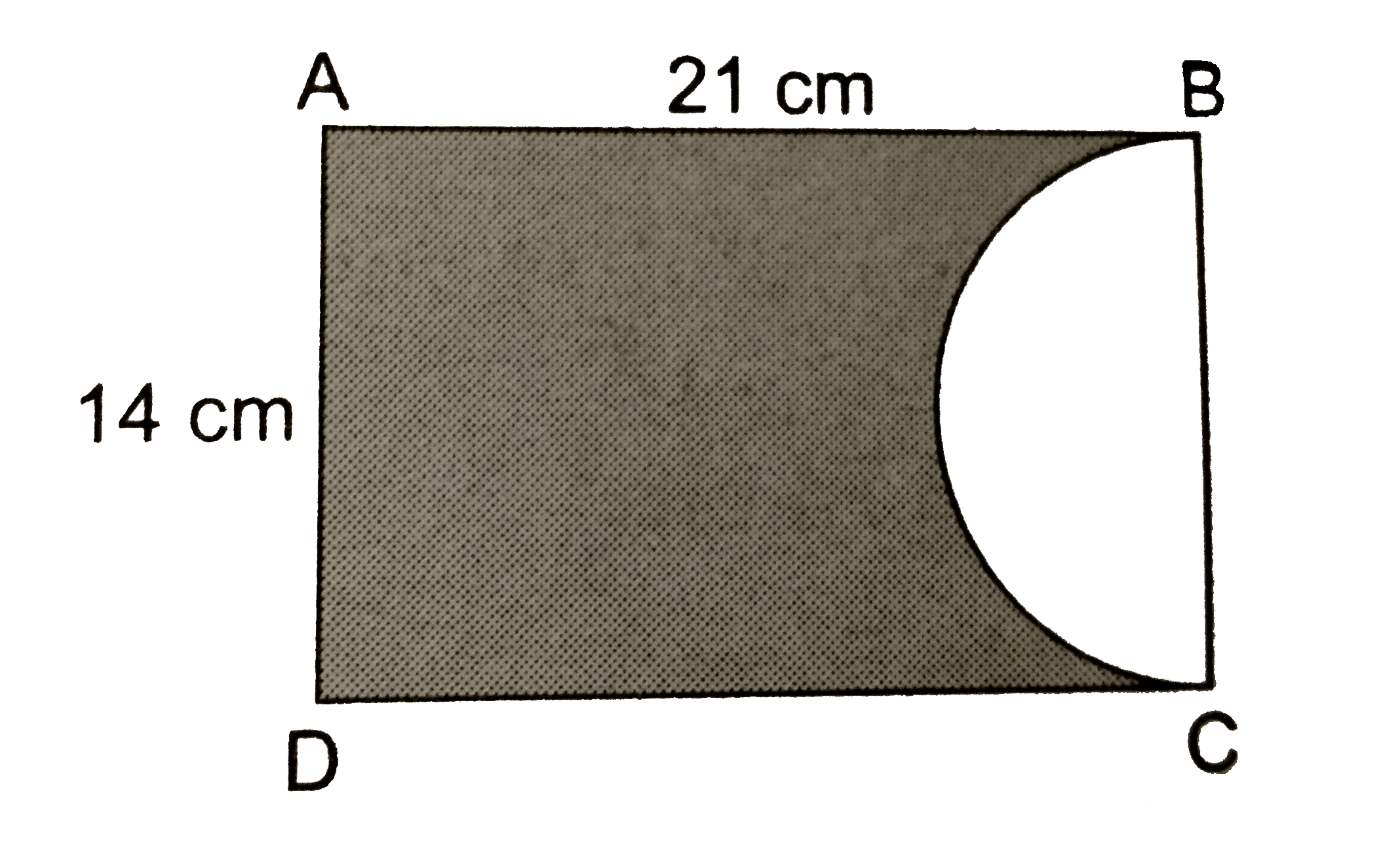
View SolutionIn the given figure ABCD is a rectangle of dimensions 21cm×14cm. A semicircle is drawn with BC as diameter. Find the area and the perimeter of the shaded region in the figure.
View Solution