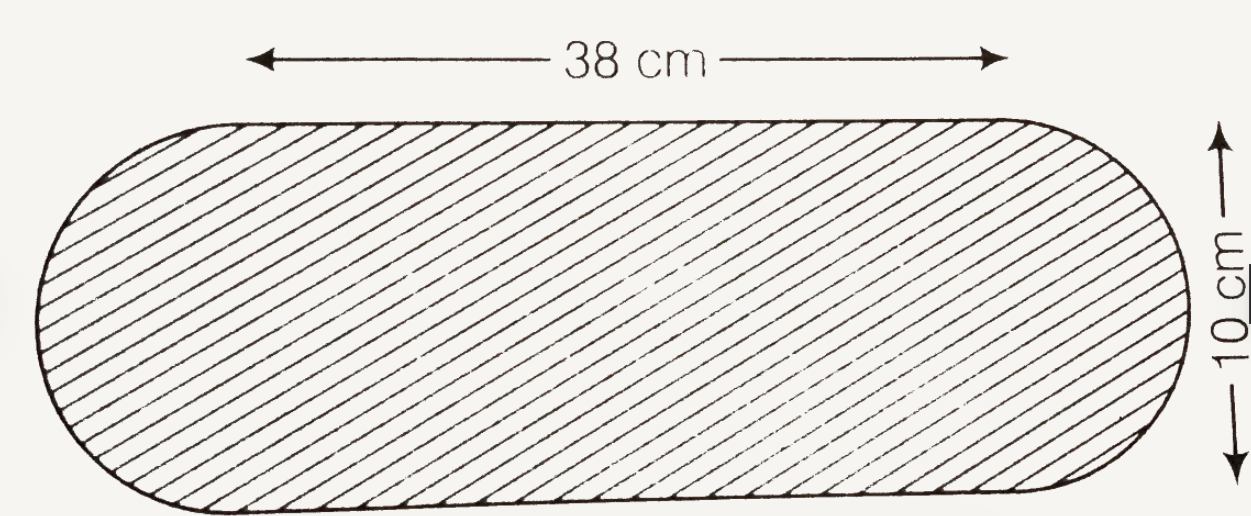
Find the area of the flower bed (with semicircular ends).

Step by step video & image solution for Find the area of the flower bed (with semicircular ends). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - LONG ANSWER TYPE QUESTIONS
10 Videos
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - PRACTICE-TEST ( AREAS RELATED TO CIRCLES ) SECTION-A
4 Videos
- AREAS RELATED TO CIRCLESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - SHORT ANSWER TYPE I QUESTIONS
10 Videos
- ARITHMETIC PROGRESSION BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - ARITHMETIC PROGRESSION EXERCISE - PRACTICE TEST (SECTION D)
1 Videos
Similar Questions
Knowledge Check
The inside perimeter of a practice running track with semicircular ends and straight parallel sides is 312 m. The length of the straight portion of the track is 90 m. If the track has a uniform width of 2 m throughout, find its area.
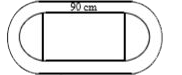 A5166 m2B5802.57 m2C636.57 m2D1273.14m2
A5166 m2B5802.57 m2C636.57 m2D1273.14m2
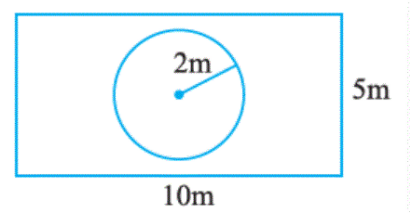
The adjoining figure represents a rectangular lawn with a circular flower bed in the middle. Find:
(i) the area of the whole land
(ii) the area of the flower bed
(iii) the area of the lawn excluding the area of the flower bed
(iv) the circumference of the flower bed.
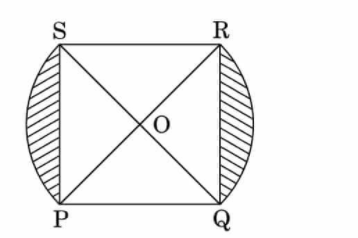
View Solution. In the following figure, PQRS is square lawn with side PQ = 42 metres. Two circular flower beds are there on the sides PS and QR with centre at O, the intersections of its diagonals. Find the total area of the two flower beds (shaded parts).
View SolutionEach side of a square flower bed is 2 m 80 cm long. It is extended by digging a strip 30 cm wide all around it. Find the area of the enlarged flower bed and also the increase in the area of the flower bed.
View SolutionEach side of a square flower bed is 2 m 80 cm long. It is extended by digging a strip 30 cm wide all around it. Find the area of the enlarged flower bed and also the increase in the area of the flower bed.
View SolutionThe given quantity of metal is to be cost into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum, the ratio of the length of the cylinder to the diameter of its semi-circular ends is π:(π+2).
View Solution40. A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum, the ratio of the length of the cylinder to the diameter of its semi-circular ends is w (
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View Solution