A random variable X follows binomial probability distribution with probability P(X), with mean as 2, probability of success as p and probability of failure as q such that p+q=1. If ΣX2P(X)=285, then the probability of exactly 2 success is
3×214510
32×21859
3×(25)10
45×(25)9
The correct Answer is:B
To solve the problem step by step, we will follow the details provided in the video transcript.
Step 1: Understand the Mean of the Binomial Distribution
The mean of a binomial distribution is given by the formula:
Mean=Np
where N is the number of trials and p is the probability of success. We are given that the mean is 2:
Np=2
Step 2: Understand the Variance of the Binomial Distribution
The variance of a binomial distribution is given by:
Variance=Npq
where q=1−p. We are also given that:
ΣX2P(X)=285
Using the formula for variance, we can express it as:
Npq=ΣX2P(X)−Mean2
Substituting the known values:
Npq=285−22=285−4=285−205=85
Step 3: Relate N, p, and q
From the mean, we have:
Np=2
From the variance, we have:
Npq=85
Substituting q=1−p into the variance equation:
Np(1−p)=85
Substituting Np=2:
2(1−p)=85
Solving for p:
1−p=45⟹p=1−45=15
Step 4: Find q
Now, since q=1−p:
q=1−15=45
Step 5: Find N
Using the mean equation:
Np=2⟹N⋅15=2⟹N=2⋅5=10
Step 6: Calculate the Probability of Exactly 2 Successes
The probability of getting exactly r successes in a binomial distribution is given by:
P(X=r)=(Nr)prqN−r
For r=2:
P(X=2)=(102)(15)2(45)10−2
Calculating (102):
(102)=10!2!(10−2)!=10×92×1=45
Now substituting into the probability formula:
P(X=2)=45(15)2(45)8
Calculating:
=45⋅125⋅(4858)=45⋅125⋅65536390625
Simplifying:
=45⋅6553625⋅390625
Calculating the final result:
=29491209765625
Final Answer
Thus, the probability of exactly 2 successes is:
P(X=2)=29491209765625
The mean of a binomial distribution is given by the formula:
Mean=Np
where N is the number of trials and p is the probability of success. We are given that the mean is 2:
Np=2
The variance of a binomial distribution is given by:
Variance=Npq
where q=1−p. We are also given that:
ΣX2P(X)=285
Using the formula for variance, we can express it as:
Npq=ΣX2P(X)−Mean2
Substituting the known values:
Npq=285−22=285−4=285−205=85
From the mean, we have:
Np=2
From the variance, we have:
Npq=85
Substituting q=1−p into the variance equation:
Np(1−p)=85
Substituting Np=2:
2(1−p)=85
Solving for p:
1−p=45⟹p=1−45=15
Now, since q=1−p:
q=1−15=45
Using the mean equation:
Np=2⟹N⋅15=2⟹N=2⋅5=10
The probability of getting exactly r successes in a binomial distribution is given by:
P(X=r)=(Nr)prqN−r
For r=2:
P(X=2)=(102)(15)2(45)10−2
Calculating (102):
(102)=10!2!(10−2)!=10×92×1=45
Now substituting into the probability formula:
P(X=2)=45(15)2(45)8
Calculating:
=45⋅125⋅(4858)=45⋅125⋅65536390625
Simplifying:
=45⋅6553625⋅390625
Calculating the final result:
=29491209765625
Thus, the probability of exactly 2 successes is:
P(X=2)=29491209765625
Topper's Solved these Questions
Similar Questions
Knowledge Check
A random variable has the following probability distribution

The value of p isA110B-1C310DNone of theseA binomial probability distribution is symmertrial .If the probability p of success in a single trial is
Agreater than 1/2BLess than 1/2Cless than q=1−pDequal to 1/2A random variable X has the following probability distribution

Then the variance of this distribution isA0.6B0.7C0.77D0.66
A random variable X has the following probability distribution:
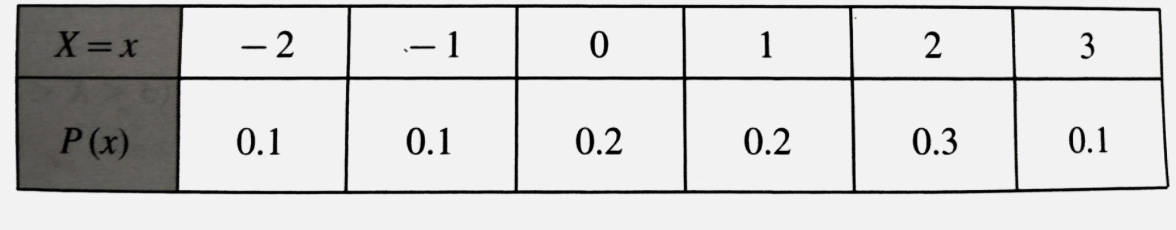
Then E(X) = …….
View SolutionA random variable X has the following probability distribution:

then Var(X)=____
View SolutionA random variable X has the following probability distribution.
Then, the mean of X is

View SolutionIf a random variable X has the following probability distribution values of X
Then P(X≥6) is equal to

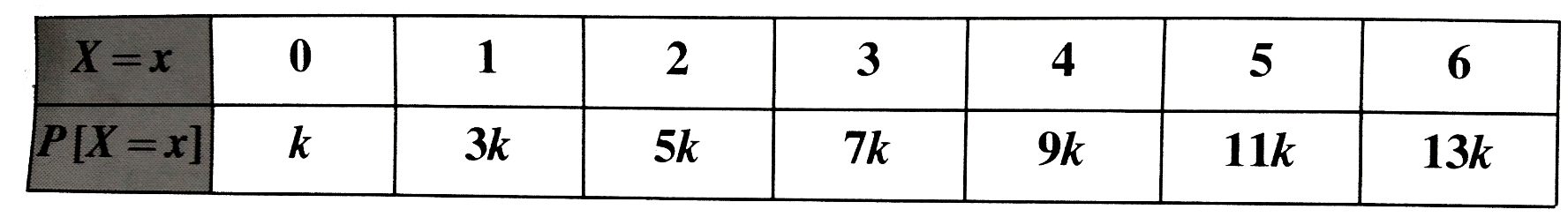
View SolutionA random variable X has the following probability distribution:Determine (i) k (ii) P(X<3)(iii) P(X>6) (iv) P(0<X<3)
View Solution