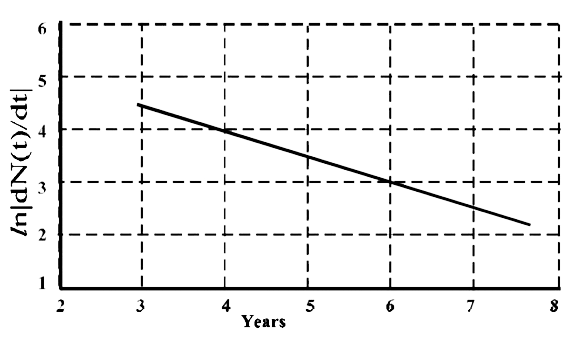
To determine the half life of a radioactive element , a student plot a graph of in $∣ ∣ ∣ \frac{d N (t)}{d t} ∣ ∣ ∣$ versus $t$ , Here $∣ ∣ ∣ \frac{d N (t)}{d t} ∣ ∣ ∣$ is the rate of radiation decay at time t , if the number of radioactive nuclei of this element decreases by a factor of p after $4.16$ year the value of p is

8.5

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

Answer

Step by step video & image solution for To determine the half life of a radioactive element , a student plot a graph of in |(dN(t))/(dt)| versus t , Here |(dN(t))/(dt)| is the rate of radiation decay at time t , if the number of radioactive nuclei of this element decreases by a factor of p after 4.16 year the value of p is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS NTA JEE MOCK TEST 57

Topper's Solved these Questions

Knowledge Check

The $t_{0.5}$ of a radioactive element is related to its average life by the expression
A $T_{12} = 1.44 T_{a v}$
B $T_{12} = 1.36 T_{a v}$
C $T_{12} = 0.693 T_{a v}$
D $T_{12} = \frac{T_{a v}}{0.693}$
The half life of a radioactive element is T and its initial activity at $t = 0$ is $A_{0}$ and at $t = t$ it is A, then
A $A = A_{0} 2^{t / T}$
B $A = A_{0} {(2 t)}^{T}$
C $A_{0} = A 2^{t / T}$
D $A_{0} = A 2^{- t / T}$
If $T$ is the half-life of a radioactive material, then the fraction that would remain after a time $\frac{T}{2}$ is
A $\frac{1}{2}$
B $\frac{3}{4}$
C $\frac{1}{\sqrt{2}}$
D $\frac{\sqrt{2} - 1}{\sqrt{2}}$

NTA MOCK TESTS-NTA JEE MOCK TEST 57-PHYSICS

Home
Ask Doubt
Profile

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

The t0.5 of a radioactive element is related to its average life by the expression

The half life of a radioactive element is T and its initial activity at t=0 is A0 and at t=t it is A, then

If T is the half-life of a radioactive material, then the fraction that would remain after a time T2 is

NTA MOCK TESTS-NTA JEE MOCK TEST 57-PHYSICS

The $t_{0.5}$ of a radioactive element is related to its average life by the expression

The half life of a radioactive element is T and its initial activity at $t = 0$ is $A_{0}$ and at $t = t$ it is A, then

If $T$ is the half-life of a radioactive material, then the fraction that would remain after a time $\frac{T}{2}$ is