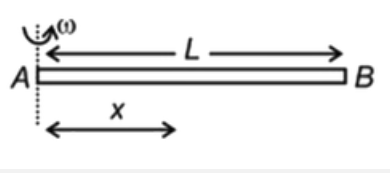
A non - conducting rod of length L with linear charge density λ=λ0x where x is the distance from end A is rotating with constant angular speed ω about the same end. If the angular velocity of the rod (ω) is large, then the magnetic dipole moment of the system is ωλ0L4n. What is the value of n?
The correct Answer is:8
Step by step video & image solution for A non - conducting rod of length L with linear charge density lambda=lambda_(0)x where x is the distance from end A is rotating with constant angular speed omega about the same end. If the angular velocity of the rod (omega) is large, then the magnetic dipole moment of the system is (omega lambda_(0)L^(4))/(n). What is the value of n? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A uniformly charged thin conducting spherical shell of radius R and charge q is rotated with constant angular velocity ω about a diameter. The magnetic dipole moment of the system is
View SolutionFind coordinates of mass center of a non-uniform rod of length L whose linear mass density lambda varies as lambda=a+bx, where x is the distance from the lighter end.
View Solution
Knowledge Check
A uniform rod of length l is rotating with constant angular speed ω as shown in figure. Choose the graph which correctly shows the variation of T with x.
_2019_CBT7_E01_017_Q01.png) A
A_2019_CBT7_E01_017_O01.png) B
B_2019_CBT7_E01_017_O02.png) C
C_2019_CBT7_E01_017_O03.png) D
D_2019_CBT7_E01_017_O04.png)
Find centre of mass of given rod of linear mass density λ=(a+b(xl)2) , x is distance from one of its end. Length of the rod is l.
A3l4(3a+b2a+b)B3l4(2a+b3a+b)Cl4(2a+b3a+b)Dl(2a+b3a+b)A metallic rod of length L rotates at an angular speed ω, normal to a uniform magnetic field. If the resitance of rod is R the heat dissipated is:
A14B2L4ω2tRB12B2L2ωtRC14B2L2ω2t2RD14BL2ω4tR
An insulating rod of length l and charge carries q distributed uniformly on it. The rod is pivoted at its one end and it rotated with angular velocity omega about a fix axis perpendicular to the rod and passing through the pivot. The magnetic moment of rhe system is
View SolutionAn insulting thin rod of length l has a linear charge density ρ(x)=ρ0xl on it. The rod is rotated about an axis passing through the origin (x=0) and perpendicular to the rod. If the rod makes n rotations per second, then the time averaged magnetic moment of the rod is :
View SolutionA uniformly charged disc of radius r and having charge q rotates with constant angular velocity ω. The magnetic dipole moment of this disc is 1nqωr2. Find the value of n.
View SolutionA rod has a total charge Q uniformly distributed along its length L. If the rod rotates with angular velocity ω about its end, compute its magnetic moment.

View SolutionA rod of length l and total charge 'q' which is uniformly distributed is rotating with angular velocity ω about an axis passing through the centre of rod and perpendicular to rod. Find the magnitude of magnetic dipole moment (in Amp. m2) of rod. If q=4C,ω=3rad/s and l=2m
View Solution
