A, B, C and D are four particles with each of mass M lying on the vertices of a square of side a. They always move along a common circle with velocity v under mutual gravitational force. Find v so that they always remain on the vertices of the square
⎷GM(2√2+1)2√2a
⎷GM(√2+1)2√2a
⎷GM√2(2+1)2√2a
none
The correct Answer is:A
To solve the problem, we need to find the velocity v of the particles A, B, C, and D, such that they remain at the vertices of a square while moving in a circular path due to mutual gravitational attraction. Here’s a step-by-step solution:
Step 1: Understand the Geometry
The particles are at the vertices of a square with side length a. The diagonal d of the square can be calculated using the Pythagorean theorem:
d=√a2+a2=a√2
The radius R of the circular path that the particles are moving along is half of the diagonal:
R=d2=a√22=a√2
Step 2: Calculate the Gravitational Force
Each particle experiences a gravitational force due to the other three particles. The gravitational force F between two particles of mass M separated by a distance r is given by:
F=GM2r2
For our configuration, the distance between adjacent particles is a and the distance between diagonal particles is a√2.
Step 3: Find the Net Gravitational Force
The net gravitational force acting on one particle can be calculated by considering the contributions from the other three particles:
- The force due to one adjacent particle is:
F1=GM2a2
- The force due to the diagonal particle is:
F3=GM2(a√2)2=GM22a2
Since there are two adjacent particles contributing equally and one diagonal particle, the net force Fnet on one particle is:
Fnet=2F1+F3=2(GM2a2)+GM22a2=2GM2a2+GM22a2=4GM2+GM22a2=5GM22a2
Step 4: Centripetal Force Requirement
For the particles to move in a circle, the net gravitational force must provide the necessary centripetal force:
Fcentripetal=Mv2R
Setting the gravitational force equal to the centripetal force gives:
5GM22a2=Mv2R
Step 5: Substitute for R
Substituting R=a√2 into the equation:
5GM22a2=Mv2a√2
This simplifies to:
5GM22a2=Mv2√2a
Canceling M from both sides:
5G2a2=v2√2a
Step 6: Solve for v2
Rearranging gives:
v2=5Ga√22
Step 7: Final Expression for v
Taking the square root to find v:
v=√5Ga√22
Conclusion
Thus, the velocity v required for the particles to remain at the vertices of the square while moving in a circle is:
v=√5Ga√22
The particles are at the vertices of a square with side length a. The diagonal d of the square can be calculated using the Pythagorean theorem:
d=√a2+a2=a√2
The radius R of the circular path that the particles are moving along is half of the diagonal:
R=d2=a√22=a√2
Each particle experiences a gravitational force due to the other three particles. The gravitational force F between two particles of mass M separated by a distance r is given by:
F=GM2r2
For our configuration, the distance between adjacent particles is a and the distance between diagonal particles is a√2.
The net gravitational force acting on one particle can be calculated by considering the contributions from the other three particles:
- The force due to one adjacent particle is:
F1=GM2a2
- The force due to the diagonal particle is:
F3=GM2(a√2)2=GM22a2
Since there are two adjacent particles contributing equally and one diagonal particle, the net force Fnet on one particle is:
Fnet=2F1+F3=2(GM2a2)+GM22a2=2GM2a2+GM22a2=4GM2+GM22a2=5GM22a2
For the particles to move in a circle, the net gravitational force must provide the necessary centripetal force:
Fcentripetal=Mv2R
Setting the gravitational force equal to the centripetal force gives:
5GM22a2=Mv2R
Substituting R=a√2 into the equation:
5GM22a2=Mv2a√2
This simplifies to:
5GM22a2=Mv2√2a
Canceling M from both sides:
5G2a2=v2√2a
Rearranging gives:
v2=5Ga√22
Taking the square root to find v:
v=√5Ga√22
Thus, the velocity v required for the particles to remain at the vertices of the square while moving in a circle is:
v=√5Ga√22
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Four particles each of mass m are placed at the vertices of a square of side l. the potential at the centre of square is
A−2√2GmlB−3√2GmlC−2GmlD−4√2Gml - Question 2 - Select One
Find the potential energy of 4-particles, each of mass 1 kg placed at the four vertices of a square of side length 1 m.
A+4.0GB7.5GC−5.4GD+6.3G - Question 3 - Select One
Four particles, each of mass m and charge q, are held at the vertices of a square of side 'a'. They are released at t = 0 and move under mutual repulsive forces speed of any particle when its distance from the centre of square doubles is
A[14π∈0q2ma(1+12√2)]12B(14π∈0q2ma)12C[14π∈02q2ma2(1+12√2)]12D(14π∈0q2ma2)12
Find the work done to bring 4 particle each of mass 100 gram from distances to the vertices of a square of side 20cm.
View SolutionFour particles each of mass m are kept at the four vertices of a square of side 'a' . Find graitational potential energy of this system.
View SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
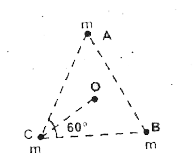
Force acting on particle C, due to particle A and BView SolutionThree particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
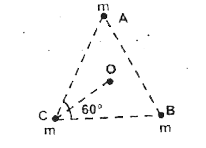
The gravitational potential at O isView SolutionFind the gravitational potential energy of a system of four particles, each of mass m placed at the verticles of a square of side l. Also obtain the gravitaitonal potential at centre of the square.
 View Solution
View Solution

