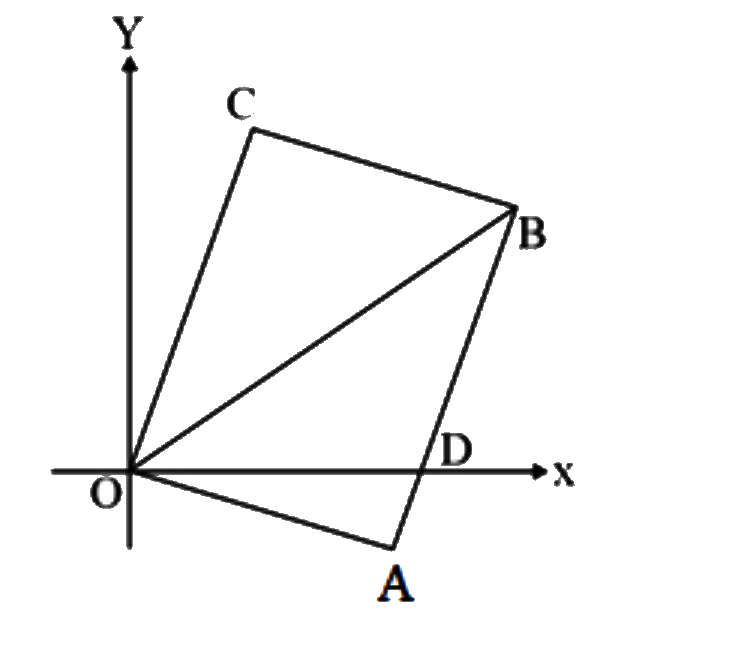
In the figure shown, OABC is a rectangle with OA=3 units, OC = 4 units. If BD = 2.5 units, then the slope of the diagonal OB is equal to
1√2
√2√3
12
13
The correct Answer is:C
Step by step video & image solution for In the figure shown, OABC is a rectangle with OA=3 units, OC = 4 units. If BD = 2.5 units, then the slope of the diagonal OB is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the figure shown, OABC is rectangle with dimensions OA=3 units and OC=4 units. If AD=1.5 units then slope of diagonal OB is
View SolutionIf the length of a rectangle is reduced by 5 units and its breadth is increased by 2 units then the area of the rectangle is reduced by 80 sq units. However, if we increase its length by 10 units and decrease the breadth by 5 units, its area increased by 50 sq units. Find the length and breadth of the rectangle.
View Solution
Knowledge Check
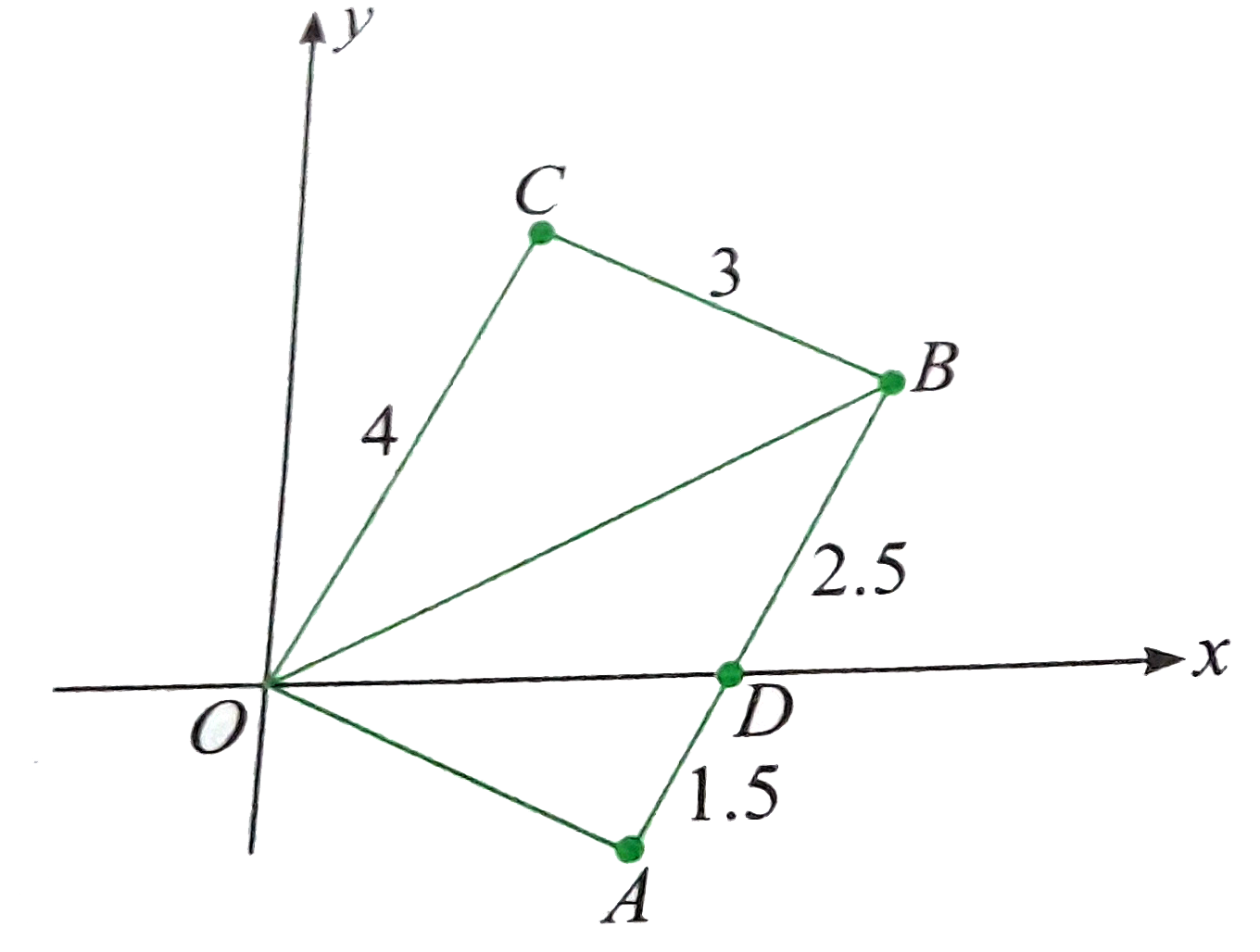
In the given figure, OABC is a rectangle. Slope of OB is
 A1/4B1/3C1/2DCannot be determined
A1/4B1/3C1/2DCannot be determinedIn the given figure, ABCD is a rectangle whose diagonals AC and BD intersect at 0. If ∠OAB=28∘, then ∠OBC is equal to
 A72∘B50∘C62∘D75∘
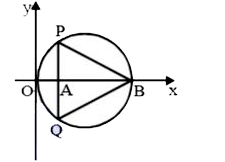
A72∘B50∘C62∘D75∘In the circle given below . Let OA = 1 unit , OB = 13 unit and PQ ⊥ OB. Then the area of the triangle PQB ( in square units)is
 A24√2B24√3C26√3D26√2
A24√2B24√3C26√3D26√2
Let ABCD be a parallelogram the equation of whose diagonals are AC:x+2y=3; BD: 2x + y = 3. If length of diagonalAC=4 units and area of ABCD=8 sq. units. (i) The length of the other diagonal is (ii) the length of side AB is equal to
View SolutionQ. In quadrilateral PORS ,PQ=5 unit QR=7 unit , RS=5 unit and PS=9 unit. the length of the diagonal QS (in units ) can be
View SolutionIf the perimeter of a rectangle is 24 units and the length exceeds the breadth by 4 units, then find the area of a rectangle .
View SolutionIn a ΔABC, if 2AC=3CB, then 2OA+3OB is equal to
View SolutionThe length of rectangle is (7x - 3y) units and its breadth is (3x + 4y) units. The perimeter of the rectangle is
View Solution
