The specific heat of an ideal gas varies with temperature T as
T1
T2
T−2
T0
The correct Answer is:D
To solve the question regarding how the specific heat of an ideal gas varies with temperature T, we can follow these steps:
1. Understand Specific Heat:
Specific heat is defined as the amount of heat required to raise the temperature of a unit mass of a substance by one degree Celsius (or one Kelvin).
2. Behavior of Specific Heat in Ideal Gases:
For ideal gases, the specific heat does not change significantly over a wide range of temperatures. It is often treated as a constant for practical purposes.
3. Negligible Dependence on Temperature:
The specific heat of an ideal gas has a negligible dependence on temperature, meaning that for many calculations, we can assume it remains constant as the temperature changes.
4. Mathematical Representation:
If we denote the specific heat as C, we can express its dependence on temperature T as:
C(T)≈C0
where C0 is a constant value.
5. Evaluating the Options:
- Option 1: C∝T (Directly proportional to temperature) - Incorrect.
- Option 2: C∝T2 - Incorrect.
- Option 3: C∝T−2 - Incorrect.
- Option 4: C∝T0 (Constant) - Correct.
6. Conclusion:
Since the specific heat of an ideal gas can be treated as a constant over a wide range of temperatures, the correct answer is that the specific heat varies as T0, indicating it is independent of temperature.
Final Answer:
The specific heat of an ideal gas varies with temperature T as C∝T0 (constant).
---
Specific heat is defined as the amount of heat required to raise the temperature of a unit mass of a substance by one degree Celsius (or one Kelvin).
For ideal gases, the specific heat does not change significantly over a wide range of temperatures. It is often treated as a constant for practical purposes.
The specific heat of an ideal gas has a negligible dependence on temperature, meaning that for many calculations, we can assume it remains constant as the temperature changes.
If we denote the specific heat as C, we can express its dependence on temperature T as:
C(T)≈C0
where C0 is a constant value.
- Option 1: C∝T (Directly proportional to temperature) - Incorrect.
- Option 2: C∝T2 - Incorrect.
- Option 3: C∝T−2 - Incorrect.
- Option 4: C∝T0 (Constant) - Correct.
Since the specific heat of an ideal gas can be treated as a constant over a wide range of temperatures, the correct answer is that the specific heat varies as T0, indicating it is independent of temperature.
The specific heat of an ideal gas varies with temperature T as C∝T0 (constant).
Topper's Solved these Questions
Similar Questions
How does specific heat of a solid vary with temperature?
View SolutionThe specific heat of a metal at low temperatures varies according to S=(45)T3, where T is the absolute temperature, Find the heat energy (in SI unit) needed to rate the temperature of unit mass of the metal from T=1K to T=2K
View Solution
Knowledge Check
The ednsity (ρ) of an ideal gas varies with temperature T as shown in figure. Then
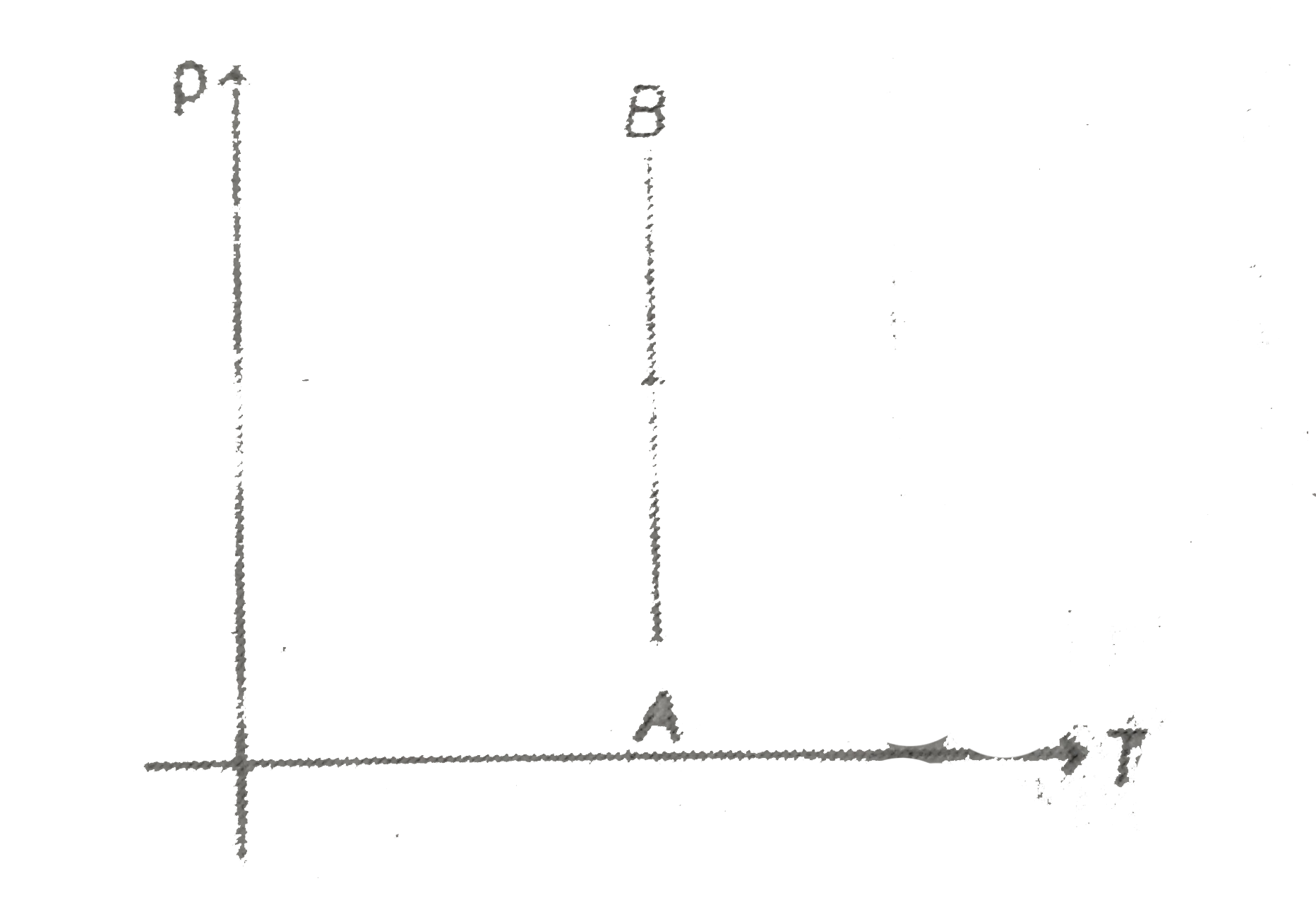 Athe product of P and V at A is equal to the product of P and V at BBpressure at B is greater than the pressure at ACwork done by the gas during process AB is negativeDthe change in internal energy from the A to B is zero
Athe product of P and V at A is equal to the product of P and V at BBpressure at B is greater than the pressure at ACwork done by the gas during process AB is negativeDthe change in internal energy from the A to B is zeroThe specific heat of a substance varies with temperature t(.∘C) as
c=0.20+0.14t+0.023t2(cal/g∘/C)
The heat required to raise the temperature of 2 g of substance from 5∘C to 15∘C will beA24 calB56 calC82 calD100 calThe specific heat of an ideal gas is proportional to
AT∘BT−1CT1/2DT3
The specific heat of a metal at low temperature varies according to S=(4/5)T3 where T is the absolute temperature. Find the heat energy needed to raise unit mass of the metal from T = 1 K →T= 2K.
View SolutionHow specific heat capacity of a substance varies with temperature?
View SolutionSpecific heat of a metal at very low temperatures varies as
View SolutionThe specific heat of many solids at low temperatures varies with absolute temperature T according to the relation S=AT3, where A is a constant. The heat energy required to raise the temperature of a mass m of such a solid from T = 0 to T = 20 K is
View SolutionThe specific heat of a metal at low temperatures varies according to S=aT3, where a is a constant and T is absolute temperature. The heat energy needed to raise unit mass of the metal from temperature T=1K to T=2K is
View Solution
