A and B participate in a race with acceleration a1anda2 , respectively . A reaches t times earlier than B at finish line and their velocities at finish line are v1andv2 , respectively. If difference between their velocities is v , then find the value of v
a1+a22t
√a1a2t
a1a2a1+a2t
2a1a2a1+a2t
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of both A and B in the race, using the equations of motion and the information provided.
Step 1: Define Variables
Let:
- a1 = acceleration of A
- a2 = acceleration of B
- t1 = time taken by A to finish the race
- t2 = time taken by B to finish the race
- t = time difference, such that t2=t1+t
- v1 = final velocity of A
- v2 = final velocity of B
- v = difference in their final velocities, v=v1−v2
Step 2: Write the Equations for Final Velocities
Using the equations of motion, the final velocities can be expressed as:
- For A:
v1=a1t1
- For B:
v2=a2t2=a2(t1+t)
Step 3: Set Up the Equation for Velocity Difference
From the problem, we know that:
v=v1−v2
Substituting the expressions for v1 and v2:
v=a1t1−a2(t1+t)
This simplifies to:
v=a1t1−a2t1−a2t
v=(a1−a2)t1−a2t
Step 4: Write the Equations for Distances
Since both A and B cover the same distance S:
- For A:
S=12a1t21
- For B:
S=12a2t22=12a2(t1+t)2
Step 5: Equate the Distances
Equating the distances gives:
12a1t21=12a2(t1+t)2
Cancelling 12 from both sides:
a1t21=a2(t21+2tt1+t2)
Step 6: Rearranging the Equation
Rearranging gives:
a1t21=a2t21+2a2tt1+a2t2
(a1−a2)t21−2a2tt1−a2t2=0
Step 7: Solve for t1
This is a quadratic equation in t1:
(a1−a2)t21−2a2tt1−a2t2=0
Using the quadratic formula:
t1=−(−2a2t)±√(−2a2t)2−4(a1−a2)(−a2t2)2(a1−a2)
This simplifies to:
t1=2a2t±√4a22t2+4a2t2(a1−a2)2(a1−a2)
t1=a2t±√a22t2+a2t2(a1−a2)(a1−a2)
Step 8: Substitute t1 Back to Find v
Now substitute t1 back into the equation for v:
v=(a1−a2)t1−a2t
After simplification, we find:
v=√a1a2t
Final Result
Thus, the difference in their velocities is:
v=√a1a2t
Let:
- a1 = acceleration of A
- a2 = acceleration of B
- t1 = time taken by A to finish the race
- t2 = time taken by B to finish the race
- t = time difference, such that t2=t1+t
- v1 = final velocity of A
- v2 = final velocity of B
- v = difference in their final velocities, v=v1−v2
Using the equations of motion, the final velocities can be expressed as:
- For A:
v1=a1t1
- For B:
v2=a2t2=a2(t1+t)
From the problem, we know that:
v=v1−v2
Substituting the expressions for v1 and v2:
v=a1t1−a2(t1+t)
This simplifies to:
v=a1t1−a2t1−a2t
v=(a1−a2)t1−a2t
Since both A and B cover the same distance S:
- For A:
S=12a1t21
- For B:
S=12a2t22=12a2(t1+t)2
Equating the distances gives:
12a1t21=12a2(t1+t)2
Cancelling 12 from both sides:
a1t21=a2(t21+2tt1+t2)
Rearranging gives:
a1t21=a2t21+2a2tt1+a2t2
(a1−a2)t21−2a2tt1−a2t2=0
This is a quadratic equation in t1:
(a1−a2)t21−2a2tt1−a2t2=0
Using the quadratic formula:
t1=−(−2a2t)±√(−2a2t)2−4(a1−a2)(−a2t2)2(a1−a2)
This simplifies to:
t1=2a2t±√4a22t2+4a2t2(a1−a2)2(a1−a2)
t1=a2t±√a22t2+a2t2(a1−a2)(a1−a2)
Now substitute t1 back into the equation for v:
v=(a1−a2)t1−a2t
After simplification, we find:
v=√a1a2t
Thus, the difference in their velocities is:
v=√a1a2t
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
AzeroBπ/4Cπ/2DπLet v and a denote the velocity and acceleration respectively of a body.
Aa can be nonzero when v = 0Ba must be zero when v = 0Ca may be zero when v≠0DThe direction of a must have some correlation with the direction of v.Two cars start in a race with velocities u1 and u2 and travel in a straight line with acceleration α and β. If both reach the finish line at the same time, the range of the race is
A2(u1−u2)(β−α)2(u1β−u2α)B2(u1−u2)β+α(u1α−u2β)C(2u1−u2)2(β−α)2D2u1u2βα
The velocities of our molecules are V, 2V, 4V and √2V, respectively. Their r.m.s. speed is
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
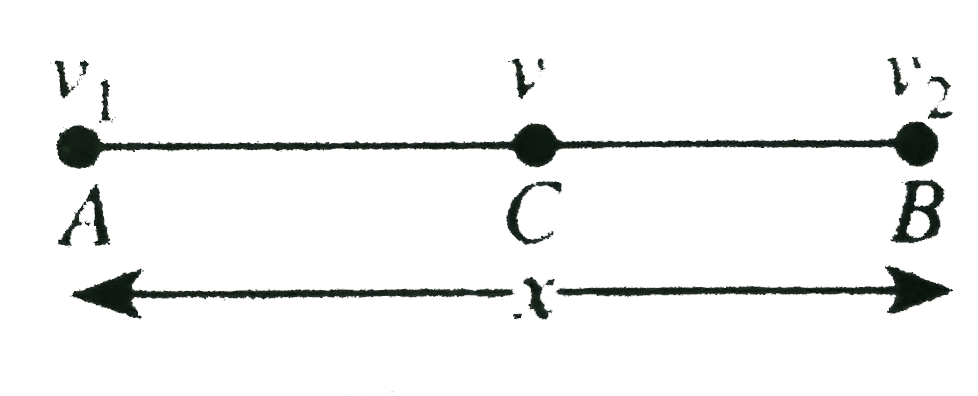 .
.
View SolutionVelocity of light ray in two media A and Bare v1andv2 respectively.
If vB=1.5VA, then which medium is denser?
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionThe velocity of sound waves in an ideal gas at temperatures T1KandT2 are respectively v1andv2 . The rms velocity of gas molecules at these two temperatures are w1ande2 , respectively then
View Solution