For a sphere made out of a certain material, the moment of inertia of the sphere is proportional to [ radius of the sphere = R ]
R2
R3
R4
R5
The correct Answer is:A
To solve the question regarding the moment of inertia of a sphere made from a certain material, we need to analyze the relationship between the moment of inertia and the radius of the sphere.
1. Understanding Moment of Inertia:
The moment of inertia (I) of an object is a measure of its resistance to rotational motion about an axis. For a sphere, it depends on both its mass (m) and its radius (R).
2. Moment of Inertia for a Solid Sphere:
For a solid sphere, the moment of inertia about an axis through its center is given by the formula:
I=25mR2
Here, m is the mass of the sphere and R is its radius.
3. Moment of Inertia for a Hollow Sphere:
For a hollow sphere, the moment of inertia about an axis through its center is given by:
I=23mR2
Again, m is the mass of the hollow sphere and R is its radius.
4. Proportionality to Radius:
In both cases (solid and hollow), we can observe that the moment of inertia is proportional to R2. This means that if we increase the radius of the sphere, the moment of inertia increases with the square of the radius.
5. Conclusion:
Therefore, we conclude that the moment of inertia I of a sphere is proportional to the square of its radius R. Thus, we can express this relationship as:
I∝R2
The moment of inertia (I) of an object is a measure of its resistance to rotational motion about an axis. For a sphere, it depends on both its mass (m) and its radius (R).
For a solid sphere, the moment of inertia about an axis through its center is given by the formula:
I=25mR2
Here, m is the mass of the sphere and R is its radius.
For a hollow sphere, the moment of inertia about an axis through its center is given by:
I=23mR2
Again, m is the mass of the hollow sphere and R is its radius.
In both cases (solid and hollow), we can observe that the moment of inertia is proportional to R2. This means that if we increase the radius of the sphere, the moment of inertia increases with the square of the radius.
Therefore, we conclude that the moment of inertia I of a sphere is proportional to the square of its radius R. Thus, we can express this relationship as:
I∝R2
Topper's Solved these Questions
Similar Questions
Find the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
View Solution(a) Find the moment of inertia of a sphere about a tangent to the sphere, given the moment of inertia of the sphere about any of its diameters to be 2MR2/5,whereM is the mass of the sphere and R is the radius of the sphere.
(b) Given the moment of inertia of a disc of mass M and radius R about any of its diameters to be 14MR2, find the moment of inertia about an axis normal to the disc passing through a point on its edge.
View Solution
Knowledge Check
A sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
A5kgm2B2.7kgm2C3.5kgm2D4.5kgm2A sphere of mass 10 kg and radius 0.5 m rotates about a tangent. The moment of inertia of the sphere is
A5 kg m2B2.7 kg m2C3.5 kg m2D4.5 kg m2What is the moment of inertia of solid sphere of density ρ and radius R about its diameter ?
A105176R2ρB105176R2ρC176105R2ρD186105R2ρ
Moment of Inertia of system of particles : rod, ring, disc, sheet, sphere etc
View SolutionWhat is moment of inertia of a solid sphere about its diameter ?
View SolutionThe moment of inertia of a sphere about its diameter is I. Four such spheres are arranged as shown in figure. Find the moment of inertia of the system about the axis XX'. (radius of each sphere is 2R).
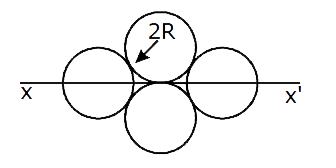
View SolutionThe moment of ineria (I) of a sphere of radius R and mass M is given by
View SolutionIf temperature increases, the moment of inertia of a solid sphere about its diameter
View Solution
