A projectile is thrown with a velocity of 18 m/s at an angle of 60∘ with horizontal. The interval between the moment when speed is 15 m/s is (g=10ms2)
(125)S
(45)S
12 S
(25)S
The correct Answer is:A
To solve the problem, we need to find the time interval during which the speed of the projectile is 15 m/s. The projectile is thrown with an initial velocity of 18 m/s at an angle of 60 degrees with the horizontal. We will break down the steps systematically.
Step 1: Determine the initial velocity components
The initial velocity u can be broken down into its horizontal and vertical components using trigonometric functions.
- Horizontal component ux=ucos(θ)
- Vertical component uy=usin(θ)
Given:
- u=18m/s
- θ=60∘
Calculating the components:
ux=18cos(60∘)=18×12=9m/s
uy=18sin(60∘)=18×√32=9√3m/s
Step 2: Write the expression for speed
The speed v of the projectile at any time t can be expressed as:
v=√v2x+v2y
Where vx is constant and equal to ux, and vy changes due to gravity.
Step 3: Set up the equation for speed
We need to find the time when the speed v=15m/s:
15=√u2x+v2y
Substituting ux=9m/s:
15=√92+v2y
Squaring both sides:
225=81+v2y
v2y=225−81=144
vy=±12m/s
Step 4: Determine the time for each case of vy
Using the vertical motion equation:
vy=uy−gt
For vy=12m/s:
12=9√3−10t1
Rearranging gives:
10t1=9√3−12
t1=9√3−1210
For vy=−12m/s:
−12=9√3−10t2
Rearranging gives:
10t2=9√3+12
t2=9√3+1210
Step 5: Calculate the time interval
The time interval Δt is given by:
Δt=t2−t1
Substituting the values:
Δt=(9√3+1210)−(9√3−1210)
Δt=(9√3+12)−(9√3−12)10
Δt=2410=2.4s
Final Answer
The time interval during which the speed of the projectile is 15 m/s is 2.4 seconds.
The initial velocity u can be broken down into its horizontal and vertical components using trigonometric functions.
- Vertical component uy=usin(θ)
- u=18m/s
- θ=60∘
ux=18cos(60∘)=18×12=9m/s
uy=18sin(60∘)=18×√32=9√3m/s
The speed v of the projectile at any time t can be expressed as:
v=√v2x+v2y
Where vx is constant and equal to ux, and vy changes due to gravity.
We need to find the time when the speed v=15m/s:
15=√u2x+v2y
Substituting ux=9m/s:
15=√92+v2y
Squaring both sides:
225=81+v2y
v2y=225−81=144
vy=±12m/s
Using the vertical motion equation:
vy=uy−gt
For vy=12m/s:
12=9√3−10t1
Rearranging gives:
10t1=9√3−12
t1=9√3−1210
−12=9√3−10t2
Rearranging gives:
10t2=9√3+12
t2=9√3+1210
The time interval Δt is given by:
Δt=t2−t1
Substituting the values:
Δt=(9√3+1210)−(9√3−1210)
Δt=(9√3+12)−(9√3−12)10
Δt=2410=2.4s
The time interval during which the speed of the projectile is 15 m/s is 2.4 seconds.
Topper's Solved these Questions
Similar Questions
A particle is projected with a velocity of 10√2m/s at an angle of 45∘ with the horizontal. Find the interval between the moments when speed is √125m/s(g=10m/s2)
View SolutionA particle is projected with a speed of "18m/s" at an angle of 60∘ with the horizontal.The average velocity of the particle between two instants when it is at the same height is
View Solution
Knowledge Check
A projectile is thrown with a velocity of 10ms−1 at an angle of 60∘ with horizontal. The interval between the moments when speed is √5gm/s is (Take, g=10ms−2)
A1sB3sC2sD4sA projectile is thrown with a velocity of 10√2m/s at an angle of 45∘ with horizontal. The interval between the moments when speed is √125m/s is (g=10m/s2)
A1.0sB1.5sC2.0sD0.5sA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
A10mB5mC2.5mD1m
A ball is thrown at a speed of 40 m/s at an angle of 600 with the horizontal. Find a. the maximum height reached and b. the range of te ball. Take g=10ms2.
View SolutionA particle is thrown with a velocity 10m/s and its horizontal range is 5m. Find angle/angles of the projection.
View SolutionA projectile is thrown with a velocity of 20 m/s, at an angle of 60∘ with the horizontal. After how much time the velocity vector will make an angle of 45∘ with the horizontal (in upward direction) is (take g=10m/s2)−
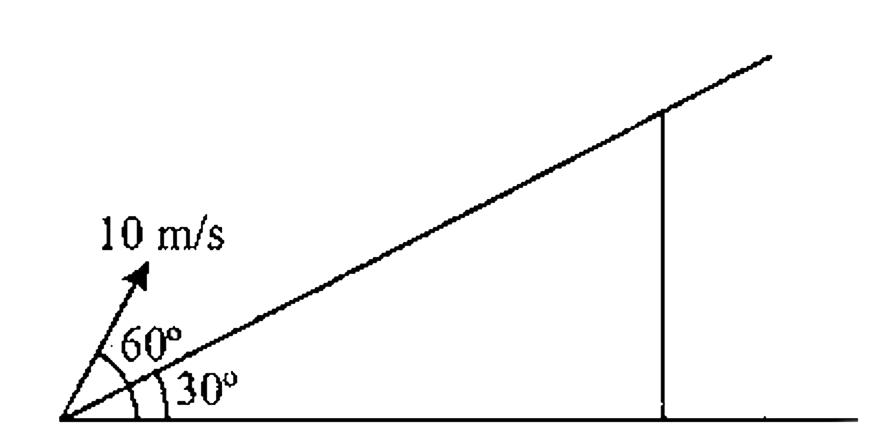
View SolutionA particle is thrown at time t=0 with a velocity of 10 m/s at an angle of 60∘ with the horizontal from a point on an incline plane, making an angle of 30∘ with the horizontal. The time when the velocity of the projectile becomes parallel to the incline is :
View SolutionWhat is the range of a projectile thrown with velocity 98m/s with angle 30∘ from horizontal ?
View Solution
