An ideal monatomic gas undergoes process PV1.25 = constant .Then
upon increase in pressure temperature decreases
upon increase in pressure heat is absorbed by gas
if heat is given to gas its internal energy increases
heat is absorbed by gas if volume increases
The correct Answer is:D
To solve the problem regarding the ideal monatomic gas undergoing the process PV1.25=constant, we will analyze the implications of this equation step by step.
1. Understanding the Process:
The equation PV1.25=constant suggests that the product of pressure P and volume V raised to the power of 1.25 remains constant throughout the process. This indicates a specific relationship between pressure and volume during the transformation.
Hint: Recognize that the relationship given can be used to derive other thermodynamic properties.
2. Using the Ideal Gas Law:
The ideal gas law states that PV=nRT, where n is the number of moles, R is the gas constant, and T is the temperature. Since PV=nRT, we can express pressure in terms of volume and temperature:
P=nRTV
Hint: Substitute P in the process equation to see how it relates to temperature.
3. Substituting into the Process Equation:
Substitute P from the ideal gas law into the process equation:
(nRTV)V1.25=constant
This simplifies to:
nRTV0.25=constant
Hint: This shows that as volume changes, the temperature must adjust to keep the product constant.
4. Analyzing Temperature Changes:
From the equation nRTV0.25=constant, we can see that if the volume V increases, the temperature T must also increase to maintain the constant. Conversely, if the volume decreases, the temperature must decrease.
Hint: Consider how changes in volume affect temperature and internal energy.
5. Applying the First Law of Thermodynamics:
The first law of thermodynamics states:
Q=ΔU+W
For an ideal gas, the change in internal energy ΔU is given by:
ΔU=nCVΔT
Since CV for a monatomic gas is 32R, if the temperature remains constant, ΔU=0.
Hint: Remember that for an isothermal process (constant temperature), the internal energy change is zero.
6. Work Done by the Gas:
The work done by the gas during expansion or compression can be defined as:
W=∫PdV
If the volume increases, the work done is positive; if the volume decreases, the work done is negative.
Hint: Relate the sign of work done to the changes in volume.
7. Conclusion on Heat Transfer:
Since ΔU=0, the first law simplifies to:
Q=W
If the volume increases (work done on the gas is negative), then heat must be absorbed by the gas. If the volume decreases (work done by the gas is negative), then heat is released.
Hint: This leads to the conclusion that the heat absorbed or released depends on the direction of the volume change.
Final Answer:
Based on the analysis, the correct conclusion is that if the volume increases, the gas absorbs heat; if the volume decreases, the gas releases heat.
Thus, the option stating that "Heat is absorbed by the gas if the volume is increased" is correct.
The equation PV1.25=constant suggests that the product of pressure P and volume V raised to the power of 1.25 remains constant throughout the process. This indicates a specific relationship between pressure and volume during the transformation.
The ideal gas law states that PV=nRT, where n is the number of moles, R is the gas constant, and T is the temperature. Since PV=nRT, we can express pressure in terms of volume and temperature:
P=nRTV
Substitute P from the ideal gas law into the process equation:
(nRTV)V1.25=constant
This simplifies to:
nRTV0.25=constant
From the equation nRTV0.25=constant, we can see that if the volume V increases, the temperature T must also increase to maintain the constant. Conversely, if the volume decreases, the temperature must decrease.
The first law of thermodynamics states:
Q=ΔU+W
For an ideal gas, the change in internal energy ΔU is given by:
ΔU=nCVΔT
Since CV for a monatomic gas is 32R, if the temperature remains constant, ΔU=0.
The work done by the gas during expansion or compression can be defined as:
W=∫PdV
If the volume increases, the work done is positive; if the volume decreases, the work done is negative.
Since ΔU=0, the first law simplifies to:
Q=W
If the volume increases (work done on the gas is negative), then heat must be absorbed by the gas. If the volume decreases (work done by the gas is negative), then heat is released.
Based on the analysis, the correct conclusion is that if the volume increases, the gas absorbs heat; if the volume decreases, the gas releases heat.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A certain amount of ideal monoatomic gas undergoes a process given by TV1/2= constant. The molar specific heat of the gas for the process will be given by
AR/2B−3R/2C3R/2D−R/2A diatomic gas undergoes a process represented by PV1.3= constant . Choose the incorrect statement
AThe gas expands by absorbing heat from the surroundingsBThe gas cools down during expansionCThe work done by surrounding during expansion of the gas is negativeDNone of theseA certain ideal gas undergoes a polytropic process PVn = constant such that the molar specific heat during the process is negative. If the ratio of the specific heats of the gas be γ, then the range of values of n will be
A0<n<γB1<n<γCn=γDn>γ
One mole of an ideal monatomic gas undergoes the process P=αT1/2, where α is constant . If molar heat capacity of the gas is βR1 when R = gas constant then find the value of β.
View SolutionAn ideal monatomic gas undergoes a cyclic process ABCA as shown in the figure. Graph of pressure versus volume of the cyclic process ABCA is
View SolutionOne mole of an ideal monatomic gas undergoes the process p=αT1/2 , whwre a is a constant. The work done by the gas if its temperature increases by 50K is 50Rx. Find the value of x.
View SolutionOne mole of an ideal monatomic gas undergoes the process p=αT1/2, where α is a constant.
(a) Find the work done by the gas if its temperature increases by 50K.
(b) Also, find the molar specific heat of the gas.
View SolutionAn ideal monatomic gas undergoes an adiabatic process , and its internal energy increases by 50 J which pair of choices below is correct for this process ?
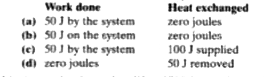
View Solution
