A bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be

mgL2AY
mgA2LY
mg2LAY
2LYmgA
The correct Answer is:A
Step by step video & image solution for A bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A bar of mass M and length L is hanging from point S as shown in figure. The Young's modulus of elasticity of the wire is Y and the area of cross-section of the wire is A.
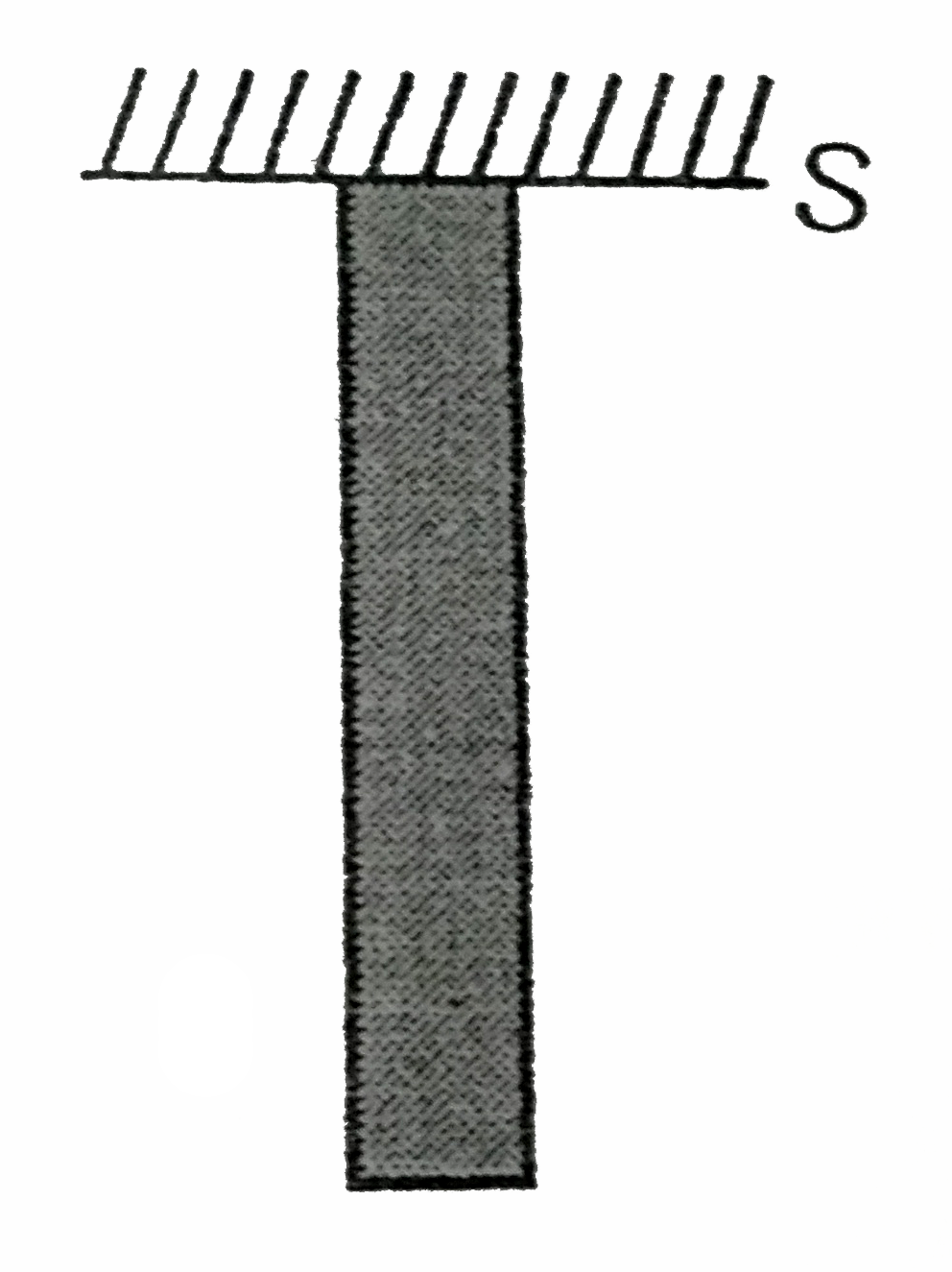
(i) Find the stress at x distance from bottom end.
(ii) Consider a samll section dx of the bar at a distance x from lowest point of bar. Find elongation (dL) in section dx.
(iii) Find total elongation in bar.
(iv) Find energy density at x distance from bottom and.
(v) Find total elastic potential energy stored in bar.
View SolutionA bar of mass m and length l is hanging from point A as shown in figure. Find the increase in its length due to its own weight. The young's modulus of elasicity of the wire is Y and area of cross-section of the wire is A.

View Solution
Knowledge Check
If Y is the Young's modulus of a wire of cross sectional area A, then the force required to increase its length by 0.1% will be
AAYBAY/100CAY/1000D1000 AYThe Young's modulus of a wire of length 2m and area of cross section 1mm2 is 2×1011N/m2. The work done in increasing its length by 2mm is
A0.1 JB0.2 JC0.02 JD0.4 JA 20 N stone is suspended from a wire and its length changes by 1%. If the Young's modulus of the material of wire is 2×1011N/m2, then the area of cross-section of the wire is 2×1011N/m2, then the area of cross-section of the wire will be
A10−3mm2B10−2mm2C10−1mm2D1mm2
A wire of length l and radius r has a weight W and the Young's modulus of elasticity Y. it is suspended vertically from a fixed point. Calculate the increase in length of wire porduced due to its own weight.
View SolutionA bar is subjected lu axial forces as shown. is the modulus of elasticity of the bar is E and A is it cross-section area. Its elongation will be
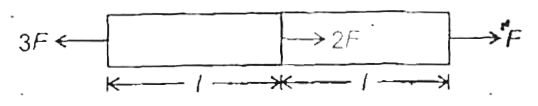
View SolutionTwo blocks of masses m1 and m2 are hanging from a pulley with the help of a ropw of Young's modulus Y as shown in the diagram. The length of the wire is L and area of cross section is A. When system is released from rest, the elongation in the length of wire is.

View SolutionA uniform wire of mass m, length L, area of cross-section A and Young's modulus Y hange from the ceiling. Its elongation under its own weight will be
View SolutionOn suspending a weight Mg, the length l of elastic wire and area of cross section A, its length becomes double the initial length. The instantaneous stress action on the wire is
View Solution